Chapter 6 Work , Energy And Power

CHAPTER NO.6 WORK,ENERGY AND POWER

6.1 INTRODUCTION

The terms ‘work’, ‘energy’ and ‘power’ are frequently used in everyday language. A farmer ploughing the field, a construction worker carrying bricks, a student studying for a competitive examination, an artist painting a beautiful landscape, all are said to be working. In physics, however,the word ‘Work’ covers a definite and precise meaning.Somebody who has the capacity to work for 14-16 hours a day is said to have a large stamina or energy. We admire a long distance runner for her stamina or energy. Energy is thus our capacity to do work. In Physics too, the term ‘energy’is related to work in this sense, but as said above the term

‘work’ itself is defined much more precisely. The word ‘power’ is used in everyday life with different shades of meaning. In karate or boxing we talk of ‘powerful’ punches. These are delivered at a great speed. This shade of meaning is close to the meaning of the word ‘power’ used in physics. We shall

find that there is at best a loose correlation between the physical definitions and the physiological pictures these terms generate in our minds. The aim of this chapter is to

develop an understanding of these three physical quantities.Before we proceed to this task, we need to develop a mathematical prerequisite, namely the scalar product of two vectors.

6.1.1 The Scalar Product

We have learnt about vectors and their use in Chapter 4.Physical quantities lke displacement, velocity, acceleration,

force etc. are vectors. We have also learnt how vectors are added or subtracted. We now need to know how vectors are multiplied. There are two ways of multiplying vectors which

we shall come across : one way known as the acalar product gives a acalar from two vectors and the other known as the

vector product produces a new vector from two vectors. We shall look at the vector product in Chapter 7. Here we take up the scalar product of two vectors. The scalar product or dot product of any two vectors A and B, denoted as A-B {read Adot B) is defined as A-B=A5cos 6 (6.14)

where @ is the angle between the two vectors as shown in Fig. 6.1(a). Since A, B and cos @ are scalars, the dot product of A and B is a scalar

quantity. Each vector, A and B, has a direction but their scalar product does not have a direction.

From Eq. (6.18), we have

AB =A(Bcos 6)

= B(Acos @}

Geometrically, B cos 6 is the projection of B onto Ain Fig.6.1 (b) and Acos 6 is the projection of A onto B in Fig. 6.1 (c). So, AB is the product of the magnitude of A and the component of B along A. Alternatively, it is the product of the Magnitude of B and the component of A along B.Equation (6.1a) shows that the acalar product

follows the commutative law :

AB=B-A Scalar product obeys the distributive law:

A (B+C)=AB+AC

Further, A: (AB)=4 (AB)

where 3, is a real number.The proofs of the above equations are left to you as an exercise.For unit vectors i, j,k we have feiej-jek-kel j.jej-k=k-i=0

Given two vectors

AzAi+A,j+ Ak

B=B,i+B,j+ Bk

their scalar product is AB=(A.i+ A,j+ A,k)-(Ba+ B,j+ Bk)= A,B, +A,B, + A,B, (6.1b)

From the definition of scalar product and (Eq. 6.1b) we have :

(1) A-A=A,A, +4,A, +4,A,

Or, A’ HAZE AP 4A? (6.1¢)

since A-A= 1A | |Al cos 0 =A”.

Gi) <A-B=0, ifAand B are perpendicular.

Example 6,1 Find the angle between force

F=(3i+4 ij -— 5k) unit and displacement

d = (5i+4j+3k) unit. Also find the

projection of F on d.Answer Fd =F .d.+ Fd, + Fd,=3 (5) + 4 (4) + (-5) (3)

=16 unit

Hence F-d@ = Fd cosé@ = 16 untt

NwFF =F oF + Fs Fe =9+16+4+25

= 50 unit and d-d =@= d+ +d

=25+16+9 = 50 unit

. 1616 og , COB 8 = Foofso 50° +

‘e=cos! 0.32 <

6.2 NOTIONS OF WORK AND KINETIC

ENERGY: THE WORE-ENERGY THEOREM

The following relation for rectilinear motion under constant acceleration a has been encountered in Chapter 3,

v-w=2as where u and v are the initial and final speeds and s the distance traversed. Multiplying both sides by m/2, we have

1 me - si = mas = Fs 6

gi —5 = = (6.2a)

where the last step follows from Newton's Second Law. We can generalise Eq. (6.1)to three dimensions by employing vectors

Y-w=2ad

Once again multiplying both sides by m/2 , we obtain

lo, 1,

3 - 3m =mad=F.d (6.2b)

The above equation provides a motivation for the definitions of work and kinetic energy. The left side of the equation is the difference in the quantity ‘half the mass times the square of the speed’ from its initial value to its final value. We

call each of these quantities the ‘kinetic energy’,denoted by K. The right side is a product of the displacement and the component of the force

along the displacement. This quantity is called ‘work’ and is denoted by W. Eq. (6.2b) is then

K,- K=W (6.3)

where K, and K, are respectively the inftial and final kinetic energies of the object. Work refers to the force and the displacement over which it acts. Work is done by a force on the body over

a certain displacement.

Equation {6.2} is also a special case of the work-energy (WE) theorem : The change in Kinetic energy of a particle is equal to the work done on it by the net force. We shall generalise the above derivation to a varying force in a later section.

Example 6.2 It is well known that a

raindrop falls under the influence of the downward gravitational force and the

opposing resistive force. The latter is

known to be proportional to the apeed of

the drop but is otherwise undetermined.

Consider a drop of mass 1.00 ¢g falling from a height 1.00 km. It hits the ground with a apeed of 50.0 ms“. (a) What is the work done by the gravitational force ? What is

the work done by the unknown reaistive

force?

Answer (a) The change in kinetic energy of the drop is

AK = =m v’-0

4 .

= 2* 10° X50x*50

=1,.25J

where we have assumed that the drop ts initially at rest.Assuming that g is a constant with a value 10.m/s*, the work done by the gravitational force

ia,W,=mgh

=10°x10x10°

=10.0J

(b) From the work-energy theorem

‘AK = W, +W,

where W, is the work done by the resistive force on the raindrop. Thus

W, =AK- W,

= 1.25-10

=-8.75J

is negative.

6.3 WORE

As seen earlier, work 1s related to force and the displacement over which it acts. Consider a constant force F acting on an object of masa m.The object undergoes a displacement d in the

positive x-direction as shown in Fig. 6.2.The work done by the force is defined to be the product of component of the force in the direction of the displacement and the magnitude of this displacement. Thus We (Fcoa jd = F.d (6.4)We see that if there is no displacement, there is no work done even if the force ts lange. Thus,when you push hard against a rigid brick wall,

the force you exert on the wall does no work. Yet your muscles are alternatively contracting and relaxing and internal energy is being used up and you do get tired. Thus, the meaning of work

in physics is different from its usage in everyday language.

No work is done if:the displacement is zero as seen in the example above. A weightlifter holding a 150 kg mass steadily on his shoulder for 30 s

does no work on the load during this time.{9 the force fs zero. A block moving ona smooth horizontal table is not acted upon by 4 hortzontal force (since there is no friction), but

may undergo a large displacement.

(ii) the force and diaplacement are mutually perpendicular. This is so since. for @= 5/2 rad {= 904, cos (4/2) = 0. For the block moving on a smooth horizontal table, the gravitational

force mg does no work since it acts at right angles to the displacement. Ifwe assume that the moon's orbita around the earth is perfectly circular then the earth’a gravitational force docs no work. The moon's instantaneous displacement is tangential while the earth's force is radially towards and

6 =nf/2.

Work can. be both positive and negative. If @ is between 0° and 90°, cos @ tn Eq. (6.4) is positive.If @ ia between 90° and 180°, cos ¢ is negative.In many examples the frictional force opposes

displacement and @ = 180°. Then the work dane by friction is negative (cos 180° = -1).

From Eq. (6.4) it ts clear that work and energy have the same dimensions, [ML?T“]. The SI unit of these ts joule (J), named after the famous British

physicist James Prescott Joule (181 1-1869}. Since work and energy are so widely used as physical concepts, alternative units abound and some of

these are Hated in Table 6.1.

Example 6.3 Acyclist comes to a skidding

stop in 10m. During this process, the force on the cycle due to the road is 200 N and is directly opposed to the motion. (a) How much work does the road do on the cycle ?

(b) How much work does the cycle do on

the road ?

Answer Work done on the cycle by the road is the work done by the stopping (irictional) force on the cycle due to the road.

(a) The stopping force and the displacement make an angle of 180° {« rad) with each other.Thus, work dane by the road,

W,= Facosé

= 200x 10x cosx

=-2000 J

It is this negative work that brings the cycle to a halt in accordance with WE theorem.

(b) From Newton's Third Law an equal and

opposite force acts on the road due to the cycle. Its magnitude is 200 N. However, the road undergoes no displacement. Thus,work done by cycle on the road is zero.The lesson of Example 6.3 is that though the force on a body Aexerted by the body B is always

equal and opposite to that on B by A (Newton's Third Law); the work done on A by B is not neceasartily equal and opposite to the work done on B by A.

6.4 KINETIC ENERGY

4s noted earlier, if an object of maas m has velocity v. tta kinetic energy K is

K 1 to,= ym v.V = yim (6.5)Kinetic energy is a scalar quantity. The kinetic

energy of an object is a measure of the work an

object can do by the virtue of its motion. This notion has been intuitively known for a long time.

The kinetic energy of a fast flowing stream has been used to grind corn. Sailing ships employ the kinetic energy of the wind. Table

6.2 lista the kinetic energies for various objects.

Example 6.4 Ina ballistics demonstration a police officer fires a bullet of mass 50.0 g with speed 200 m s” (see Table 6.2) on soft plywood of thickness 2.00 cm. The bullet emerges with only 10% of tts initial kinetic energy. What is the emergent speed of the bullet ?

Answer The initial kinetic energy of the bullet is mv?/2 = 1000 J. It has a final kinetic energy of 0.1x1000 = 100 J. Ifv, is the emergent speed of the bullet,

—mwe, =100J

2”

‘pe f2x1009

fy 0.05kg

=63.2ms!

The speed is reduced by approximately 68% (not 90%). <

6.5 WORE DONE BY A VARIABLE FORCE

Aconstant force is rare. It is the variable force,which is more commonly encountered. Fig. 6.2 is a plot of a varying force in one dimension.If the displacement Ax is small, we can take

the force FQ) as approximately constant and the work done is then AW =F () Ax

This is illustrated in Fig. 6.3(a). Adding successive rectangular areas in Fig. 6.3(a) we get the total work done as

Wey reac (6.8)

where the summation is from the initial position %, to the final position x,

If the displacements are allowed to approach zero, then the number of terms in the sum increases without limit, but the sum approaches a definite valie equal to the area under the curve

in Fig. 6.3(b). Then the work done is

. ay

Wwe a oda ee

y -

= | Fla) de 67

where ‘lim stands for the limit of the sum when Ax tends to zero. Thus, for a varying force the work done can be expressed as a definite integral of force over displacement (see also

Appendix 3.1).

Example 6,5A woman pushes a tainkon a raifway platform which has a rough

surface. She applies a force of 100 N overa distance of 10 m. Thereafter, she gets progressively tired and ber applied force reduces linearty with distance to 50 N. The total distance through which the trunk has been moved is 20 m. Plot the force applied by the woman and the frictional force, which is 50 N versus displacement. Calculate the work dons by the two forces over 20 m.a

The plot of the applied force is shown in Fig.6.4. At x =20m, F =50N (#0). We are given that the frictional force fis |f|=50 N. It opposes motion and acts in a direction opposite to F. It is therefore, shown on the negative side of the force axis.

The work done by the woman is W,-— area of the rectangle ABCD + area of

the trapeztum CEID

Wy = 100%10+5 (100+ 50)x10

= 1000 + 750

=1750J

The work done by the frictional force is

W,— area of the rectangle AGHI

W, =(-50) x 20 =-1000J

The area on the negative side of the force axis has a negative sign. <

6.6 THE WORKE-ENERGY THEOREM FOR A

VARIABLE FORCE We are now familar with the concepts of work and kinetic energy to prove the work-energy theorem for a variable force. We confine ourselves to one dimension. The time rate of change of kinetic energy is a (Ly?)

dé dt\2 .

- moe y

‘=F v (from Newton’s Second Law)

- pe

Thus dK=Filx Integrating from the initia] position (x,) to final

position ( x,), we have‘Ky xy

Jax= | Fax

K Ki

where, K, and K , are the initial and final kinetic energies corresponding to x, and x ,.

or K,—K,= J Fdx (6.8a)

From Eq. (6.7), it follows that

K,- K=W (6.8b)

Thus, the WE theorem is proved for a variable force.While the WE theorem is useful in a variety of problems, it does not, in general, incorporate the

complete dynamical information of Newton's second law. It is an integral form of Newton's second law. Newton's second law 1s a relation between acceleration and force at any instant of

time. Work-energy theorem involves an integral over an interval of time. In this sense, the temporal (time) information contained in the statement of Newton's second law is ‘integrated over’ and is notavaflable explicitly. Another observation is that Newton’s second law for two or three dimensions

is in vector form whereaa the work-energy theorem is in scalar form. In the scalar form,information with reapect to directions contained in Newton's second law is not present.

Example 6.6 A block of mass m = 1 kg,

moving on a hortontal surface with speed

u,= 2 ms* enters a rough patch ranging

from x=0.10m tox=2.01 m. The retarding

force F’ on the block in this range is inversely proportional to x over this range,-k

F= x for 0.1 <x< 2.01 m

= 0 for x<0.lmand x>2.01m

where k=0.5J. What is the final kinetic

energy and speed v, of the block aa it

crosses thia patch ?

Answer From Eq. (6.8a)

K, = K, +f ay

. 61%

= Ze kein (xfer!

_1.4

= gm -k1n(2.01/0.1)

= 2 -0.5 In (20.1)

=2-1.5 =0.5J

ure faKy/m =lms7

Here, note that In is a symbol for the natural logarithm to the base e and not the logarithm to the base 10 [In X=log_ X=2.303 log, , X]. <

6.7 THE CONCEPT OF POTENTIAL ENERGY

The word potential suggests possibility or capacity for action. The term potential energy brings to one's mind ‘stored’ energy. Aatretched bow-string possesses potential energy. When it

is released, the arrow flies off at a great speed.The earth's crust is not uniform, but has discontinuities and dislocations that are called fault lines. Theae fault lines in the earth's crust are like ‘compressed aprings’. They posseas a large amount of potential energy. An carthquake resnita when these fault lines readfust. Thus,potential energy Is the ‘stored energy by virtue

of the position or configuration of a body. The bedy left to itself releases this stored energy in the form of kinetic energy. Let us make our notion

of potential energy more concrete.

The gravitational force on a ball of mass m is mg. gray be treated as a constant near the earth surface. By ‘near’ we imply that the height h of

the ball above the carth’s aurface is very smafl compared to the carth’s radius K(k <<R} so that we can ignore the variation of g near the carth’s

surface*. In what follows we have taken the upward direction to be posttive. Let us raise the ball up toa height h. The work done by the external agency against the gravitational force is mah. This

work gets stored as potential energy.

Gravitational potential energy of an object, as a function of the height h, fs denoted by Vif) and it is the negative of work done by the gravitational force tn raising the object to that height.

V (h} = mgh

If k ia taken as a variable, it is easily scen that the gravitational force F equals the negative of the derivative of V{h) with reapect to &. Thus,

F= a V(Al = -mg dh

The negative sign indicates that the

gravitational force is downward. When released,the ball comes down with an increasing speed.Just before it hits the ground, its speed is given by the kinematic relation,w= 2gh

This equation can be written as

1 3m wemgh which shows that the gravitational potential energy of the object at height h, when the object

is released, mantiests itself as kinetic energy of the object on reaching the ground.

Physically, the notion of potential enemy is applicable only to the class of forces where work done against the force gets ‘stored up’ as energy.When externa) constraints are removed, it

mantipsts itselfas kinetic energy. Mathematically,(for simplicity, in one dimension) the potential energy V0 is defined if the force FQ can be

written as

F(x) dx

This implies that

Ne ve

J Foddx =-fdv=v,-v,

x vy,

The work done by a conservative force such as gravity depends on the initial and final positions only. In the previous chapter we have worked

on examplea dealing with inclined planea. If an object of mass mis released from reat, from the

top of a smooth (frictionless) inclined plane of height A, its speed at the bottom is /2gh irrespective of the angle of inclination.Thus, at the bottom of the inclined plane it acquires a kinetic energy, mgh. If the work done

or the kinetic energy did depend on other factors such as the velocity or the particular path taken by the object, the force would be called non-

conservative.

The dimensions of potential energy are

[ML?T-*] and the unit is joule (J), the same as kinetic energy or work. To reiterate, the change in potential energy, for a conservative force,

AV is equal to the negative of the work done by the force

AV =-— Fld Ax (6.9)

In the example of the falling ball considered in this section we saw how potential energy was converted to kinetic energy. This hints at an

fmportant principle of conservation in mechanics,which we now proceed to examine.

6.8 THE CONSERVATION OF MECHANICAL

ENERGY

For simplicity we demonstrate this important principle for one-dimensional motion. Suppose that a body undergoes displacement Ax under the action of a

conservative force F. Then from

the WE theorem we have,

AK= Fix Ax

If the force is conservative, the potential energy function V4 can be defined such that

—-AV = FI Ax

The above equations imply that

AK +AV=0

AIK +V)=0 (6.10)

which means that K + V, the sum of the kinetic and potential energies of the body is a constant.Over the whole path, x,to x, this means that

K,+ Vix) =K, + Vix) (6.11)

The quantity K +V(¥j, is called the total mechanical energy of the system. Individually the kinetic energy K and the potential energy V9 may vary from point to point, but the sum is a constant. The aptnesa of the term

‘conservative force’ is now clear.

Let us consider some of the definitions of a conservative force.A force FW is conservative if it can be derived from a scalar quantity V[X) by the relation

given by Eq. (6.9). The three-dimensional generalisation requires the use of a vector derivative, which is outside the scope of this book.

The work done by the conservative force

depends only on the end points. This can be seen from the relation,w= -K=V )-

which detent on th end eotnta,Athird definition states that the work done

by this force in a closed path is zero. This is once again apparent from Eq. (6.11) since % =.

Thus, the principle of conservation of total mechanical energy can be stated as

The total mechanical energy of a system is conserved if the forces, doing work on it, are conservative.

The above discussion can be made more

concrete by considering the example of the gravitational force once again and that of the spring force in the next section. Fig. 6.5 depicta a ball of mass m being dropped from a cliff of

height H.

The total mechanical energies E., E,,, and E,of the ball at the indicated heights zero (ground level), hand H, are

E,, =mgi (6.11 a)

E, = mgh+ Sm (6.11 b)

E, =(1/3 mu? (6.11 ¢

The constant force is a special case of a spatially dependent force FIN. Hence, the mechanical energy is conserved. Thus

BE,

or, mgH = Smet

ry =f2gH

a result that was obtained in section

3.7 fora

freely falling body.

Further,

B,=E,

which implies,vf = 2g(H-h) (6.11 d)

and is a familiar result from kinematics.

At the height H, the energy is purely potential.Itis partially converted to kinetic at height h and is fully kinetic at ground level. This illustrates

the conservation of mechanical energy.

Example 6.7 Abob ofmass mis suspended

by a light string of length L. It is tmparted a horizontal velocity v, at the lowest point A such that it completes a semi-circular trajectory in the vertical plane with the string beconing slack only an reaching the topmost point, C. This is shown in Fig. 6.6, Obtain an

expression for (i) v_; (i) the speeds at points Band C; (it) the ratio of the kinetic energies (K,/K, at B and C. Comment on the nature of the trajectory of the bob after it reaches the paint C.

Answer () There are two external forces on the bob : gravity and the tension (T) in the string. The latter does no work since the displacement of the bob is always normal to the string. The potential energy of the bob is thus

associated with the gravitational force only. The total mechanical energy E of the system is conserved. We take the potential energy of the system to be zero at the lowest point A. Thus,

at A:

. 1,

E= Bee (6.12)

Ty - nga [Newton’s Second Law]

where T, is the tension in the string at A. At the highest point C, the string slackens, as the tension in the string (T,} becomes zero.

Thus, at C

E= 5 mu? +2mgL (6.13)

mie

mg=— [Newton's Second Law] (6.14)

where v, is the speed at C. From Egg. (6.13) and

(6.14

E= 3 mal

Equating this to the energy at A

, Gg 2 mgL = > ve rm y= J5gL

(i It is clear from Eq. (6.14)

eo = io

At B, the energy is E= = mui +mgL

Equating this to the energy at A and employing the result from (), namely v5 =Sgl ,= mi +mgL= Sm

= om gL

tk Up = ¥3gL

(iif) The ratio of the kinetic energies at B and C is:

. Lg

Ky 2) 3

Ke sine? 1

At point C, the string becomes slack and the velocity of the bob is horizontal and to the left. If the connecting string is cut at this instant, the

bob will execute a projectile motion with horizontal projection akin to a rock kicked horizontally from the edge of a cliff. Otherwise the bob will continue on {ts circular path and

complete the revohition.

6.9 THE POTENTIAL ENERGY OF A SPRING

The spring force is an example ofa variable force which is conservative. Fig. 6.7 shows a block attached to a spring and resting on a smooth

horizontal surface. The other end of the spring is attached to a rigid wall. The spring is ight and may be treated as massless. In an ideal spring, the spring force F, is proportional to x where x is the displacement of the block from

the equilibrium position. The displacement could be either positive [Fig. 6.7(b)] or negative Tig. 6.7(c)]. This force law for the spring is called

Hooke's law and is mathematically stated asF,= — ke

The constant kis called the spring constant. Its unit is Nm. The spring is said to be stiff if kis large and soft if kis small.

Suppose that we pull the block outwards as in Fig. 6.7(b). If the extension is x_, the work done by the spring force is

W.= fy Fi.dx = -{ kx dx

: 2

=-im (6.15)

This expression may also be obtained by

considering the area of the triangle as in Fig. 6.7(a). Note that the work done by the external pulling force F is positive since it overcomes the spring force.

Fig. 6.7 Mustration of the spring force with a block attached to the free end of the spring.fa) The spring force F, is zero when the displacement x from the equilibria position fs zero. (b) For the stretched sprig x > 0 and F< © (c) For the compressed spring x<Oand F, > 0.(a) The plot of F, versus i The area of the shaded triangle represents the work done by the spring force. Due to the

opposing signs of F, and.x, this work done (s negative, W. = —kx* / 2.

The same is true when the spring is

compressed with a displacement x, (< 0). The spring force does work W, =- kx? /2 while the external force Fdoes work + kx? /2. Ifthe block is moved from an initial displacement x, to a

final displacement x,, the work done by the spring force W, is Wa-feed -k2 - ES 617

iL 2 2

Thus the work done by the spring force depends only on the end points. Specifically, if the block is pulled from x, and allowed to return to x, ;

xy 2 2

=0 6.18)

The work done by the spring force im a cyclic process is zero. We have explicitly demonstrated that the spring force ( is position dependent

only as first stated by Hooke, (F, = — jog; (ii)does work which only depends on the initial and final positions, e.g. Eq. (6.17). Thus, the spring force is a conservative force.

‘We define the potential energy VX) of the spring ‘to be zero when block and spring system is in the equilibrium position. For an extension (or

compression) x the above analysis suggests that vo) = 6.19)

You may easily verify that - dV/dx = —k«x, the spring force. If the block of mass min Fig. 6.7 is extended to x, and released from rest, then its total mechanical energy at any arbitrary point x,where xlies between —x, and +x,, will be given by 4K xtalte xan vp?

2 2 2

where we have invoked the conservation of mechanical energy. This suggests that the speed and the kinetic energy will be maximum at the equilibrium position, x = 0, Le.,

lo» less

3" vy, =5K x2

where uv, is the maximum speed.

or Cn=yt— Xin

Note that k/m has the dimensions of [T*] and our equation fs dimenstonally correct. The kinetic energy gets converted to potential energy

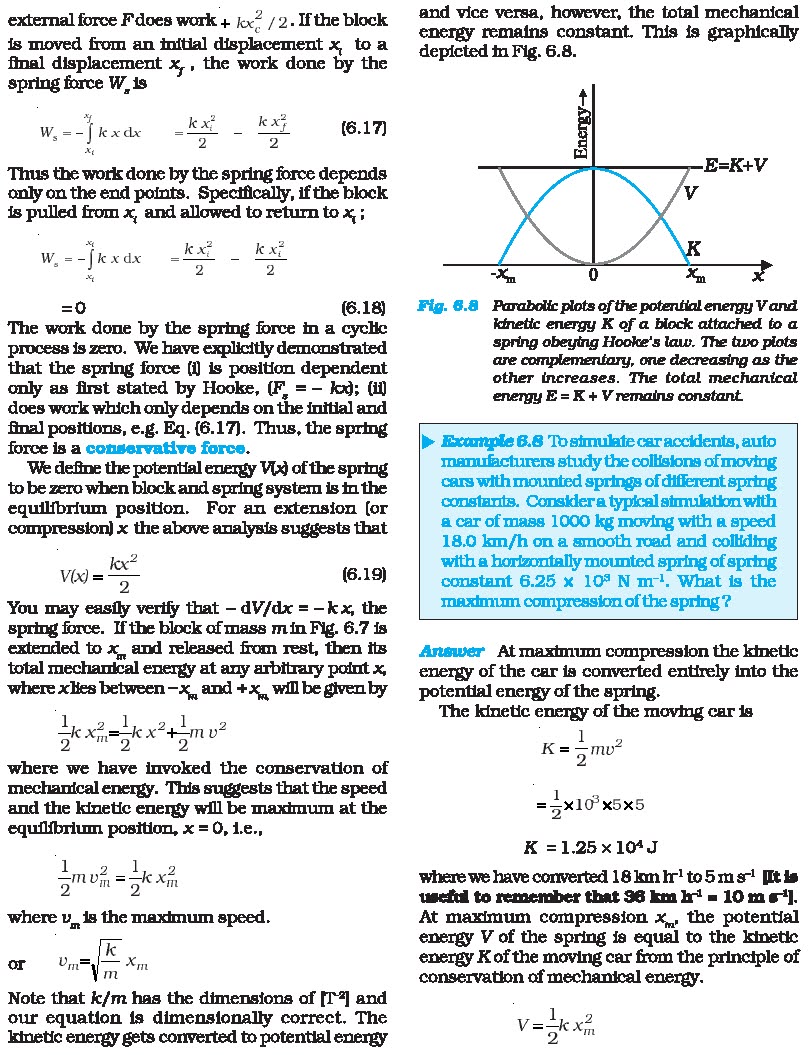
and vice versa, however, the total mechanical energy remains constant. This is graphically depicted in Fig. 6.8.

Example 6.8 To simulate car accidents, auto manufacturers study the collisions of moving cars with mounted springs of different spring constants. Consider a typical simulation with a car of mass 1000 kg moving with a speed 18.0 km/h on a smooth road and colliding with a horizontally mounted spring of spring

constant 6.25 x 10° N m'!. What is the

maximo compression of the spring?

Answer At maximum compression the kinetic energy of the car is converted entirely into the potential energy of the spring.The kinetic energy of the moving car is

K=Smv?

=5%10°x5x5

K =1.25x10J

where we have converted 161m Ir! toS ms [it is useful to remember that 36 km h’ = 10 m “J.At maximum compression x,, the potential energy V of the spring is equal to the kinetic energy K of the moving car from the principle of

conservation of mechanical energy.

ae Vash xin

=1.25x 10tJ

We obtain

x, = 2.00m

We note that we have idealised the situation.The spring is considered to be massless. The surface has been considered to possess negligible friction. We conclude this section by making a few remarks on conservative forces.

Information on time is absent from the above discussions. In the example considered above, we can calculate the compression, but not the time over which the compression occurs. Asolution of Newton’s Second Law for this system is required for temporal information.

(ii) Not all forces are conservative. Friction, for example, is a non-conservative force. The principle of conservation of energy will have

to be modified in this case. This is Mlustrated in Example 6.9.

(ii) The zero of the potential energy is arbitrary.It is set according to convenience. For the spring force we took V(X =0, at x=0, ie. the

unstretched spring had zero potential

energy. For the constant gravitational force mg, we took V=0 on the earth’s surface. In a later chapter we shall see that for the force due to the universal law of gravitation, the zero 1s beat defined at an infinite distance

from the gravitational source. However, once the zero of the potential energy is fixed ina given discussion, it must be consistently adhered to throughout the discussion. You cannot change horses in midstream !

Example 6.9 Consider Example 6.8 taking

the coefficient of friction, p, to be 0.5 and calculate the maximum compression of the spring.

Answer In presence of friction, both the spring force and the frictional force act so aa to oppose the compression of the spring as shown in Fig. 6.9.

We invoke the work-energy theorem, rather than the conservation of mechanical energy.The change in kinetic energy is

AK #K-K=0-5m wv

The work done by the net force is

Wa-s kext - un gx,

Equating we have

=m w= > K Xi + MN GX,

Now ymg =0.5 x 10°x 10=5 x 10°N (taking

g =10.0 ms”). After rearranging the above equation we obtain the following quadratic equation in the unknown x,,

‘k x? +Qum gXx,, —m’ =0

-umg+[pem?g? +mk vw |

Xm rs

where we take the positive square root since x,is positive. Putting in numerical values we obtain

*, =1.35m

which, as expected, is less than the result in Example 6.8.

If the two forces on the body consist of a conservative force F, and a non-conservative force F__ , the conservation of mechanical energy

formula will have to be modified. By the WE theorem

(F,+ F) Ae =AK

But F, Ax=- AV

Hence, AK + V) = F_Ax

AE =F Ax

where £ is the total mechanical energy. Over the path this assumes the form

Ek, =W,,

Where W,, is the total work done by the

non-conservative forces over the path. Note that unlike the conservative force, W_, depends on the particular path i to f <

6.10 VARIOUS FORMS OF ENERGY : THE LAW

OF CONSERVATION OF ENERGY

In the previous section we have discussed mechanical energy. We have seen that it can be classified into two distinct categories : one based

on motion, namely kinetic energy; the other on configuration (position), namely potential energy.Energy comes in many a forms which transform

into one another in ways which may not often be clear to us.

6.10.1 Heat

We have seen that the frictional force is not a conservative force. However, work is associated with the force of friction, Example 6.5. A block of

mass m sliding on a rough horizontal surface with speed v, comes to a halt over a distance x,The work done by the force of kinetic friction f over x, 1s —f x, By the work-energy theorem

mv,/2 =S Xo. If we confine our scope to

mechanics, we would say that the kinetic energy of the block is ‘lost’ due to the frictional force.On examination of the block and the table we would detect a slight increase in their temperatures. The work done by friction is not

‘ost’, but is transferred as heat energy. This raises the internal energy of the block and the table. In winter, in order to feel warm, we generate heat by vigorously rubbing our palms

together. We shall see later that the internal energy is associated with the ceaseless, often random, motion of molecules. A quantitative idea

of the transfer of heat energy is obtained by noting that 1 kg of water releases about 42000 J of energy when it cools by10 °C.6.10.2 Chemical Energy

One of the greatest technical achievements of humankind occurred when we discovered how to ignite and control fire. We learnt to rub two flint stones together (mechanical energy), got

them to heat up and to ignite a heap of dry leaves (chemical energy), which then provided sustained warmth. A matchstick ignites into a bright flame when struck against a specially Prepared chemical surface. The lighted matchstick, when applied to a firecracker,results in a spectacular display of sound and

light.

Chemical energy arises from the fact that the molecules participating in the chemical reaction have different binding energies. Astable chemical compound has less energy than the separated parts.Achemical reaction is basically a rearrangement ofatoms. Ifthe total energy of the reactants is more

than the products of the reaction, heat is released and the reaction is said to be an exothermic Teaction. Ifthe reverse is true, heat is absorbed and the reaction is endothermic. Coal consists of carbon and a kilogram of it when burnt releases about 3 x 10’ J ofenergy.

Chemical energy is associated with the forces that give rise to the stability of substances. These forces bind atoms into molecules, molecules into

polymeric chains, etc. The chemical energy arising from the combustion of coal, cooking gas.wood and petroleum is indispensable to our daily existence.

6.10.3 Electrical Energy

The flow of electrical current causes bulbs to glow, fans to rotate and bells to ring. There are laws governing the attraction and repulsion of charges and currents, which we shall learn

later. Energy ie associated with an electric current. An urban Indian household consumes about 200 J of energy per second on an average.

6.10.4 The Equivalence of Mass and Energy Till the end of the nineteenth century, physicists believed that in every physical and chemical process, the masa of an isolated system is conserved. Matter might change its phase, e.g.

glactal ice could melt into a gushing stream, but matter is neither created nor destroyed; Albert Einstein (1879-1955) however, showed that maas

and energy are equivalent and are related by the relation

E=meé (6.20)

where c, the speed of light in vacuum is

approximately 3 x10° m s'. Thus, a staggering amount of energy is associated with a mere kilogram of matter

E=1x (3 x10}? J = 9 x10!° J.

This is equivalent to the annual electrical output of a large (S000 MW) power generating station.

6.10.5 Nuclear Energy

The most destructive weapons made by man, the fission and fusion bombs are

mantfestations of the above equivalence of mass and energy [Eq.(6.20)]. On the other hand the explanation of the

life-nourishing energy output of the sun is also based on the above equation. In this case effectively four light hydrogen nuclei fuse to form a helium nucleus whose mass is less than the

suum of the masses of the reactants. This mass difference, called the mass defect Am is the source of energy (Amjc*. In fission, a heavy nucleus like wrantum %° U, is split by a neutron

into Highter nuclei. Once again the final mass is less than the inittal mass and the mase difference translates tnto energy, which can be tapped to provide electrical energy as tn nuclear power

plants (controlled nuclear fission) or can be employed in making nuclear weapons (uncontrolled nuclear fission). Strictly, the enenty AE released in a chemical reaction can also be related to the mass defect Am = AE/c?. However,

for a chemical reaction, this mass defect is much smaller than for a nuclear reaction. Table 6.3 lists the total energies for a variety of events and phensmena.

Kxampte 6.10 Examine Tablea 6.1-6.3

and expreaa (a) The energy required to

break one bond in DNA in eV; (b) The

kinetic energy of an air molecule (10~*! J} tn eV; (c) The dafly tntake ofa human adult in kilocaloriea.

Answer (a) Energy required to break one bond

of DNA is

-20

104 _ og o6 ev

1.6x10°°° J/ev

Note 0.1 eV = 100 meV (100 millielectron volt).(b) The kinetic energy of an air molecule is

-21

10 J _ 9. 0062 ev

1.6x10°°° J/eV

This is the same as 6.2 meV.

{c) The average human consumption in a day is

: 7

1d _ 9400 kcal

4.2x10° J/kcal

We point out a common misconception created by newspapers and magazines. They mention food values in calories and urge us to restrict diet intake to below 2400 calories. What they should be saying is kilocalories (kca]} and not

calories. A person consuming 2400 calories a day will soon starve to death! 1 food calorie is 1 kcal. <

6.10.6 The Principle of Conservation of

Energy ‘We have seen that the total mechanical energy of the system is conserved if the forces doing work

on it are conservative. If some of the forces involved are non-conservative, part of the mechanical energy may get transformed into other forms such as heat, light and sound.However, the total energy of an isolated system does not change, as long as one accounts for all forms of energy. Energy may be transformed from one form to another but the total energy of an isolated system remains constant. Energy can neither be created, nor destroyed.Since the universe as a whole may be viewed as an isolated system, the total energy of the

universe is constant. If one part of the universe loses energy, another part must gain an equal amount of energy.

The principle of conservation of energy cannot be proved. However, no violation of this principle has been observed. The concept of conservation and transformation of energy into various forms links together various branches of physics,chemistry and life sciences. It provides a unifying, enduring element in our scientific pursuits. From engineering point of view all electronic, communication and mechanical

devices rely on some forms of energy

transformation.

6.11 POWER

Often it is interesting to know not only the work done on an object, but also the rate at which this work is done. We say a person is physically fit if he not only climbs four floors of a building

but climbs them fast. Power is defined as the time rate at which work is done or energy is transferred.

The average power of a force is defined as the ratio of the work, W, to the total time t taken

, w

Pa. =

The instantaneous power is defined as the Itmiting value of the average power as time interval approaches Zero,

P di (6.21)

The work dW done by a farce F fora displacement dz is dW = F.dr. The instantaneous power can also he expressed as

P=F., di

aKy (6.22)

where v is the instantaneous velocity when the force is F.Power, like work and energy, is a scalar quantity. Its dimensions are [ML7T~]. In the SI,

its unit is called a watt (W). The watt is 1 Js".The unit of power is named after James Watt,one of the innovators of the steam engine in the eighteenth century.

There is another unit of power, namely the horse-power (hp)lhp=746W

This unit is still used to describe the output of automobiles, motorbikes, etc.

We encounter the unit watt when we buy

electrical goods such as bulbs, heaters and refrigerators. A 100 watt bulb which is on for 10 hours uses 1 kilowatt hour (kWh) of energy.

100 (watt) x 10 (hour)

= 1000 watt hour

=1 kilowatt hour (kWh)

=3.6x 10° J

Our electricity bills carry the energy

consumption in units of kWh. Note that kWh is a unit of energy and not of power.

Example 6.112 An elevator can carry a

maximum load of 1800 kg (elevator +

passengers) is moving up with a constant

speed of 2m 1. The frictional force opposing the motion is 4000 N. Determine the minimum power delivered by the motor to the elevator tn watts as well as tn horse power.

Answer The downward force on the elevator is F=mg+ F= (1800 x 10) + 4000 = 22000 N The motor must supply enough power to balance this force. Hence,

P=F,. v =22000 x 2 = 44000 W =59 hp <

6.12 COLLISIONS

In physics we study motion (change in position).At the same time, we try to discover physical quantities, which do not change in a physical process. The laws of momentum and energy conservation are typical examples. In this section we shall apply these laws to a commonly

encountered phenomena, namely collisions.Several games such as billiards, marbles or carrom involve collisions.We shall study the

collision of two masses in an idealised form.Consider two masses m, and m,. The particle m, is moving with speed vu,,, the subscript ‘f implying initial. We can cosider m, to be at rest.No loss of generality is involved in making such

a selection. In this situation the mass m,collides with the stationary mass m, and this is depicted in Fig. 6.10.

The masses m, and m, fly-off in different directions. We shall see that there are relationships, which connect the masses, the velocities and the angles.

6.12.1 Elastic and Inelastic Collisions

In all collisions the total lmear momentum is conserved; the initial momentum of the system is equal to the final momentum of the system.One can argue this as follows. When two objects

collide, the mutual impulsive forces acting over the collision time At cause a change in their respective momenta :

Ap, = F,, At

where F , is the force exerted on the first particle by the second particle. F’, is likewise the force exerted on the second particle by the first particle.

Now from Newton's third law, F,, = -F,,. This implies Ap, + Ap,= 0

The above conclusion is true even though the forces vary tn a complex fashion during the collision time At Since the third law fs true at every instant, the total impulse on the first object

is equal and opposite to that on the second.

On the other hand, the total kinetic energy of the system is not necessarily conserved. The impact and deformation during collision may generate heat and sound. Part of the inttial kinetic

energy is transformed into other forms of energy.Auseful way to visualise the deformation during collision is in terms of a ‘compressed spring’. If the ‘spring’ connecting the two masses regains its original shape without lose tn energy, then the initial kinetic energy is equal to the final kinetic energy but the kinetic energy during the collision time Af is not constant. Such a collision is called an elastic collision. On the other hand the deformation may not be relieved and the two bodies could move together after the collision. A collision in which the two particles move together after the collisian ts called a completely inelastic collision. The intermediate case where the deformation is partly relieved and some of the inttial kinetic energy is lost is more common and

is appropriately called an inelastic collision.

6.12.2 Collisions in One Dimension

Consider first a completely inelastic collision in one dimension. Then, in Fig. 6.10,6,=@,=0

m,v,,= (m,+mJu, (momentum conservation)

. _ vo

tO m+ m, (6.23)

The loss in kinetic energy on collision is , 1. 1 2 AK = Bln - aim +My vy

1 n yim ee fusing Eq. (6.23)]

“2° 2m +m," _

ut 2)y

= ihe F my + =I

An experiment on head-on collision

In performing an experiment on collision on a horizontal surface, we face three difficulties.One, there will be friction and bodice will not travel with untform velocities. Two, iftwo bodies of different sizes collide on a table, ft would be difficult to arrange them for a head-on collision unless their centres of mass are at the same height above the surface. Three, ft will be fairly

dificult to measure velocities of the two bodies just before and just after collision.

By performing this experiment in a vertical] direction, all the three difficultice vanish. Take two balls, one of which is heavier (hasketball/foothall/volleyball) and the other lighter (tennis ball/nubber ball/table tennis bal). First tale only the heavier ball and drop ft vertically from some height, say 1 m. Note to which it rises. This gives the velocities near the floor or ground,just before and fust after the bounce (by using v’ =2 gh). Hence you will get the coefficient of restitution.

Now take the big ball and a small ball and hold them in your hands one over the other, with the heavier ball below the lighter one, as shown here. Drop them together, taking care that they remain

together while falling, and see what happens. You will find that the

heavier ball rises less than when it was dropped alone, while the lighter one shoots up to about 3 m. With practice, you will be able to hold the ball properly so that the lighter ball rises vertically up and does not fly sideways. This is head-on collision.

You can try to find the best combination of balls which gives you ,the best effect. You can measure the masses on a standard balance.

We leave it to you to think how you can determine the imitial and final velocities of the balls. pe 8

1 MM, 2

2m +m,

which is a positive quantity as expected.Consider next an elastic collision. Using the above nomenclature with 0, = 6, = 0, the Momentum and kinetic energy conservation equations are

MV, = M0, +M,0,, (6.24)

mye = Myvi, + M405, (6.25)

From Eqs. (6.24) and (6.25) it follows that,

Mw, (Ws; — YJ = ML, (Ls; — UY ,)

or, ble, -o, )= UL _ vy

= (v,, - & ML, + v7)

Hence, “ bp HO, +4; (6.26)

Substituting this in Eq. (6.24), we obtain

. _ im =m.)

I m+m, (" (6.27)

. — 2mpv,

and U2; = m, +m, +m, (6.28)

Thus, the ‘unknowns’ {u,, v,) are obtained in terms of the ‘knowns’ {m,, m,, v,). Special cases of our analysis are interesting.Case I: If the two masses are equal vy=90 Bay = Ve

The first mass comes to rest and pushes off the second mass with its initial speed on collision. Case II; If one mass dominates, e.g. m, > > m,hyp Vy Uy O

The heavier mass is undisturbed while the lighter mass reverses its velocity.

Example 6.12 Slowing down of neutrons:

In a nuclear reactor a neutron of high

speed (typically 10’ m 57) must be slowed to 10° m s" so that it can have a high probability of interacting with isotope 3 and causing it to fission. Show that a neutron can lose most of tts kinetic energy in an elastic collision with a light nuclei Ike deutertum or carbon which has a mass of only a few times the neutron mass. The material making up the light nuclel, usually

heavy water (D,0) or graphite, is called a moderator.

Answer The initial kinetic energy of the neutron is , ls.K, = gen while its final kinetic energy from Eq. (6.27)

_l 2 lt m,—m, ) 3 Kips pmuvip =m & +My Mi

The fractional kinetic energy lost is

Kip (m-m,Si= K,, -( a while the fractional kinetic energy gained by the

moderating nuclei K,,/K,, is

4, =1-f (elastic collision)

7 4num,im, +m,)°

One can also verify this result by substituting from E4. (6.28).For deuterium m, = 2m, and we obtain

J, 21/9 while f, = 8/9. Almost 909% of the neutron’s energy is transferred to deutertum. For carbon f = 71.6% and £ = 28.4%. In practice,however, this number is smaller since head-on collisions are rare.

If the initial velocities and final velocities of both the bodies are along the same straight line,then it is called a one-dimensional collision, or

head-on collision. In the case of small spherical bodies, this is possible if the direction of travel of body 1 passes through the centre of body 2 which is at rest. In general, the collision is two-

dimensional, where the initial velocities and the final velocities lie in a plane.

6.12.3 Collisions in Two Dimensions

Fig. 6.10 also depicts the collision of a moving mass m, with the stationary mass m,. Linear momentum is conserved in such a collision.Since momentum is a vector this implies three equations for the three directions {x, y, 2}.

Consider the plane determined by the final velocity directions of m, and m,and chooae tt to be the x-y plane. The conservation of the z-component of the linear momentum implies that the entire collision is in the x-y plane. The

x and y-component equations are

MLV, = M,U,,cos 6, +m,v,,cos 8, (6.29)

0 =mv,, sin 6, — mv,,sin 6, (6.30)

One knows {m,, m,, v,} in most situations. There are thus four unknowns {v,,, v,,, @,and 0}, and only two equations. If @, =@, = 0, we regain

Eq. (6.24) for one dimensional collision.If, further the collision is elastic,too, log] 3 ate = Qtr + geter (6.31)

We obtain an additional equation. That atill leaves us one equation short. At least one of the four unknowns, aay @,, must be made known for the problem to be solvable. For example, @,can be determined by moving a detector in an

angular fashion from the x to the y axis. Given {m, m,, v,, 9} we can determine {u,,, 0,,, 0,from Ega. (6.29)-(6.31).

Example 6.13 Consider the collision

depicted tn Fig. 6.10 to be between two

billiard balls with equal massea m, = m,.The first ball is called the cue while the second ball is called the target. The billiard player wants to ‘sink’ the target ball in a corner pocket, which 1s at an angle 6, =37°. Asaume that the collision is elastic and that friction and rotational

motion are not fmportant. Obtain @,.

Answer From momentum conservation, since

the masses are equal Vy SV FV

or v= (vy + var) (Vip +Vaz]

‘= Vip + Wop + QV poy

= { vf +b, ,° +20, 0), 0s (0, + 37°) } (6.32)

Since the collision is elastic and m, =m, tt followa from conservation of kinetic energy that v=o? + ey/° (6.33)

Comparing Eqs. (6.32) and (6.33), we get

cos (0, + 37°) =0

or 6,+37°=90°

Thus, @, =55°

This proves the following result : when two equal masses undergo a glancing elastic collision with one of them at rest, after the collision, they will

move at right angles to each other. <

The matter simplifies greatly if we consider spherical masses with smooth surfaces, and assume that collision takes place only when the bodies touch each other. This is what happens

in the games of marbles, carrom and billiards.

In our everyday world, collisions take place only when two bodies touch each other. But consider a comet coming from far distances to the sun, or alpha particle coming towards a nucleus and

going away in some direction. Here we have to deal with forces involving action at a distance.Such an event is called scattering. The velocities

and directions in which the two particles go away depend on their initial velocities as well as the

type of interaction between them, their masses,shapes and sizes.

SUMMARY

1. The work-energy theorem states that the change in kinetic energy of a body is the work done by the net force on the body.K,-K,= Wh,

2. A force is conservative if () work done by it on an object is path tdependent and depends only on the end points {x, x], or (i) the work done by the force is zero for an arbitrary dosed path taken by the object mich that ft returns to its initial poattion.

3. For a conservative force in one dimension, we may define a potential energy function Vixd such that

F(x)=- dv (x)

or V,-V,= f F(x)dx

4. The princtple of conservation of mechanical energy states that the total mechanical energy of a body remains constant ff the only forces that act on the body are conservative.

5. The grazfational potential energy of a particle of mass m at a height x about the earth's aurface is

Vid =mgx

where the variation of g with height is ignored.

6. The elastic potential energy of a spring of force constant k and extension x is Vix)= tee 2

7. The scalar or dot product of two vectors A and BE is written as AB and is a scalar quantity given by: A-B = AB cos 6, where @ is the angle between Aand B. It can be positive, negative or zero depending upon the value of @ The scalar preduct of two vectors can be interpreted as the product of magnitude of one vector and component of the other vector along the first vector. For unit vectors :i-i-j-j-&-&=1 andi-j-j-&-%-i-0

Scalar products obey the commutative and the distributive laws.

POINTS TO PONDER

1. The phrase ‘calculate the work done’ is fncomplete. We should refer (or imply

Clearly by context) to the work done by a specific force or a group of forces on a given body over a certain displacement.

2. Work done ia a scalar quantity. It can be positive or negative unlike mass and kinetic energy which are poattive scalar quantities. The work done by the friction or viscous force on a moving body is negative.

3. For two bodies, the sum of the mutual forces exerted between them is zero from

Newton's Third Law,P+ F,, =0 But the sum of the work done by the two forces need not always cancel, te,Wo + W,,4# 0

However, it may sometimes be true.

4, The work done by a force can be calculated sometimes even if the exact nature of the force is not known. This ia clear from Example 6.2 where the WE theorem is used in such a situation.

5. The WE theorem ta not independent of Newton's Second Law. The WE theorem

may be viewed as a acalar form of the Second Law. The principle of conservation of mechanical energy may be viewed as a consequence of the WE theorem for conservative forces.

6. The WE theorem holda in all tnertial frames. It can also be extended to non-

inertial frames provided we include the pseudoforces in the calculation of the

net force acting on the body under consideration.

7. The potential energy of a body subjected to a conservative force is always undetermined upto a constant. For example, the point where the potential

energy is zero ia a matter of choice. For the gravitational potential energy mgh,the zero of the potential energy is chosen to be the ground. For the spring

potential energy fo4/2 , the zero of the potential energy is the equilibrium position of the oscillating masa.

8. Every force encountered in mechanics does not have an associated potential

energy. For example, work done by friction over a closed path ia not zero and no potential energy can be associated with friction.

9. During a collision : (a) the total lincar momentum is conserved at each instant of the colliaion ; {b) the kinetic energy conservation feven if the collision ia elastic}applies after the collision is over and docs not hold at every instant of the collisian.

In fact the two colliding objects are deformed and may be momentarily at rest

with reapect to each other.

EXERCISES

8.1 The sign of work done by a force on a body is important to understand. State carefully if the following quantities are positive or negative:

(a) work done by a man in lifting a bucket out of a well by means of a rope tied to the bucket.

(b) work done by gravitational force in the above case,

sliding down an inclined plane,

(d) work done by an applied force on

a body moving on a rough horizontal plane with uniform velocity,

e) work done by the resistive force of

afr on a vibrating pendulum in

bringing it to rest.

6.2 A body of mass 2 kg initially at rest Moves under the action of an applied horizontal force of 7 N on a table with coefficient of kinetic friction = 0.1,Compute the

fa) work done by the applied force in

108,(b) work done by friction in 10 a,

(ce) work done by the net force on the

body in 10 a,(@) change in kinetic energy of the body in 10 s, ‘and interpret your resulta.

6.3 Gtven in Fig. 6.11 are examples of some potential energy functions in one

dimension. The total energy of the

particle is indicated by a crosa on the

ordinate axis. In each case, specify the

regions, if any, im which the particle

cannot be found for the given energy.

Also, indicate the minimum total

energy the particle must have in each ¢

case. Think of simple physical contexts

for which these potential energy shapes

are relevant

6.4 The potential energy function for a

perticle executing linear simple

harmonic motion is given by Vid =

fo@/2, where k ia the force constant

of the oacillator. For k =0.6 N m-,

the graph of Vig versus x is ahown

in Fig. 6.12. Show that a particle of

total energy 1 J moving under thia

potential must ‘turn back’ when it

reaches x = + 2m.

6.5 Anawer the following :

(a) The casing of a rocket in flight

burns up due to friction. At whose expense is the heat energy required for burning obtained? The rocket or the

atmosphere?

(b) Comets move around the sun in highly elliptical orbite. The gravitational force on the comet due to the sun is not

norma] to the comet's velocity in general. Yet the work done by the gravitational force over every complete orbit of the comet ia zero. Why ?

(c) An artificial satellite orbiting the earth in very thin atmosphere loses ita energy gradually due to dissipation against atmospheric reaistance, however amall. Why then does ita apeed increase progresaively as it comes closer and closer to the earth ?

(a) In Fig. 6.13() the man walks 2 m carrying a mase of 15 kg on his handa. In Fig.6.1301), he walka the same distance pulling the rope behind him. The rope goea over a pulley, and a mase of 16 kg hangs at ita other end. In which case is the work done greater ?

6.6 Underline the correct alternative :

(a) When a conservative force does positive work on a body, the potential energy of the body increases / decreases /remains unaltered.

(b) Work done by a body against friction always results in a loss of tts kinetic/ potential energy.

(c) The rate of change of total momentum of a many-particle system is proportional to the external force/sum of the internal forces on the system.

(a) In an inelastic collision of two bodies, the quantities which do not change after the collision are the total kinetic energy/total Hnear momentum/total energy of the syatem of two bodies.

6.7 State if each of the following stataments is truc or false. Give reasone for your answer.

(a) In an elastic collision of two bodice, the momentum and energy of each body is conserved.

(b) Total energy of a system is always conserved, no matter what internal and external forces on the body are present.

(d) In an inelastic colliaion, the final kinetic energy is always lees than the initial kinetic energy of the system.

6.8 Answer carefully, with reasona :

(a) In an elastic collision of two billiard balls, is the total kinetic energy conserved during the short time of collision of the balls (Le. when they are in contact) ?

(b) Is the total linear momentum conaerved during the short time of an elastic colliaion of two balls ?

{c) What are the answers to (a) and (b) for an inelastic colliaion ?

{d} Ifthe potential energy of two billiard balls depends only on the separation distance between their centres, is the collision elastic or inelastic ? (Note, we are talking

here of potential energy corresponding to the force during collision, not gravitational potential energy).

6.9 A body ia initially at rest. It undergoes one-dimengional motion with constant acceleration. The power delivered to it at time fis proportional to 9 #9 Gt fi) 6 iv) #

6.10 A body is moving unidirectionally under the influence of a source of constant power.Ite displacement in time tis proportianal to {9 #72 wt Gi) 8 ivy) 2

6.11 A body constrained to move along the z-axia of a coordinate system is subject to a constant force F given by

F =-142j4+3kN

where i,j,k are unit vectors along the x-, y- and 2-axis of the aystem respectively.What is the work done by this force in moving the body a distance of 4 m along the z-axia ?

6.12 An electron and a proton are detected in a cosmic ray experiment, the first with kinetic energy 10 keV, and the second with 100 keV. Which is faster, the electron or the proton ? Obtain the ratio of their speeds. (electron mass = 9.11x10° kg, proton mass = 1.67x10" kg, 1 €V= 1.60 x10"? J).

6.13 Arain drop of radius 2 mm falls from a height of 500 m above the ground. It falls with decreasing acceleration (due to viscous resistance of the air} until at half its original height, it attains its maximum ftermina]) speed, and moves with uniform speed thereafter. What is the work done by the gravitational force on the drop im the first and second half of its journey ? What is the work done by the resisttve force in the entire journey if ite speed on reaching the ground ts 10 m a"! @

6.14 Amolecule in a gas container hits a horizontal wall with speed 200 m s" and angle 30° with the normal, and rebounds with the same speed. Is momentum conserved in the collision ? Is the colliaion elastic or inelastic 7

6.165 A pump on the ground floor of a building can pump up water to fill a tank of volume 30 m?in 15 min. If the tank is 40 m above the ground, and the efficlency of the pump is 30%,how much electric power is consumed by the pump ?

6.16 Two identical ball bearings in contact with each other and resting on a frictionless table are hit head-on by another ball bearing of the same mass moving mitially with a speed V. If the collision is elastic, which of the following (Fig. 6.14) 1s a possible result after collision ?

6.17 The bob A of a pendulum released from 30° to the vertical hits another bob B of the same mass at rest on a table as shown in Fig. 6.15. How high doce the bob A rise after the collision ? Neglect the size of the bobs and assume the collision to be clastic.

6.18 The bob of a pendulum is released from a horizontal position. If the length of the pendulum is 1.6 m,

what ia the speed with which the bob arrives at the lowermost point, given that it dissipated 5% af ita initial energy agatnat air reaistance ?

6.19 A trolley of mass 300 kg carrying a sandbag of 26 kg is moving uniformly with a speed of 27 km/h on a frictionlese track. After a while, sand starts leaking out of a hole on the floor of the trolley at the rate of

0.05 kg o'. What is the speed of the trolley after the entire sand bag is anpty 7?

6.20 A body of mass 0.6 kg travels in a straight line with velocity ved) where a=5010 7.What is the work done by the net force during ite displacement from x = 0 to x=2m?

6.31 The blades of a windmill sweep out a circle of area A (a) If the wind flows at a velocity v perpendicular to the circle, what is the mass of the air passing through it in time ¢? (b) What is the kinetic energy of the air ? (c}) Assume that the windmill converts 25% of the wind’s energy into electrical energy, and that A = 30 m?, v = 36

km/h and the density of air i 1.2 kg m*. What ia the electrical power produced ?

6.22 A person trying to lose weight (dieter} lifts a 10 kg mass, one thousand times, to a height of 0.6 m each time. Assume that the potential energy lost each time she lowers the mass ia disalpated. (a) How nruch work does she do against the gravitational

force ? (b) Fat supplies 3.8 x 107J of energy per kilogram which is converted to mechanical energy with a 20% efficiency rate. How much fat will the dieter use up?

6.23 A family uses 8 kW of power. (a) Direct solar energy is incident on the horizontal surface at an average rate of 200 W per square meter. If 20% of this energy can be converted to useful electrical energy, how large an area is needed to supply 8 kw?

{b) Compare thie area to that of the roof of a typical house.

Additional Exercises

6.24 A bullet of mass 0.012 kg and horizontal speed 70 m s“ strikes a block of wood of mags 0.4 kg and instantly comes to rest with respect to the block. The block is suspended from the ceiling by means of thin wires. Calculate the height to which the block rises. Also, eatimate the amount of heat produced in the block.

6.25 Two inclined frictionless tracks, one gradual and the other steep meet at A from where two stones are allowed to slide down from rest, one on each track (Fig. 6.16).Will the stones reach the bottom at the same time? Will they reach there with the same speed? Explain. Given 6, = 30°, 8, = 60°, and h= 10 m, what are the speeds and times taken by the two stones 7

6.26 A 1 kg block aituated on a rough incline is connected to a spring of spring constant 100 N m' as shown in Fig. 6.17, The block is released from rest with the spring in the unstretched position. The block moves 10 cm down the incline before coming to rest.Find the coefficient of friction between the block and the incline. Assume that the spring haa a negligible mass and the pulley is frictionless.

6.27 A bolt of masa 0.3 kg falls from the ceiling of an elevator moving down with an untform speed of 7m s". It hits the floor of the elevator (length of the elevator = 3 m) and does not rebound. What is the heat produced by the tmpact ? Would your answer be different

if the elevator were stationary ?

6.28 A trolley of mass 200 kg moves with a uniform speed of 36 km/h on a frictionless track.A child of masa 20 kg runs on the trolley from one end to the other (10 m away) with a speed of 4 m s° relative to the trolley in a direction opposite to the ita motion, and jumpe out of the trolley. What is the final speed of the trolley ? How much haa the

trolley moved from the time the child begins to run ?

6.29 Which of the following potential energy curves in Fig. 6.18 cannot posalbly describe the elastic collision of two billiard balls ? Here r is the distance between centres of the balls.

6,30 Conalder the decay of a free neutron at rest: n ~pt+e Show that the two-body decay of this type must necessarily give an electron of fixed

energy and, therefore, cannot account for the observed continuous energy distribution in the #-decay of a neutron or a nucleus (Fig. 6.19).

[Note: The simple result of this exercise was one among the several arguments advanced by W.Pauli to predict the existence of a third particle in the decay products of S-decay. This partide is known as neutrino. We now know that it fa a particle of intrinaic spin % (like e—, p or nj, but ia neutral, and either massless or having an extremely small mass (compared to the mass of electron) and which interacts very weakly with matter. The correct decay process of neutron is: n> pte +v|

APPENDIX 6.1 : POWER CONSUMPTION IN WALKING

The table below lista the approximate power expended by an adult human of maas 60 kg.Table 6.4 Approximate power consumption Mechanical work must net be confused with the everyday usage

of the tearm work. A woman standing with a very heavy load on her head may get very tred. But no mechanical work is involved.That is not to say that mechanical work cannot be estimated in

ordinary human activity.Consider a person walking with constant speed v,. The mechanical work he does may be estimated simply with the help of the work-energy theorem. Assume :

(a) The major work done in walking is due te the acceleration and deceleration of the legs with each stride(See Fig. 6.20).

(b) Neglect air resistance.

(d) Neglect the swinging of hands etc. as ie comman in walking.

As We can see in Fig. 6.20, in each atride the leg is brought from rest to a speed, approximately equal to the

apeed of walking, and then brought to rest again.Fig. 6.20 An tlustration of a single stride in walking. While the first leg ts maximally off the round, the second leg (s on the ground and vice-versa —The work done by one leg in cach stride is 17; vg by the work-energy theorem. Here m, is the masa of the leg.

Note 7, vg /2 energy is expended by one set of leg muscles to bring the foot from rest to speed v, while an

additional 1, vp /2 is expended by a complementary ect of leg muscles to bring the foot to reat from speed v,.

Hence work done by both legs in one etride is (study Fig. 6.20 carefully)

W,=2y VS (6.34)Assuming m,= 10 kg and slow running of a nine-minute mile which tranglates to 3 ms" mm SI units, we obtain 'W, =180 J /stride If we take a atride to be 2 m long, the peraon covers 1.5 atrides per second at his speed of 3m 5’. Thus the power expended

P=1s0—2_x1 5 Ste stride second

= 270W

We must bear in mind that this is a lower catimate alnce several avenues of power loss (c.g. swinging of hands,

air realetance etc.) have been ignored. The interesting point is that we did not worry about the forces involved.The forces, mainly friction and those exerted on the leg by the muscles of the rest of the body, are hard to estimate. Static friction does ne work and we bypassed the imposaible task of estimating the work done by the

muscles by taking recourse to the work-energy theorem. We can also sce the acivantage of a wheel. The wheel

Permits smooth locomotion without the continual starting and stopping in mammalian locomotion.