Chapter 7 Systems Of Particles And Rotational Motion

CHAPTER NO.7 SYSTEMS OF PARTICLES AND ROTATIONAL MOTION

 

7.1 INTRODUCTION

In the earlier chaptera we primarily considered the motion ofa single particle. (A particle is represented as a point mass.It has practically no size.) We applied the resulta of our

study even to the motion of bodies of finite size, assuming that motion of such bodies can be described in terms of the motion of a particle.

 

Any real body which we encounter in daily life has a finite size. In dealing with the motion of extended bodies

(bodies of finite size) often the idealised model of a particle is

fnadequate. In thia chapter we shall try to go beyond this tnadequacy. We shall attempt to bufld an understanding of

the motton of extended bodies. An extended body, in the firat place, ia a system of particles. We shall begin with the consideration of motion of the syatem as a whole. The centre of mass of a system of particles will be a key concept here.We shall discuss the motion of the centre of masa of a system

of particles and usefulness of this concept in understanding the motion of extended bodies.

 

A large class of problema with extended bodies can be solved by considering them to be rigid bodies. Ideally a rigid bady is a body with a perfectly definite and

unchanging shape. The distances between all pairs of particles of such a body do not change. It is evident from

thia definition of a rigid body that no real body ts truly rigid,since real bodies deform under the influence of forces. But in many situations the deformations are negligible. Ina number

of situations involving bodiea such as wheels, tops, ateel ‘beams, molecules and planets on the other hand, we can ignore that they warp, bend or vibrate and treat them as rigid.7.1.1 What kind of motion can a rigid body have?

Let us try to explore this question by taking some examples of the motion of rigid bodies. Let us begin with a rectangular block sliding down an inclined plane without any sidewise

movement. The block is a rigid body. Its motion down the plane is such that all the particles of the body are moving together, 1.c. they have the

same velocity at any instant of time. The rigid body here is in pure translational motion (Fig. 7.1).

 

In pure translational motion at any instant of time all particles of the body have the same velocity.

 Consider now the rolling motion of a solid metallic or wooden cylinder down the same inclined plane (Fig. 7.2). The rigid body in this problem, namely the cylinder, shifts from the top to the bottom of the inclined plane, and thus,

has translational motion. But as Fig, 7.2 shows,all its particles are not moving with the same velocity at any instant. The body therefore, is

not in pure translation. Its motion is translation plus ‘something elze.’

 

In order to understand what this ‘something else’ is, let us take a rigid body ao constrained that it cannot have translational motion. The most common way to constrain a rigid body so that ft doca not have translational motion is to

fix it along a straight line. The only possible mnotion of such a rigid body is rotation. The line along which the body ts fhoed is termed as its axis of rotation. If you look around, you

will come across many examples of rotation about an axis, a cedling fan, a potter's wheel, a giant wheel in a fair, a merry-go-round and so on (Fig 7.3(a) and (b)).

 

Let us try to understand what rotation is,what characterises rotation. You may notice that in rotation of a rigid body about a fixed axis, every particle of the body moves in a circle, which lies in a plane perpendicnolar to the axis and has its centre on the axis. Fig.

7.4 shows the rotational motion of a rigid body about a fixed axis (the z-axis of the frame of reference}. Let P, be a particle of the rigid body,

arbitrarily chosen and at a distance r, from fixed axia. The particle P, describes a circle of radius

7, with its centre C, on the fixed axis. The circle lies in a plane perpendicular to the axis. The figure also shows another particle P, of the rigid

body, P, is at a distance r, from the fixed axis.The particle P, moves in a circle of radius rand with centre C, on the axis. This circle, too, Hes

in a plane perpendicular to the axis. Note that the circles described by P, and P, may He in different planes; both these planes, however,are perpendicular to the fixed axis. For any particle on the axis like P,, r = 0. Any such

particle remains stationary while the body rotates. This is expected since the axis is fixed.

 

In some examplea of rotation, however, the axis may not be fixed. A prominent example of this kind of rotation is a top spinning in place [Fig. 7.5{a)]. (We assume that the top does not.slip from place to place and so doea not have

translational motion.) We know from experience that the axia of such a spinning top moves around the vertical through its point of contact.with the ground, sweeping out a cone as shown

in Fig. 7.5{a). (This movement of the axis of the top around the vertical ia termed precession.)Note, the point of contact of the top with ground is fixed. The axis of rotation of the top

at any instant passes through the point of contact. Another simple exampk of this kind of rotation is the oscillating table fan or a pedestal fan. You may have observed that the axis of

rotation of such a fan has an oscillating (sidewise) movement in a horizontal plane about the vertical through the point at which the axis

is pivoted (point O in Fig. 7.5(b)).

 

While the fan rotates and its axis moves

sidewise, this point is fixed. Thus, in more general cases of rotation, such as the rotation of a top or a pedestal fan, one point and not one line, of the rigid body is fixed. In this case the axis is not fixed, though it always passes

through the fixed point. In our study, however,‘we mostly deal with the simpler and special case of rotation in which one line (Le. the axis) is Fg 7.6 (a} and 7.6 (b) Mustrate different motions of the same body. Note P ts an arbitrary potul of the bedy; O ts the centre of mass of the body, which is defined in the next section. Suffice to say here that the trajectories of O are the translational trajectories Tr, and Tr, of the body. The posttions O and P at

twee different instants of time are shown by O,, O,,and O,, and P,, P, and P,, respectively, in both gs. 7.6 fa} and (b) . As seen from Fig. 7.6ia), at any tnstant the velocities of any particles ice O and P of the body are the same in pure translation. Notice, in

tits case the orientation of OP, Le. the angle OP makes with a fixed direction, say the horizontal, remains the same, Le. a, = a, = a, Fig. 7.6 (b} tlusirates a case of combination of translation and rotation. In this case, at any instants the velocities of O and P differ. Also, a,, a, and a, may all be different.

fixed. Thus, for us rotation will be about a fixed axis only unless stated otherwise.

 

The rolling motion of a cylinder down an

inclined plane is a combination of rotation about a fixed axis and translation. Thus, the ‘something else’ in the case of rolling motion

which we referred to earlier is rotational motion.You will find Fig. 7.6(a) and (b) instructive from

this point of view. Both these figures show motion of the same body along identical translational trajectory. In one case, Fig. 7.6(a),the motion is a pure translation; in the other

case [Fig. 7.6(b)] it is a combination of translation and rotation. (You may try to reproduce the two types of motion shown using a rigid object like a heavy book.)

 

We now recapitulate the most important

observations of the present section: The motion of a rigid body which is not pivoted or fixed in some way is either a pure translation or a combination of translation and rotation. The

motion of a rigid body which is pivoted or fired in some way is rotation. The rotation may be about an axis that is fixed (e.g. a ceiling fan) or moving (e.g. an oscillating table fan). We

shall, in the present chapter, consider rotational motion about a fixed axis only.

 

7.2 CENTRE OF MASS

‘We shall first see what the centre of mass of a system of particles is and then discuss its significance. For simplicity we shall start with

a two particle system. We shall take the line joiing the two particles to be the x- axis.

 

Let the distances of the two particles be x,and x, respectively from some origin 0. Let m,and m, be respectively the masses of the two particles. The centre of mass of the system is

that point C which fs at a distance X from O,

where X is given by

MX, FIMX;

X= 11 es

m, +m, (7.1)

 

In Eq. (7.1), Xcan be regarded as the maas-weighted mean of x, and x,. If the two particles have the same maaa m, = m, = m then

xe MY, FM, _ +X,

2m 2

 

Thus, for two particles of equal mass the centre of mass lies exactly midway between them.

 

If we have n particles of masses m,, m,,

...m, respectively, along a straight line taken as the x- axis, then by definition the position of the centre of the maas of the system of particles

is given by

xe FMA te AMX yx,

M, +My +....4M, ym.

(7.2)

 

where X,, X,,...x, are the distances of the particles from the origin; X is alao measured from the same origin. The symbol > (the Greek letter sigma) denotes summation, in this case

over n particles. The sum

yn =M

is the total mass of the system.

 

Suppose that we have three particles, not lying in a straight line. We may define x and y-axes in the plane in which the particles lie and represent the positions of the three particles by

coordinates O¢,.y,), (x,y) and bc,.¥,) respectively.Let the masses of the three particles be m,, ™ and m, respectively. The centre of mass C o the system of the three particles is defined and located by the coordinates (X. Y) given by

, MX, HAMAX, FMX,

X= yl ere Je3

Mm, FM, +M; (7.3a)

, MY, FOU, FOLLY,

Y = 1SA1 2efs JSf3

Mm +My, #M; (7.30)

For the particles of equal mass m= m, =m,

= My.

Xe MX, Xo FXy) OX AX + Xy

- 3m - 3

7 3m - 3

 

Thus, for three particles of equal mass, the centre of mass coincides with the centroid of the triangle formed by the particles.

 

Results of Eqs. (7.3a} and (7.3b) are

generalized easily to a system of n particles, not necessarily lying in a plane, but distributed in pace. The centre of mass of such a system is

at (X, Y, Z), where

, Ymrx,

Xa

M (74a)

ye ee

M (7.4b)

gee

and M (7.4c)

 

Here M = yn, is the total mass of the

system. The index iruns from | to n; m, is the mass of the @ particle and the position of the & particle is given by (x, y,, Z).

 

Eqs. (7.4a), (7.4b) and (7.4c) can be

combined into one equation using the notation of position vectors. Let r, be the position vector of the ¢ particle and R be the position vector of

the centre of mass:

r=x,i+y, j+z,k

and R=Xi+y j+Zk

Then R= 2% (7.40)

M

 

The sum on the right hand side is a vector sum.

 

Note the economy of expressions we achieve by use of vectors. If the origin of the frame of reference (the coordinate system) is chosen to

be the centre of mass then Ym, = 0 for the given system of particles.

 

A rigid body, such as a metre stick or a

flywheel, is a system of closely packed particles;Eqs. (7.4a), (7.4b), (7.4c) and (7.4d) are therefore, applicable to a rigid body. The number of particles (atoms or molecules) in such a body

is so large that it is impossible to carry out the summations over individual particles in these equations. Since the spacing of the particles is small, we can treat the body as a continuous

distribution of mass. We subdivide the body into nsmall elements of mass; Am,, Am... Am,; the f element Amis taken to be located about the point (x, ¥, Z). The coordinates of the centre of

mass are then approxhnately given by

7 Yam x, Ylanu, Yam, )z,

xX =" yea Zao

yAm, yam, yam,

 

As we make n bigger and bigger and each

Am, smaller and smaller, these  expressions become exact. In that case, we denote the stums over i by integrals. Thus,yam, > Jam =M,

Yam, > fxam.

Tiampy, 3 Juam,

and Tam, )Z, 9 Jz dm

 

Here M is the tota] mass of the body. The coordinates of the centre of mass now are X= a xdm Y= = Jydm and z-—f zdm (7.5a)

 

The vector expression equivalent to these three scalar expressions is

1

R=>,Jrdm (7.5b)

 

If we choose, the centre of mass as the origin of our coordinate system,

R=0

Le., jrdm =0

or fxdm =Jydm= [zdm=o (7.8)

 

Often we have to calculate the centre of mass of homogeneous bodies of regular shapes like rings, discs, spheres, rods etc. (By a homogeneous body we mean a body with uniformly distributed mass.) By using symmetry consideration, we can easily show that the centres of mass of these bodies lie at their geometric centres.

dm din

x-axis

x x

Fig. 7.8 Determining the CM of a thin rod.

 

Let us consider a thin rod, whose width and breath (in case the cross section of the rod is rectangular) or radius (in case the cross section of the rod is cylindrical) is much smaller than

its length. Taking the origin to be at the geometric centre of the rod and x-axis to be along the length of the rod, we can say that on account of reflection symmetry, for every element dm of the rod at x, there is an element of the same mass din located at —x (Fig. 7.8).

 

The net contribution of every such pair to the integral and hence the integral | xm itself is zero. From Eq. (7.6), the point for which the integral itself is zero, is the centre of mass.Thus, the centre of mass of a homogenous thin

rod coincides with its geometric centre. This can.be understood on the basis of reflection symmetry.

 

The same symmetry argument will apply to

homogeneous rings, discs, spheres, or even thick rods of circular or rectangular cross section. For all such bodies you will realise that for every element dm at a point (x y, 2) one can

always take an element of the same mass at the point (=x, -y, -2). (In other words, the origin is a point of reflection symmetry for these

bodies.) As a result, the integrals in Eq. {7.5 a)all are zero. This means that for all the above bodies, their centre of mass coincides with their geometric centre.

 

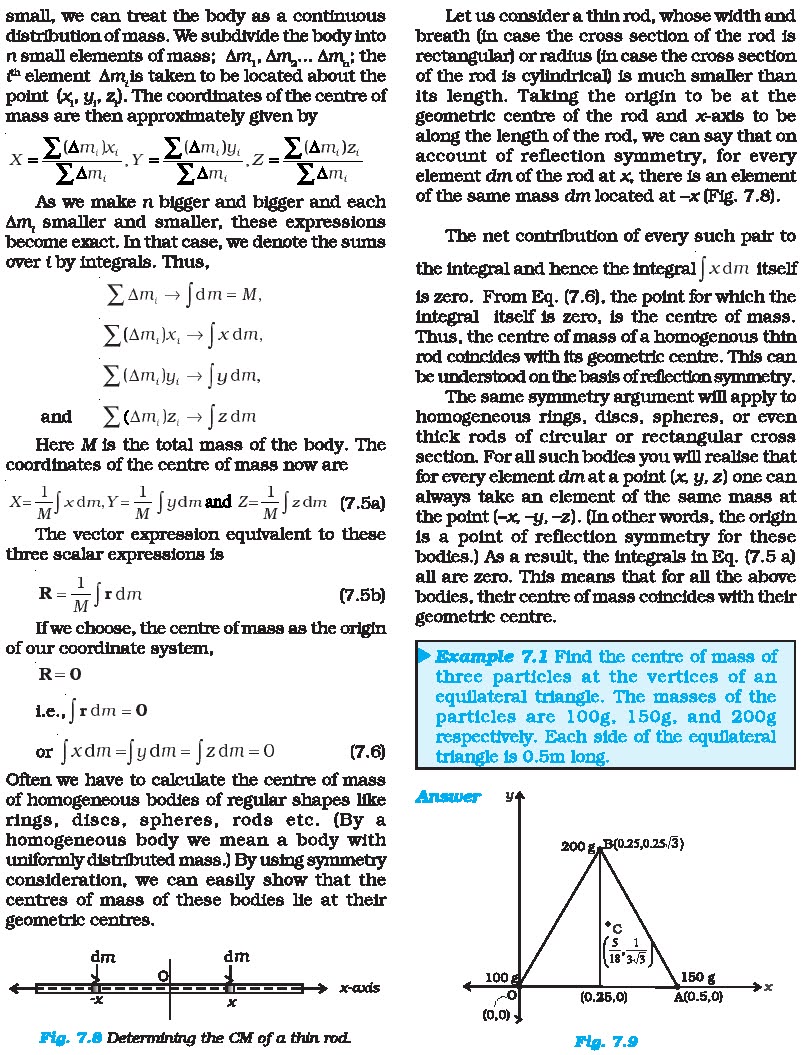
Example 7.1 Find the centre of mass of

three particles at the vertices of an

equilateral triangle. The masses of the

particles are 100g. 150g. and 200g

respectively. Each side of the equilateral triangle is 0.5m long.

 

With the x-and y-axes chosen as shown in Fig.7.9, the coordinates of points O, Aand B forming the equilateral triangle are respectively (0,0),(0.5,0), (0.25,0.25./3). Let the masses 100 g,

150g and 200g be located at O, A and B be respectively. Then,

x _ YX, FM, Mg Xy

“my, +m, +m;

[100 (0) + 150(0.5) + 200(0.25)] gm

~ (100 +150 + 200) g

_75+50, 1255

=—— m= ——m=—m

450 450 18

y [100(0) + 150(0) + 200(0.25¥3)} gm

~ 450 g

_ 50V3 3), = a

450 9 3¥3

 

The centre of mass C is shown in the figure.Note that ft is not the geometric centre of the triangle OAB. Why? <

 

Example 7.2 Find the centre of mass ofa

triangular lamina.Arnssaver The lamina (ALMN) may be subdivided into narrow atrips each parallel to the base (MN)

as shown in Fig. 7.10

 

By symmetry each strip has ita centre of

maaa at its midpoint. If we join the midpoint of all the strips we get the median LP. The centre of maas of the triangle as a whole therefore,

has to lie on the median LP. Similarly, we can argue that it lies on the median MQ and NR.This means the centre of maas lies on the point of concurrence of the medians, i.e. on the centroid G of the triangle. <

 

Example 7.3 Find the centre of mass ofa

uniform L-shaped lamina (a thin flat plate}with dimensions as shown. The mass of the lamina is 3 kg.

Answer Choosing the X and Yaxes as shown in Fig. 7.11 we have the coordinates of the vertices of the L-shaped lamina as given in the figure. We can think of the

L-shape to consist of 3 squares each of length lm. The mass of each square is 1kg, since the lamina is uniform. The centres of mass C,, C,and C, of the squares are, by symmetry, their

geometric centres and have coordinates (1/2,1/2),(3/2,1/2), (1/2,3/2) respectively. We take the masses of the squares to be concentrated at

these points. The centre of mass of the whole L shape (X, Y) is the centre of mass of these mass points.

Hence

x [1(1/2)+1(3/2)+10/2)|kgm 5

~ (+1+1kg -—

[[11/2)+10/2)+1(3/2)| |kgm 5

Y 5 > = -—m

(1+ 1+1)kg 6

 

The centre of mass of the L-shape lies on the line OD. We could have guessed this without cakulations. Can you tell why? Suppose, the three squares that make up the L shaped lamina of Fig. 7.11 had different masses. How will you

then determine the centre of mass of the

lamina?

 

7.3 MOTION OF CENTRE OF MASS

Equipped with the definition of the centre of mass, we are now in a position to discuss its physical importance for a system of particles.We may rewrite Eq.(7.4d) as

MR= Ym, =Snyr tr, +..4m0, (7.7

Differentiating the two sides of the equation with respect to time we get

|, dR dr, dr, dr,

Ma= mat Mage AME

or

“MV= my, 4mv, +...+,v, (7.8)

 

where v, (= dr, /dt) is the velocity of the first particle Ve {=adr,/dt)is the velocity of the second particle etc. and V = dR/di is the velocity of the centre of mass. Note that we assumed the masses m,. m,, ... etc. do not change in time. We have therefore, treated them

as constants in differentiating the equations with respect to time.

 

Differentiating Eq.(7.8) with respect to time,‘We obtain

uv _ mM, aw, mM, on +7, on

dt dt dt dt

or

‘MA =ma,+m,a,+..+m,a, (7.9)

 

where a, (= ctv, /dt) 1s the acceleration of the first particle, ‘as (=dv, /dt) is the acceleration of the second particle etc. and A(= dv /dt) is

the acceleration of the centre of mass of the system of particles.

 

Now, from Newton's second law, the force

acting on the first particle is given by F, =m_a,.The force acting on the second particle is given by F, =m.,a, and so on. Eq. (7.9) may be written

aa

MA=F,+F, +...+F, (7.10)

 

Thus, the total mass of a system of particles times the acceleration of its centre of masa is the vector sum of all the forces acting on the system of particles.

 

Note when we talk of the force F, on the first particle, ft ig net a single force, but the vector sum of all the forces on the first particle; likewise

for the secomd particle etc. Among these forces on each particle there will be external forces exerted by bodies outside the system and also internal forces exerted by the particles on one

another. We know from Newton’s third law that these internal forces occur in equal and opposite paire and in the sum of forces of Eq. (7.10),their contribution is zero. Only the external

forces contribute to the equation. We can then rewrite Eq. (7.10) as

MA=F.., (7.11)

 

where F.., represents the sum of all external forces acting on the particles of the system.

Eq. {7.11} states that the centre of masa of a system of particles moves as if all the mass of the system was concentrated at the centre of mass and all the external farces were applied at that point.

 

Notice, to determine the motion of the centre of mass no knowledge of tnternal forces of the system of particles is required; for this purpose we need to know only the external forces.To obtain Eq. (7.11) we did not need to specify the nature of the system of particles.

The system may be a collection of particles in which there may be all kinds of internal motions, or it may be a rigid bedy which has either pure translational motion or a combination of translational and rotational motion. Whatever is the aystem and the motion

of {ts individual particles, the centre of mass movea according to Eq. (7.11).

 

Instead of treating extended bodies as single particles as we have done in earlier chapters,we can now treat them as systems of particlea.We can obtain the translational component of their motion, i.e. the motion centre of maas of the system, by taking the mass of the whole system to be concentrated at the centre of mass and all the external forces on the system to be acting at the centre of masa.

 

This fa the procedure that we followed earlier in analysing forces on bodies and solving problems without explicitly outlining and justifying the procedure. We now realise that in earlier studies we assumed, without saying so,that rotational motion and/or internal motion

of the particles were either absent or negligible.We no longer need to do this. We have not only found the justification of the procedure we followed earlier; but we also have found how to describe and separate the translational motion

of (1) a rigid body which may be rotating as well, or (2) a system of particles with all kinds of internal motion.

 

Figure 7.12 is a good illustration of Eq. (7.11). A projectile, following the usual parabolic trajectory, explodes into fragments midway in air. The forces leading to the explosion are internal forces. They contribute nothing to the

motion of the centre of mass. The total external force, namely, the force of gravity acting on the body, is the same before and after the explosion.The centre of mass under the influence of the external force continues, therefore, along the same parabolic trajectory as it would have followed if there were no explosion.

 

7.4 LINEAR MOMENTUM OF A SYSTEM OF

PARTICLES

Let us recall that the linear momentum of a particle is defined as

‘p=amv (7.12)

 

Let us also recall that Newton's second law written in symbolic form for a single particle is

dp

F di (7.13)

 

where F is the force on the particle. Let us consider a system of n particles with masses m,, M,,...m, respectively and velocities V,.V.,.......¥,, Respectively. The particles may be

interacting and have external forces acting on them. The linear momentum of the first particle is m,v,, of the second particle is m.v. and so on.

 

For the system of n particles, the linear Momentum of the system is defined to be the vector sum of all individual particles of the system,

P=p, +p, +...+P,

SMV, +M,V, +...4M,¥, (7.14)

Comparing this with Eq. (7.8)

‘P=MV (7.15)

 

Thus, the total momentum of a system

of particles is equal to the product of the total mass of the system and the velocity of ita centre of mass. Differentiating Eq. (7.15)with respect to time,

‘dP y.dv

—=M——=MA

dt dt (7-16)

Comparing Eq.(7.16) and Eq. (7.11),

P

=F. (7.17)

 

This is the statement of Newton's second

law extended to a system of particles.

Suppose now, that the sum of external

forces acting on a system of particles is zero.Then from Eq.(7.17)

‘dP

*=0 or P =Constant (7.18a)

 

Thus, when the total external force acting on a system of particles is zero, the total linear momentum of the system is constant. This is the law of conservation of the total linear momentum of a system of particles. Because of Eq. (7.15), this also means that when the total external force on the system is zero the velocity of the centre of mass remains constant. (We assume throughout the discussion on systema of particles in this chapter that the total mass of the system

remains conatant.)

 

Note that on account of the internal forces,i.e. the forces exerted by the particles on one another, the individual particles may have complicated trajectories. Yet, if the total external

force acting on the system is zero, the centre of mass moves with aconstant velocity, i.c., moves uniformly in a straight line like a free particle.

 

The vector Eq. (7.18a) is equivalent to three scalar equations,

P_=¢,P,=¢ and P,=¢, (7.18 b)

 

Here P,, P, and P, are the components of

the total near momentum vector P along the % y and 2 axea respectively; ¢,, ¢, and «, are constants.

 

As an example, let us consider the

radioactive decay of a moving unstable particle,like the nucleus of radium. A radium nucleus disintegrates into a nucleus of radon and an alpha particle. The forces leading to the decay

are internal to the system and the external forces on the system are negligible. So the total linear momentum of the system is the same before and after decay. The two particles produced in the decay, the radon nucleus and

the alpha particle, move in different directions in such a way that their centre of mass moves along the same path along which the original decaying radium nucleus was moving Fig. 7.13(a)].

 

If we observe the decay from the frame of reference in which the centre of mass is at rest,the motion of the particles involved in the decay looks particularly simple; the product particles move back to back with their centre of mass

remaining at rest as shown in Fig.7.13 {b).In many problems on the system of

particles as in the above radioactive decay problem, it is convenient to work in the centre of mass frame rather than in the laboratory frame of reference.

 

In astronomy, binary (double) stars is a

common occurrence. If there are no external forces, the centre of mass of a double star moves like a free particle, as shown in Fig.7.14 (a). The trajectories of the two stars of equal

mass are also shown in the figure; they look complicated. If we go to the centre of mass frame, then we find that there the two stars are moving in a circle, about the centre of masa, which fs at rest. Note that the position of the stars have to be diametrically opposite

to each other [Fig. 7.14(b)]. Thus in our frame of reference, the trajectories of the stars are a combination of () uniform motion in a straight line of the centre of mass and (ii) circular orbits of the stars about the centre of masa.

 

As can be seen from the two examples,

separating the motion of different parts of a system into motion of the centre of mass and motion about the centre of mase ts a very useful technique that helps in understanding the motion of the system.

7.8 VECTOR PRODUCT OF TWO VECTORS

We are already familiar with vectors and their use in physics. In chapter 6 (Work, Energy,Power) we defined the scalar product of two vectors. An important physical quantity, work,

is defined as a scalar product of two vector quantities, force and displacement.We shall now define another product of two vectors. This product is a vector. Two important quantities in the study of rotational motion,namely, moment of a force and angular momentum, are defined as vector  products.Definition of Vector Product

A vector product of two vectors a and b is a vector ¢ such that magnitude ofe =c = ahsing where a and b are magnitudes of a and b and 4 is the angle between the two vectors.

 

(i) is perpendicular to the plane containing a and b.

 

(ii ifwe take a right handed screw with ite head lying in the plane of a and b and the screw perpendicular to this plane, and if we turn the head in the direction from a to b, then the tip of the screw advances in the direction

of sc. This right handed screw rule is

Mlustrated in Fig. 7.15a.

 

Alternately, if one curls up the fingers of right hand around a line perpendicular to the plane of the vectors a and b and if the fingers

are curled up in the direction from a to b, then the stretched thumb points in the direction of as shown in Fig. 7.15b.

 

A simpler version of the right hand rule is the following : Open up your right hand paln and curl the fingers pointing from a to b. Your stretched thumb points in the direction of e.

 

It should be remembered that there are two angles between any two vectors a and b. In Fig. 7.15 (a) or (b) they correspond to 0 (as shown) and (360°- §. While applying either of the above rules, the rotation should be taken

through the smaller angle (<180°) between a and b. It is 6 here.

 

Because of the cross used to denote the

vector product, it is also referred to as cross product.Note that scalar product of two vectors is commutative as said earlier, a.b = ba

 

The vector product, however, is not

commutative, ie.axbzbxa The magnitude of both a x b and b x a is the same (absing); also, both of them are

Perpendicular to the plane of a and b. But the rotation of the right-handed screw in case of axhb ie from atob, whereas in case of bx a it is from b to a. This means the two vectors are

in opposite directions. We have

axb=-bxa

 

Another interesting property of a vector

product is its behaviour under reflection.Under reflection (le. on taking the mirror image) we have x >-—x.y -yamdz—4-z.As a result all the components of a vector change sign and thus a@—>-a. bo-b.What happens to a x b under reflection?

a x b— (-a)x(-b) =axb

 

Thus, a x b does not change sign under

reflection.Both scalar and vector products are distributive with respect to vector addition.Thus,

a(b+c)=abtac

ax(b+c)=axb+axc

 

We may write c = a x b in the component

form. For this we first need to obtain some elementary cross products:

ax a=0 (0 is a null vector, i.e. a vector with zero magnitude)

 

This follows since magnitude ofa x a is

a* sin0®=0-

From this follow the results

ixi=0. jxj=0. kxk=0

i) ixj-k

 

Note that the magnitude of i x j is sin90°or 1, since j and j both have unit

magnitude and the angle between them is 90°.Thus, ix j is a unit vector. A unit vector perpendicular to the plane of ; and j and Telated to them by the right hand screw rule is ‘- Hence, the above result. You may verify similarly,

jxk=i and kxi=j From the rule for commutation of the cross product, it followa:jxie-& &xje-i ixk--j

 

Note if i,j. koccur cyclically in the above vector product relation, the vector product is positive. If i.j.k do not occur in cyclic order,the vector product is negative.

Now,

axb=(a,i+ a,j + ak) x(b.i+ b,j + bk)

= a,b, k -a,b,j- a,b. + a,b,i +a,bj- a,b,i

= (a,b, — a,b, ji+ (a,b, —a,b,)j+ (a,b, - a,b Jk

 

We have used the elementary cross products in obtaining the above relation. The expression fora xb can be put in a determinant form which is easy to remember.

. ij t

axb=la, a, a,

b, by b,

 

Example 7.4 Find the scalar and vector

products of two vectors. a = (31 - 44 + 5k)and b = (- 21+ j- 3k)

Answer

‘arb = (3i— 4j + Ske)o(-2i+ j- 3k)

=-6-4-15

=-25

. ij k

axb=|3 -4 5|=7i-j-5k

—2 1 -3

Note bxa=-7i+j+5k <

 

7.6 ANGULAR VELOCITY AND ITS RELATION WITH LINEAR VELOCITY

In this section we shall study what is angular velocity and its role in rotational motion. We have seen that every particle of a rotating body

moves in a circle. The linear velocity of the particle is related to the angular velocity. The Telation between these two quantities involves a vector product which we learnt about in the

last section.

 

Let us go back to Fig. 7.4. As said above, in rotational motion of a rigid body about a fixed axis, every particle of the body moves in a circle,

which lies in a plane perpendicular to the axis

and has its centre on the axis. In Fig. 7.16 we redraw Fig. 7.4, showing a typical particle {at a point P) of the rigid body rotating about a fixed

axis (taken as the z-axis). The particle describes a circle with a centre C on the axis. The radius of the circle is r, the perpendicular distance of the point P from the axis. We also show the

linear velocity vector v of the particle at P. It ts along the tangent at P to the circle.

 

Let P’ be the position of the particle after an interval of time At (Fig. 7.16). The angle PCP’describes the angular displacement Aé@ of the

partick: in time At. The average angular velocity of the particle over the interval Af is A@/At. As At tends to zero {i.e. takes smaller and smaller

values), the ratio A6/Atapproaches a limit which is the instantancous angular velocity d6/dt of the particle at the position P. We denote the instantancous angular velocity by (the Greek letter omega}. We know from our study of circular motion that the magnitude of linear velocity v of a particle moving in a circle is related to the angular velocity of the particle o by the simple relation v=@r, where r ts the radius of the circle.

 

We observe that at any given instant the

relation C= ©r applies to all particles of the Tigid body. Thus for a particle at a perpendicular distance r, from the fixed axis, the linear velocity

at a given instant v, is given by

vo =or, (7.19)

 

The index {runs from 1 ton, where nis the total number of particles of the body.

 

For particles on the axis, y = 0. and hence v= o@r= 0. Thus, particles on the axis are stationary. This verifics that the axis is fixed.

 

Note that we use the same angular velocity for all the particles. We therefore, refer to o as the angular velocity of the whole body.

 

We have characterised pure translation of a body by all parts of the body having the same velocity at any instant of time. Simflarly, we may characterise pure rotation by all parts of ths body having the sams angular velocity at any inatant of time. Note that this

characterisation of the rotation of a rigid body about a fied axis is just another way of saying as in Sec. 7.1 that each particle of the body moves

in a circle, which Hes in a plane perpendicular to the axis and has the centre on the axis.

 

Tn our discussion so far the angular velocity appears to be a scalar. In fact, it is a vector. We shall not justify this fact, but we shall accept

it. For rotation about a fixed axis, the angular velocity vector lies along the axis of rotation,and points out in the direction in which a right handed screw would advance, ifthe head of the

screw is rotated with the body. (See Fig. 7.17a).

 The magnitude of this vector is #= d0/di

referred as above.

We shall now look at what the vector product @ xX Fr corresponds to. Refer to Fig. 7.17(b) which is a part of Fig. 7.16 reproduced to show the path of the particle P. The figure shows the

vector o directed along the fixed (24 axis and also the position vector r= Op of the particle at P of the rigid body with respect to the origin O. Note that the origin is chosen to be on the

axis of rotation.

Now @ x r=@ x OP=a@ x(OC + CP)

But @ x OC =0 as @ is along OC

Hence @ x r=a0xCP

 

The vector w CP is perpendicular to o, Le.to the z-axis and also to CP, the radius of the circle described by the particle at P. It ta therefore, along the tangent to the circle at P.

Also, the magnitude of o x CP is w (CP) since @ and CP are perpendicular to each other. We shall denote CP by r_ and not by r, as we did earlier.

 

Thus, @ x r is a vector of magnitude or,

and is along the tangent to the circle deacribed by the particle at P. The linear velocity vector v at P has the same magnitude and direction.

Thus,

‘v=oxr (7.20)

 

In fact, the relation, Eq. (7.20), holds good even for rotation of a rigid body with one point fixed, auch as the rotation of the top [Fig. 7.6(a)].

In this case r represents the position vector of the particle with respect to the fixed point taken as the origin.

 

We note that for rotation about a fized

axis, the direction of the vector o does not change with time. Ite magnitude may,

however, change from instant to instant. For the more general rotation, both the

magnitude and the direction of o may change from instant to instant.

 

7.6.1 Angular acceleration You may have noticed that we are developing the study of rotational] motion along the lines

of the atudy of translational motion with which we are already familar. Analogous to the kinetic variables of linear displacement and velocity (v)

in translational motion, we have angular

displacement and angular velocity ()} in

rotational motion. It is then natural to define in rotational motion the concept of angular acceleration in analogy with linear acceleration defined as the time rate of change of velocity in translational motion. We define angular

acceleration as the time rate of change of angular velocity; Thus,de

oat (7.21)

 

If the axis of rotation is fixed, the direction of and hence, that of a is fixed. In this case the vector equation reduces to a scalar equation

da

an (7.22)

 

7.7 TORQUE AND ANGULAR MOMENTUM

In this section, we shall acquaint ourselves with two physical quantities which are defined as vector products of two vectors. These as we shall see, are especially important in the discussion

of motion of systems of particles, particularly rigid bodies.

 

7.7.1 Moment of force (Tarque)

We have learnt that the motion of a rigid body in general ts a combination of rotation and translation. If the body is fixed at a point or along a line, it has only rotational motion. We know that force is needed to change the translational state of a body, i.e. to produce linear acceleration. We may then ask, what is the analogue of force in the case of rotational motion? To lock into the question in a concrete

situation let us take the example of opening or closing of a door. A door is a rigid body which can rotate about a fixed vertical axis passing through the hinges. What makes the door rotate? It is clear that unless a force is applied

the door does not rotate. But any force does not do the job. A force applied to the hinge line cannot produce any rotation at all, whereas a force of given magnitude applied at right angles

to the door at ite outer edge is most effective in producing rotation. It is not the force alone, but how and where the force is applied is important

in rotational motion.

 

The rotational analogue of force is moment of force. It is also referred to as torque or couple. (We shall use the words moment of force and torque interchangeably.) We shall firat

define the moment of force for the special case of a single particle. Later on we shall extend the concept to systems of particles including rigid bodies. We shall also relate it to a change in the state of rotational motion, Le. is angular acceleration of a rigid body.

 

Ifa force acts on a single particle at a point P whose position with respect to the origin O is given by the poattion vector r (Fig. 7.18), the moment of the force acting on the particle with

respect to the origin O ts defined as the vector product

t=FrxF (7.23)

 

The moment of force (or torque) is a vector quantity. The symbol + stands for the Greek letter tax The magnitude of < is s=7 Fain (7.248)

 

where r is the magnitude of the position vector F, 1.¢. the length OP, Fis the magnitude of force F and is the angle between r and F as shown.

 

Moment of force has dimensions M L? T?.

Its dimensions are the same as those of work or energy. It is, however, a very different physical quantity than work. Moment of a force is a vector, while work is a scalar. The SI unit of

moment of force is newton metre (N m)}. The magnitude of the moment of force may be written

s=(rsin@é)F=arF (7.24b)

or t=rFsin@=rF (7.240)

 

where 7, =rsin@ia the perpendicular distance of the line of action of F form the origin and F\(= F sin@)is the component of F in the direction perpendicular to r. Note that ¢ = 0 if

r=0, F=0 or 6= 0° or 180° . Thus, the moment of a force vanishes if either the magnitude of the force is zero, or if the line of action of the force passes through the origin.

 

One may note that since rx F is a vector

product, properties of a vector product of two vectors apply to it. If the direction of F is reversed, the direction of the moment of force

is reversed. If directions of both r and F are reversed, the direction of the moment of force remains the same.

 

7.7.2 Angular momentum of a particle

Just as the moment of a force is the rotational analogue of force, the quantity angular momentunn is the rotational analogue of linear

momentum. We shall first define angular

momentum for the special case of a single Particle and look at its usefulness in the context of single particle motion. We shall then extend

the definition of angular momentum to systems of particles including rigid bodies.

 

Like moment ofa force, angular momentum

is also a vector product. It could also be referred to as moment of (linear) momentum. From this term one could guess how angular momentum is defined.

 

Consider a particle of mass m and linear

momentum p at a position r relative to the origin O. The angular momentum 1 of the particle with respect to the origin O is defined to be

l=rxp (7.25a)

 

The magnitude of the angular momentum

vector is  l=rpsin@g (7.26a)where pis the magnitude of p and @1is the angle between r and p. We may write

l=rp, or rnp (7.26b)

 

where r, (=rainé) is the perpendicular distance of the directional line of p from the origin and Pp, psin@) is the component of p in a direction

perpendicular to r. We expect the angular momentum to be zero (f = 0), if the linear momentum vanishes (p = 0), if the particle is at the origin (r = 0), or if the directional line of p

passea through the origin @ = 0° or 180°.

 

The physical quantities, moment of a force and angular momentum, have an important relation between them. It is the rotational analogue of the relation between force and linear momentum. For deriving the relation in the context of a single particle, we differentiate

1=rx p with respect to time,a _idyy )

aoa?

 

Applying the product rule for differentiation to the right hand side,

Ste xp)=Axpirx®

 

Now, the velocity of the particle is v = dr/dt and p=mv

‘dr

 

Because of this ax*Poyxm v=0,

as the vector product of two parallel vectors vanishes. Further, since dp / dt =F,

rx oP ex Rae

dt

Hence “(¢ Xp)=t

dt pi

‘d

or aot (7.27)

 

Thus, the time rate of change of the angular momentum of a particle is equal to the torque acting on it. This is the rotational analogue of the equation F = dp/dt, which expresses Newton's second law for the translational motion

of a single particle.

 

Torque and angular momentum for a system

of particles To get the total angular momentum of a system of particles about a given point we need to add vectorially the angular momenta of individual

particles. Thus, for a system of n particles,

L=1+h+..+1,=¥1

il

 

The angular momentum of the & particle

is given by

L=,x P,

where r, is the position vector of the f particle with respect to a given origin and p = (my) 1s the linear momentum of the particle. (The 4a experiment with the bicycle rim Take a bicycle rim

and extend its axle on both sides.Tie two atrings at both ends A and B,as shown in the adjoining figure. Hold

both the strings together in one hand such that the rim is vertical. If you

Jeave one string. the rim will tilt. Now keeping the rim in vertical position with both the strings in one hand, put the wheel in fast rotation around the axle with the other hand. Then leave

one string, say B, from your hand, and observe what happens.

 

The rim keeps rotating in a vertical plane and the plane of rotation turus around the string A which you are holding. We say that the axis of rotation of the rim or equivalently

its angular momentum precesses about the

string A.

 

The rotating rim gives riee to an angular momentum. Determine the dtrection of this angular momentum. When you are holding the rotating rim with string A. a torque is generated.

[We leave it to you to find out how the torque is generated and what ite direction is.) The effect of the torque on the angular momentum is to

make it precess around an axis perpendicular to both the angular momentum and the torque.Verify all these statements.particle has mass m, and velocity v,) We may Write the total angular momentum of a system

of particles as

L=Y 1-5 ~P, (7.25)

t

 

This is a generalisation of the definition of angular momentum (Eq. 7.25a) for a single Particle to a system of particles.

Using Eqs. (7.23) and (7.25b), we get

dL od dl,

ae dh h)= Dg Ls (7.288)

 

where 1,18 the torque acting on the @ particle;

1, =4XF,

 

The force F,on the f particle 1s the vector sum of external forces F;“' acting on the particle and the internal forces F'"" exerted on it by the

other particles of the system. We may therefore separate the contribution of the external and the internal forces to the total torque

ce Tot Faas

where Tex = Dy x F™

and Tu = > x F*

 

We shall assume not only Newton's third

law, i.e. the forces between any two particles of the system are equal and opposite, but also that these forces are directed along the line joining

the two particles. In this case the contribution of the internal forces to the total torque on the system is zero, since the torque resulting from each action-reaction pair of forces is zero. We thus have, +, = 0 and therefore t =+_,Since t= )'¢,, it follows from Eq. (7.28a)that * = Text (7.28 b)

 

Thus, the time rate of the total angular

momentum of a system of particles about a point (taken as the origin of our frame of reference) is equal to the sum of the external torques (1.c. the torques due to external forces)

aoting on the system taken about the same point. Eq. (7.28 b) is the generalisation of the single particle case of Eq. (7.23) to a system of

particles. Note that when we have only one particle, there are no internal forces or torques.Eq.(7.28 b) is the rotational analogue of ‘d FR (7.17

 

Note that like Eq.(7.17), Eq.(7.28b) holds good for any syatem of particles, whether it is a rigid body or its individual particles have all kinds of internal motion.

 

Answer Let the particle with velocity v be at point P at some instant f. We want to calculate the angular momentum of the particle about an arbitrary point.

 

The angular momentum is 1 = r x nw. Its

Magnitude is mur sin@, where 6 is the angle between r and v as shown in Fig. 7.19. Although the particle changes position with time, the line of direction of v remains the same and hence OM =r sin 6. is a constant.

 

Further, the direction of 1 is perpendicular to the plane ofr and v. It is into the page of the figure.This direction does not change with time.

 

Thus, l remains the same in magnitude and direction and is therefore conserved. Is there any external torque on the particle? <

 

7.8 EQUILIBRIUM OF A RIGID BODY

We are now going to concentrate on the motion of rigid bodies rather than on the motion of general systems of particles.

 

We shall recapitulate what effect the

external forces have on a rigid body. (Henceforth we shall omit the adjective ‘external’ because unless stated otherwise, we shall deal with only

external forces and torques.) The forces change the translational state of the motion of the rigid body, Le. they change its total near momentum

in accordance with Eg. (7.17). But this is not the only effect the forces have. The total torque on the body may not vanish. Such a torque changes the rotational state of motion of the

rigid body, i.e. it changes the total angular Momentum of the body in accordance with Eq.(7.28 b).

 

A rigid body is said to be in mechanical

equilibrium, if both its linear momentum and angular momentum are not changing with time,or equivalently, the body has neither linear acceleration nor angular acceleration. This means

 

(1) the total force, i.e. the vector sum of the forces, on the rigid body is zero;F,+F,+..+F,= )F,=0 (7.30)

If the total force on the body is zero, then the total Inear momentum of the body does not change with time. Eq. (7.30a) gives the condition for the translational equilibrium of the body.

 

(2) The total torque, i.e. the vector sum of the torques on the rigid body is zero,Hth+u4+t, =) t, =O (7.30b)

 

If the total torque on the rigid body is zero,the total angular momentum of the body docs not change with time. Eq. (7.30 b) gives the condition for the rotational equilibrium of the body.

 

One may raise a question, whether the

rotational equilibrium condition [Eq. 7.30(b)]remains valid, if the origm with respect to which the torques are taken is shifted. One can show that if the translational equilfbrium condition

[Eq. 7.30{a)) holds for a rigid body, then such a shift of origin does not matter, i.e. the rotational equilibrium condition ia independent of the location of the origin about which the torques

are taken. Example 7.7 gives a proof of this result in. a special case ofa couple, i.e. two forces acting on a rigid body in translational equilibrium. The generalisation of this result to

n forces is left as an exercise.

Eq. (7.30a) and Eq. (7.30b), both, are vector equations. They are equivalent to three scalar equations each. Eq. (7.30a) corresponds to dF =0, LF, =0 and DA.=0 (7.31a)where F,, F, and Ff, are respectively the x, y and z components of the forces F.. Similarly,Eq. (7.30b) is equivalent to three scalar

equations

nh nt n

 

Dtm=0, Bt =O and Lt. (7.310)

where +,, 7, and t,are respectively the x, y and z components of the torque f, .

 

Eq. (7.314) and (7.31b) give six independent conditions to be satisfied for mechanical equilfbrium of a rigid body. In a number of problems all the forces acting on the body are coplanar. Then we need only three conditions

to be aatisfied for mechanical equilibrium. Two of these condftions correspond to translational equilfbrium; the sum of the components of the forces along any two perpendicular axes in the

plane must be zero. The third condition

corresponds to rotational equilfbrium. The sum of the components of the torques along any axis perpendicular to the plane of the forces must be zero.

 

The conditions of equilibrium ofa rigid body may be compared with those for a particle,which we considered in earlier chapters. Since consideration of rotational motion does not apply to a particle, only the conditions for

translational equilfprium (Eq. 7.30 a) apply to a particle. Thus, for equilibrium of a particle the vector sim of all the forces on ft must be

zero. Since all these forces act on the single particle, they must be  concurrent. Equilfbrium under concurrent forces was discussed in the earlier chapters.

 

Abody may be in partial equilfbrium, j.e., it may be in translational equilibrium and not in rotational equilibrium, or it may be in rotational

equilibrium and not in translational

equilforium.

 

Consider a light {1.c. of negligible mass) rod {AB), at the two enda (A and B) of which two parallel forces both equal in magnitude are applied perpendicular to the rod as shown in

Fig. 7.20(a).

——— nn a B

c

C v

Fig. 7.20 fa)

 

Let C be the midpoint of AB, CA = CB = a.the moment of the forces at A and B will both be equal in magnitude (aF), but opposite in sense as shown. The net moment on the rod will be zero. The system will be in rotational equilfbrium, but it will not be in translational equilibrium; ) F 4 0

 

The force at B in Fig. 7.20{a) is reversed in Fig. 7.20{b). Thus, we have the same rod with two equal and opposite forces applied perpendicular to the rod, one at end A and the other at end B. Here the moments of both the forces are equal, but they are not opposite; they

act in the same sense and cause anticlockwise rotation of the rod. The total force on the body is zero; so the body is in translational equilfbrium; but it is not fn rotational equilibrium. Although the rod is not fixed in

any way, it undergoes pure rotation (i.e. rotation without translation).

 

A pair of equal and opposite forces with

different lines of action is known as a couple or torque. A couple produces rotation without translation.

 

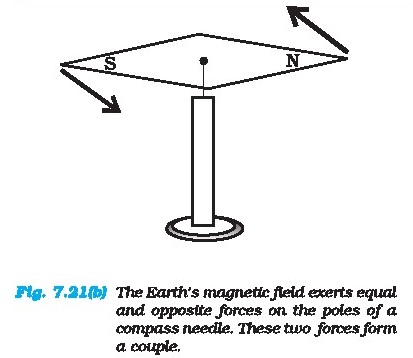
When we open the lid of a bottle by turning it, our fingers are applying a couple to the lid [Fig. 7.21(a)]. Another known example is a compaaa needle in the earth's magnetic field as

shown in the Fig. 7.21(h). The earth's magnetic field exerts equal forces on the north and south poles. The force on the North Pole is towards the north, and the force on the South Pole is toward the south. Except when the needle points

in the north-south direction; the two forces do not have the aame Hine of action. Thus there is a couple acting on the needle due to the earth's magnetic field. ;

 

Example 7.7 Show that moment of a

couple does not depend on the point about which you take the moments.

 

Anawer Consider a couple as shown in Fig. 7.22 acting on a rigid body. The forces F and -F act respectively at points B and A These pointe have

posttion vectors r, and r, with respect to originO. Let us take the moments of the forces about the origin.

 

The moment of the couple = sum of the

moments of the two forces making the couple

=2,x(-F)+4r,*F

=r,xF-9r,xF

= (r,-2,) x F

Butz, + AB =z,, and hence AB =r, -f,.

 

The moment of the couple, therefore, is

AB x F.Clearly this ts independent of the origin, the point about which we took the moments of the forces. <

 

7.8.1 Principle of moments

An ideal lever ia essentially a light (i.e. of negligible mass) rod pivoted at a point along ita length. This point is called the fulcrum. A see-saw on the children’s playground is a typical

example of a lever. Two forces F, and F,, parallel to each other and usually perpendicular to the lever, aa shown here, act on the lever at distances d, and d, respectively from the fulcrum as shown in Fig. 7.23.

 

The lever is a system in mechanical

equilibrium. Let R be the reaction of the support at the fulcrum; R is directed opposite to the forces F, and F,. For translational equilibrium,

R-F,-F,=0 @

 

For considering rotational equilibrium we take the moments about the fulcrum; the sum of moments must be zero,

GF, - 2,F,=0 (i Normally the anticlockwise (clockwise)moments are taken to be positive (negative). Note

Racts at the fulcrum itself and has zero moment about the fulcrum.

 

In the case of the lever force F, is usually some weight to be lifted. It is called the load and its distance from the fulcrum d, is called the load arm. Force F, is the effort applied to lift

the load; distance d, of the effort from the fulcrum is the effort arm.

Eq. (i) can be written as

dF. =d,F, (7.32)

or load arm x load = effort arm x effort

 

The above equation expresses the principle of moments for a lever. Incidentally the ratio F,/F,1s called the Mechanical Advantage (M.A);

F_d,

MASE G (7.32b)

 

If the effort arm d, is larger than the load arm, the mechanical advantage is greater than one. Mechanical advantage greater than one means that a small effort can be used to lift a large load. There are several examples of a lever

around you besides the see-saw. The beam ofa balance is a lever. Try to find more such examples and identify the fulcrum, the effort and effort arm, and the load and the load arm of the lever in each case.You may easily show that the principle of moment holds even when the parallel forces F,and F, are not perpendicular, but act at some angle, to the lever.

 

7.8.2 Centre of gravity

Many of you may have the experience of

balancing your notebook on the tip of a finger.Figure 7.24 illustrates a similar experiment that you can easily perform. Take an irregular-shaped cardboard and a narrow tipped object like a pencil. You can locate by trial and error a point G on the cardboard where it can be

balanced on the tip of the pencil. (The cardboard remains horizontal in this position.) This point of balance is the centre of gravity (CG) of the cardboard. The tip of the pencil provides a

vertically upward force due to which the

cardboard is in mechanical equilibrium. As shown in the Fig, 7.24, the reaction of the tip is equal and opposite to Mg, the total weight of (i.e., the force of gravity on) the cardboard and

hence the cardboard is in translational

equilibrium. It is also in rotational equilfbrium; if it were not so, due to the unbalanced torque it would tilt and fall. There are torques on the card board due to the forces of gravity like mg,mg .... etc, acting on the individual particles that make up the cardboard.

 

The CG of the cardboard is so located that the total torque on ft due to the forces mg, mg a. te. is Zero,

 

If r, is the position vector of the tth particte ofan extended bady with respect to its CG, then the torque abont the CG, due to the force of gravity on the particle is t,= 1, x mg. The total

gravitational torque about the CG fs zero, Le.

t= t= Nrxmg=0 (7.33)

 

We may thereiore, define the CG of a body as that point where the total gravitational torque on the body is zero.

 

We notice that in Eq. (7.33), g is the same for all particles, and hence it comes out of the summation. This gives, since g is non-zero,¥ nx, =. Remember that the posttion vectors (r) are taken with respect to the CG. Now, in

accordance with the reasoning given below Eq. (7.4a] in Sec. 7.2, if the sum is zero, the origin must be the centre of mass of the bedy.Thus, the centre of gravity of the body cotacides with the centre of mass in uniform gravity or

gr

avity-free space. We note that this is true because the body being small, g does not

 vary from one point of the body to the other. If the body is ac extended that g varies from part to part of the body, then the centre of gravity

and centre of maas will not coincide. Basically,the two are different concepts. The centre of mass has nothing to do with gravity. It depends only on the distribution of mass of the body.

 

In Sec. 7.2 we found out the position of the centre of mass of several regular, homogeneous objects. Obviously the method used there gives us also the centre of gravity of these bodies, if

they are small enough.

 

Figure 7.25 illustrates another way of

determining the CG of an regular shaped body like a cardboard. If you suspend the body from some point like A, the vertical line through A passea through the CG. We mark the vertical AA,. We then suapend the body through other

points like B and C. The intersection of the verticals gives the CG. Explain why the method works. Since the body is small enough, the method allows us to determine also its centre of mass.

 

Example 7.8 A metal bar 70 cm long

and 4.00 kg in mass supported on two

knife-edgea placed 10 cm from each end.

A6.00 kg load is suspended at 30 cm from

one end. Find the reactions at the knife-edges. (Assume the bar to be of untform crosa section and homogeneous.)

Answer Figure 7.26 shows the rod AB, the positions of the knife edges K, and K, , the centre of gravity of the rod at G and the suspended load at P.

 

Note the weight of the rod W acts at its

centre of gravity G. The rod is uniform in cross section and homogeneous; hence G is at the centre of the rod; AB = 70 cm. AG = 35 cm, AP = 30 cm, PG = 5.cm, AK = BK, = 10 cm and K,G = KG = 25 cm. Also, W= weight of the rod =4.00 kg and W,= suspended load = 6.00 kg;R, and R, are the normal reactions of the support at the knife edges.

 

For translational equilibrium of the rod,R,+R,-W,-W=0 0 Note W, and W act vertically down and R,and R, act vertically up.

 

For considering rotational equilibrium, we take moments of the forces. A convenient point to take moments about is G. The moments of R, and W, are anticlockwise {+ve), whereas the

moment of R, {a clockwise (-ve).

For rotational equilibrium,

-R, (K,G) + W, (PG) + R, (K,G) = 0 (i

It ia given that W = 4.00g N and W, = 6.00g N, where g = acceleration dune to gravity. We take g = 9.8 m/s’.With numerical values inserted, from (i)

R,+ R, - 4.00g- 6.009 = 0

or R + K, = 10.00g N (iif)

= 98.00 N

From (ii), - 0.25 R, + 0.05 W, + 0.25 R,=0

or R, - R, = 1.2g N=11.76N (tv)

From (ft) and (iv), R, = 54.88 N,

R,=43.12N

Thus the reactions of the aupport are about 55 N at K and 43 N at K.. <

 

Example 7.9 A 3m long ladder weighing

20 kg leans on a frictionless wall. Its feet rest on the floor 1 m from the wall as shown in Fig.7.27. Find the reaction forces of the wall and the floor.

 

The ladder AB is 3 m long, its foot A is at distance AC = 1 m from the wall. From

Pythagoras theorem, BC = 2./2 m. The forces on the ladder are its weight W acting at its centre of gravity D, reaction forces F, and F, of the wall

and the floor respectively. Force F, is

perpendicular to the wall, since the wall is frictionless. Force F, is resolved into two components, the normal reaction N and the force of friction F: Note that F prevents the ladder from sliding away from the wall and is therefore directed toward the wall.

 

For translational equilibrium, taking the forces in the vertical direction,

N-W=0 (a)Taking the forces in the horizontal direction,F-F,=0 (ii)

 

For rotational equilibrium, taking the

moments of the forces about A,

2f2 F, - (1/2) W=0 (ii

Now W= 20 g=20x9.8N= 196.0N

From () N= 196.0

From (if) F = W/4/2 = 196.0/4V2 =34.6N

From fi) F = F, =34.6N

F, =-¥F° 4+ N° =199.0N

The force F, makes an angle @ with the

horizontal,tan@=N/F=4J2, a@=tan(4¥2)=80

 

7.8 MOMENT OF INERTIA

We have already mentioned that we are

developing the study of rotational motion parallel to the study of translational motion with which we are familiar. We have yet to answer

one major question in this connection. What is the analogue of mass in rotational motion?

We shall attempt to answer this question in the present section. To keep the discussion simple,we shall consider rotation about a fixed axis only. Let us try to get an expression for the

Kinetic energy of a rotating body. We know that for a body rotating about a fixed axis, each particle of the body moves in a circle with linear velocity given by Eq. (7.19). (Refer to Fig. 7.16).For a particle at a distance from the axis, the linear velocity is v, = @. The kinetic energy of motion of this particle is k =i my? ime

1 Qt ~” Q 0°

 

where m,is the masse of the particle. The total kinetic energy K of the body is then given by the sum of the kinetic energies of individual particles,

K=>k = 5 Lincie)

iol tel

 

Here nis the number of particles in the body.Note ois the same for all particles. Hence, taking out of the sum,

Kate (Sime)

2 ‘fe

 

We define a new parameter characterising

the rigid body, called the moment of inertia J,given by

I= > mr (7.34)

tel

With this definttion,

‘el ae

Kepler (7.35)

 

Note that the parameter J is independent of the magnitude of the angular velocity. It is a characteristic of the rigid body and the axis about which it rotates.

 

Compare Eq. (7.35) for the kinetic energy of a rotating body with the expression for the kinetic energy of a body in linear (translational)

motion,

K=imv

2

 

Here m is the mass of the body and uv is its velocity. We have already noted the analogy between angular velocity «(in respect ofrotational motion about a fixed axis) and linear velocity u (in

respect of linear motion). It is then evident that the parameter, moment of inertia fF, is the desired rotational analogue of mass. In rotation (about a

fixed axis), the moment of inertia plays a similar Tole as mass does in inear motion.

 

We now apply the definition Eq. (7.34), to calculate the moment of inertia in two simple cases.

 

(a) Consider a thin ring of radius R and mass M, rotating in its own plane around its centre with angular velocity #. Each mass element of the ring is at a distance R from the axis, and moves with a speed Ra. The kinetic energy is therefore,Lo lage

Ke= 2 My = 2 MR‘ ar

Comparing with Eq. (7.35) we get I = MR?

for the ring.1

 

(b) Next, take a rigid massless rod of length ¢ with a pair of small masses, rotating about an axis through the centre of mass perpendicular to the rod (Fig. 7.28). Each mass M/2 is at a distance t/2 from the axis.

 

The moment of inertia of the masses is

therefore given by

(M/2) (1/2)? + (M/2)(1/ 2)?

 

Thus, for the pair of masses, rotating about the axis through the centre of mass perpendicular to the rod

I= MP/4 Table 7.1 gives the moment of inertia of various familiar regular shaped solids about specific axes.

 

As the mass of a body resists a change in its atate of linear motion, it is a meaaure of its inertia in linear motion. Similarly, aa the moment of inertia about a given axis of rotation

resists a change in tte rotational motion, ft can be regarded as a measure of rotational inertia of the body; it is a measure of the way in which

different parts of the body are distributed at different distances from the axis. Unlike the mass of a body, the moment of inertia is not a fixed quantity but depends on the orientation

and position of the axis of rotation with respect to the body as a whole. As a measure of the way in which the maas of a rotating rigid body is distributed with respect to the axis of rotation,

we can define a new parameter, the radius of gyration. It is related to the moment of inertia and the total mass of the body.

 

Notice from the Table 7.1 that in all

cases, we can write I = Mk*, where k has

the dimension of length. For a rod, about the perpendicular axis at its midpoint,i =V/l2.ie. k? =L/V12 . Similarly, k = R/2 for the circular disc about its diameter. The length iis a geometric property of the body and

axis of rotation. It is called the radius of @yration. The radius of gyration of a body about an axis may be defined as the distance from the axia of a mass point whose masa is equa] to the mass of the whole body and whose

moment of inertia is equal to the moment of inertia of the body about the axis.

 

Thus, the moment of inertia of a rigid body depends on the mass of the body, its shape and size; distribution of mass about the axis of rotation, and the posttion and orientation of the

axis of rotation.

 

From the definition, Eq. {7.34), we can infer that the dimensions of moments of inertia we ML? and its SI units are kg m?.

 

The property of this extremely important

quantity J as a measure of rotational inertia of the body has been put to a great practical use.The machines, such as steam engine and the automobile engine, etc., that produce rotational

motion have a disc with a large moment of inertia, called a flywheel. Because of ita lange moment of inertia, the flywheel resists the sudden increase or decrease of the speed of the vehicle. It allows a gradual change in the speed

and prevents jerky motions, thereby ensuring asmooth ride for the passengers on the vehicle.

 

7.10 THEOREMS OF PERPENDICULAR AND

PARALLEL AXES

These are two useful theorema relating to moment of inertia. We shall first discuss the theorem of perpendicular axes and its simple yet instructive application in working out the

moments of inertia of some regular-shaped bodies.

 

Theorem of perpendicular axes

This theorem is applicable to bodies which are planar. In practice this means the theorem applies to flat bodies whose thickness is very small compared to their other dimensions (e.g.length, breadth or radius). Fig. 7.29 illustrates the theorem. It states that the moment of inertia of a planar body (lavaina) about an axis perpendicular to its plane is equal to the sam of its moments of inertia about two perpendicular axes concurrent with

perpendicular axis and lying in the plane of the body.

 

The figure showa a planar body. An axis

perpendicular to the body through a point O ts taken as the z-axis. Two mutually perpendicular axes lying in the plane of the body and concurrent with 2-axis, i.e. passing through O,are taken as the x and y-axes. The theorem

states that Lal +l, (7.36)

Let us look at the usefulness of the theorem through an example.

 

Example 7.10 What is the moment of

inertia of a disc about one ofits diameters?

Answer We aasume the moment of inertia of the disc about an axis perpendicular to ft and through its centre to be known; it is MR /2,where M is the mass of the diac and R is its tadtus (Table 7.1)

 

The disc can be considered to be a planar body. Hence the theorem of perpendicular axes is applicable to it. As shown in Fig. 7.30, we take three concurrent axes through the centre

of the disc, O as the x,y,z axes; x and y-axes lie in the plane of the disc and z is perpendicular to it. By the theorem of perpendicular axes,Leal. +1,

 

Now, x and y axes are along two diameters of the disc, and by symmetry the moment of inertia of the disc is the same about any diameter. Hence

L=l,

and I= 21,

But i= MR/2

So finally, [,=1/2= MR/4

 

Thus the moment of inertia of a disc about any of its diameter is MR°/4 . <

Find similarly the moment of inertia of a ring about any ofits diameter. Will the theorem be applicable to a solid cylinder?

7.10.1 Theorem of parallel axes

This theorem is applicable to a body of any shape. It allows to find the moment of inertia of a body about any ands, given the moment of inertia of the body about a parallel axis through the centre of mass of the body. We shall only

state this theorem and not give its proof. We shall, however, apply it to a few simple situations which will be enough to convince us about the

usefulness of the theorem. The theorem may be stated as follows:

 

The moment of inertia of a body about any axis is equal to the sum of the moment of inertia of the body about « parallel axis passing through its centre of mass and the product of ite mass and the square of the distance between the two parallel axes. As shown in the Mig. 7.31, z and 2 are two parallel axes separated by a distance a. The z-axis passes through the centre of mass O of the rigid body.Then according to the theorem of parallel axes L=h+M@ (7.37)

where J, and Fare the moments of inertia of the body about the z and 2’ axes respectively, Mis the total mass of the body and ais the perpendicular distance between the two parallel axes.

 

Example 7.11 What is the moment of

inertia of a rod of mass M, length l about an axis perpendicular to it through one end?

Answer For the rod of mass M and length lt,I= MP/12. Using the parallel axes theorem,P=! + Me with a=t/2 we get,

remen(s) aM 12 2 3

 

We can check this independently since I 1s half the moment of inertia of a rod of mass 2M and length 21 about its midpoint,Peomteyi MO 4

12 2 3

 

Example 7.12 What is the moment of

inertia of a ring about a tangent to the

circle of the ring?

Answer The tangent to the ring in the plane of the ring is parallel to one of the diameters of the ring.

 

Example 7.13 Obtain Eq. (7.38) from first principles.

 

Answer The angular acceleration is uniform,hence a = a= constant {i)

Integrating this equation,a= Ja di+c

‘=at+c (as a is constant)

Att=0, w= a, (given)From (f we get at t= 0, m=c= am,

 

Thus, @ = ot + m, as required.

With the definition of = d8/dt we may

integrate Eq. (7.38) to get Eq. (7.39). This derivation and the derivation of Eq. (7.40) is left as an exercise.

 

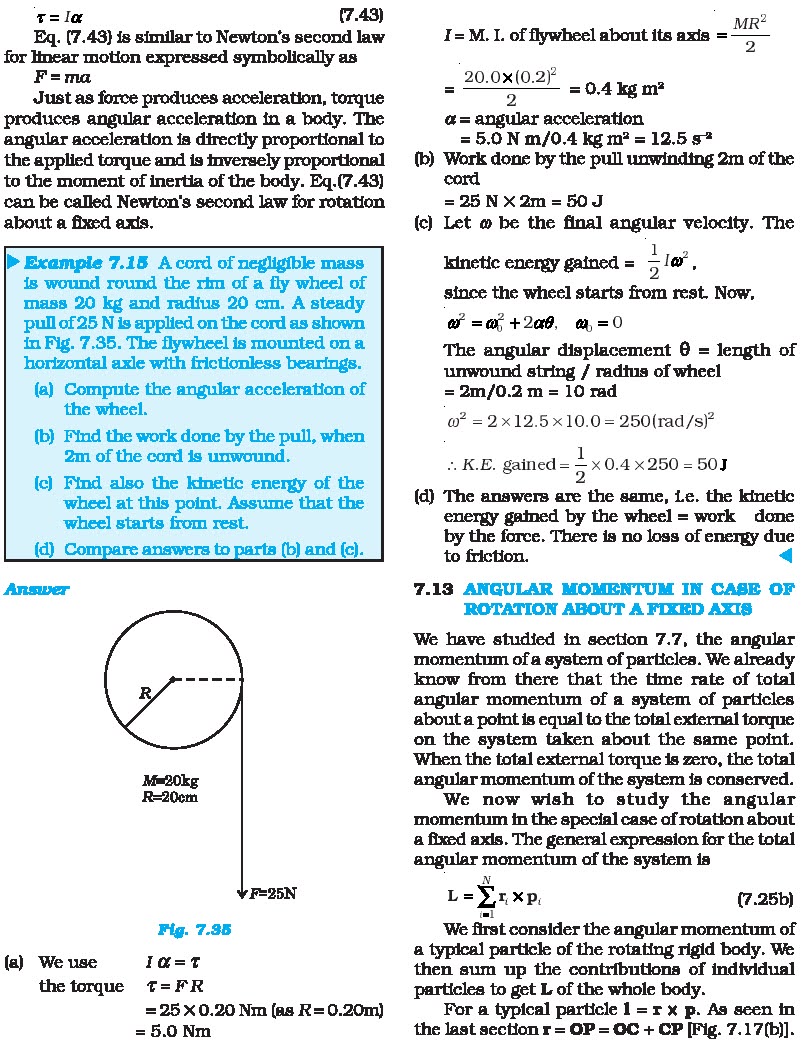
Example 7.14 The angular speed of a

motor wheel is increased from 1200 rpm

to 3120 rpm in 16 seconds. {i} What is its angular acceleration, assuming the

acceleration to be uniform? (if) How many revolutions does the engine make during this time?

Answer We shall use » = o,+ at , = initia] angular speed in rad/s = 2n*x angular speed in rev/s ‘Qn x angular speed in rev/min = 60 s/min

‘2nx 1200 = —— rad/s

60

= 40% rad/s

Similarly @ = final angular speed in rad/s = 283120 rad/s

60

= 2nx 52 rad/s

= 104 rrad/s

“ Angular acceleration

a= Soh =4 rad/s?

The angular acceleration of the engine

= 4 rad/s?

(i The angular displacement in time t is

given by

@=a,t+ +a e

2

= (408x164+5x 4=x 16") rad

=(640#% +512") rad

= 11522 rad

Number of revolutions = ot 2576 4

 

7.12 KINEMATICS OF ROTATIONAL MOTION

ABOUT A FIXED AXIS

Table 7.2 lists quantities associated with linear motion and their analogues in rotational motion.We have already compared kinematics of the two motions. Also, we know that in rotational

motion moment of inertia and torque play the same role as mass and force respectively in linear motion. Given this we should be able to guess what the other analogues indicated in the

table are. For example, we know that in linear motion, work done is given by F dx, in rotational motion about a fixed axis it should be rg,since we already know the correspondence ‘dx > d@ and F +. It is, however, necessary that these correspondences are established on

sound dynamical considerations. This is what Wwe now turn to.

 

Before we begin, we note a  simplification that arises in the case of rotational motion about a fixed azis. Since the axis ts fixed, only those components of torques, which are along

the direction of the fixed axis need to be considered in our discussion. Only these components can cause the body to rotate about the axis. A component of the torque perpendicular to the axis of rotation will tend to turn the axis from its posttion. We specifically assume that there will arise necessary forces of constraint to cancel the effect of the perpendicular components of the (external)torques, so that the fixed position of the axis will be maintained. The perpendicular components of the torques, therefore need not be taken into account. This means that for our

calculation of torques on a rigid body:

(1) We need to consider only those forces that lie in planes perpendicular to the axis.Forces which are paralle] to the axis will give torques perpendicular to the axis and need not be taken into account.

 

(2) We need to consider only those components of the position vectors which are perpendicular to the axis.Components of position vectors along the axis will result in torques perpendicular to the axis and need not be taken into account.

 

Figure 7.34 shows a cross-section ofa rigid body rotating about a fixed axis, which is taken as the z-axis  (perpendicular to the plane of the

page; see Fig. 7.33). As said above we need to consider only those forces which lie in planes perpendicular to the axds. Let F, be one such typical force acting as shown on a particle of the body at point P, with ita line of action in a

Plane perpendicular to the axis. For convenience we call this to be the x~y’ plane (coincident with the plane of the page). The particle at P,describes a circular path of radius r, with centre

C on the axis; CP, =7F,.

 

In time Af, the point moves to the poattion P,’. The displacement of the particle ds,,therefore, haa magnitude ds, = rd@ and direction tangential at P, to the circular path as shown. Here d@ is the angular displacement of the particle, d@= <P,CP, .The work done by

the force on the particle ts AW, =F,. ds,= F.ds, cos¢,= F.(r, dQsina,where ¢, is the angle between F, and the tangent

at P, and @, is the angle between F, and the radius vector OP,; ¢, + &, = 90°.

 

The torque due to F, about the origin is

OP, x F,. Now OP, = OC + OP,. [Refer to

Fig. 7.17(b).] Since OC is along the axis, the torque resulting from it is excluded from our consideration. The effective torque due to F, is +,= CPx F ; it is directed along the axis of rotation and has a magnitude ¢,= 7,F, sine , Therefore,dw, =7,de

 

If there are more than one forces acting on the body, the work done by all of them can be added to give the total work done on the body.Denoting the magnitudes of the torques due to the different forcea aa ¢,, f,, ... etc,

‘dW =(t, +t, +...)d@

 

Remember, the forces giving rise to the

torques act on different particles, but the angular displacement d@ ia the same for all particlea. Since all the torques considered are parallel to the fixed axia, the magnitude tof the total torque is just the algebraic sum of the

magnitudes of the torques, 1e., t= 4+ 1, + .....We, therefore, have ‘dW =1de (7.41)

 

This expression givea the work done by the total (external) torque + which acts on the body rotating about a fixed axis. Ita similarity with the corresponding expression dW=Fds

for linear (translational) motion is obvious.Dividing both sides of Eq. (7.41) by dt gives

P ow _ oe _ TO)

dt dt

or P= (7.42)

 

This is the instantaneous power. Compare

this expression for power in the case of

rotational motion about a fixed axis with the expression for power in the case of near motion,

P=Fu

 

Ina perfectly rigid body there is no internal motion. The work done by external torques is therefore, not dissipated and goes on to increase

the kinetic energy of the body. The rate at which work is done on the body is given by Eq. (7.42).This is to be equated to the rate at which kinetic

energy increases. The rate of increase of kinetic energy is

(te) (20) deo

dt\ 2. 2 di

 

We assume that the moment of inertia does uot change with time. This means that the mass of the body does not change, the body remains rigid and also the axis does not change its position with respect to the body.

Since a=dw/dt. we get

S[12 | 100

a2 |

 

Equating rates of work done and of increase in kinetic energy,

‘To= loa wale (7.43)Eq. (7.43) is similar to Newton's second law for Imear motion expressed symbolically as Fema

 

Just as force produces acceleration, torque produces angular acceleration in a body. The angular acceleration is directly proportional to the applied torque and is inversely proportional

to the moment of inertia of the body. Eq.(7.43)can be called Newton's second law for rotation about a fixed axis.

 

Example 7.15 A cord of negligible mass

is wound round the rim of a fly wheel of

mass 20 kg and radius 20 cm. A steady

pull of 25 N is applied on the cord as shown in Fig. 7.35. The flywheel is mounted on a horizontal axle with frictionless bearings.

(a) Compute the angular acceleration of

the wheel.

(b) Find the work done by the pull, when

2m of the cord is unwound.

(c) Find also the kinetic energy of the

whee] at this point. Assume that the

whee] starts from rest.

(d) Compare answers to parts (b) and (c).

(a) We use Ia@=f

the torque t=FR

=25 X 0.20 Nm (as R=0.20m)

= 5.0 Nm

I=M. 1. of flywheel about its axia SS

20.0x(02) = 0.4 kg m*

@= angular acceleration

= 5.0 N m/0.4 kg m? = 12.5 s*

 

(b) Work done by the pull unwinding 2m of the cord

=25NX2m=50J

 

(c) Let @ be the final angular velocity. The 1 2 kinetic energy gained = 3 @.since the wheel starts from rest. Now,of =@ +200, a,=0 The angular displacement 6 = length of unwound string / radius of wheel = 2m/0.2 m = 10 rad @ =2x12.5x10.0 = 250(rad/s)?. KE. gained = = x 0.4 x 250 = 50J (d) The answers are the same, Le. the kinetic energy gained by the wheel = work done by the force. There is no loss of energy due to friction.

 

7.13 ANGULAR MOMENTUM IN CASE OF ROTATION ABOUT A FIXED AXIS

We have studied in section 7.7, the angular momentum ofa system of particles. We already know from there that the time rate of total angular momentum of a system of particles about a point is equal to the total external torque on the system taken about the same point.When the total external torque is zero, the total angular momentum of the system is conserved.

We now wish to study the angular momentum in the special case of rotation about a@ fixed axis. The general expression for the total angular momentum of the system is

N

L= 1 Xp, (7.25)

iol

 

We firat consider the angular momentum of a typical particle of the rotating rigid body. We then sum up the contributions of individual particles to get L of the whole body.For a typical particle 1 = r x p. As seen in the last section r = OP = OC + CP [Fig. 7.17(b)].

With p=mv,1=(0C x mv) + (CPx mv)

 

The magnitude of the linear velocity w of the particle at P is given by v = wr, where r, is the length of CP or the perpendicular distance of P from the axis of rotation. Further, v is tangential at P to the circle which the particle describes.Using the right-hand rule one can check that CP x v is parallel to the fixed axis. The unit

vector along the fixed axis (chosen as the 2-axis)is , . Hence CP xmve=r, (mv) k = mrok (since v=ur,)

 

Similarly, we can check that OC x vw is

perpendicular to the fixed axis. Let us denote the part of 1 along the fixed axis {1.e. the z-axis)by L, then

‘L, = CPx mv = mrok and 1=1,+O0Cxmv

 

We note that I, is parallel to the fixed axts,but lis not. In general, for a particle, the angular momentum 1 is not along the axis of rotation,i.e. for a particle, 1 and o are not necessarily

paralle]. Compare this with the corresponding fact in translation. For a particle, p and v are always parallel to each other.

 

For computing the total angular momentum

of the whole rigid body, we add up the

contribution of each particle of the body.Thus L=31=)1,+ 0c, xmvy,We denote by L_ and L, the components of L respectively perpendicular to the z-axis and along the z-axis;L, => 0€, x my, {7.44a)

 

where m, and v, are respectively the mass and the velocity of the f particle and C, is the centre of the circle described by the particle;and L,-D1.-[E mat oR or L, = lok (7.44b)

 

The last step follows since the perpendicular distance of the f particle from the axia 1s 1;and by definttion the moment of inertia of the body about the axis of rotation is [= mr? .

Note L=L, + L_ (7.44c)

 

The rigid bodiea which we have mainly

considered in this chapter are symmetric about the axis of rotation, i.c. the axis of rotation is one of their symmetry axea. For such bodies,for a given OC, for every particle which haa a velocity v, , there is another particle of velocity -¥, located diametrically opposite on the circle with centre C, described by the particle. Together such pairs will contribute zero to L_ and asa

result for symmetric bodies L_ is zero, and hence L=L, = lok (7.44)

 

For bodies, which are not symmetric about the axis of rotation, L is not equal to L, and hence L does not lie along the axis of rotation.

 

Referring to table 7.1, can you tell in which cases L = L, will not apply?

Let us differentiate Eq. (7.44b). Since { isa fixed (constant) vector, we get

d id =

S(t, )-|2(10) i

Now, Eq. (7.28b) states

‘aL -

di

 

As we have seen in the last section, only those components of the external torques which are along the axis of rotation, need to be taken into account, when we discuss rotation about a

fixed axis. This means we can take 1+ = tk.Since L =L, + L, and the direction of L, (vector ‘i¢) 18 fixed, it follows that for rotation about a fixed axia,

‘dL, os

a {7.45a)

‘dL

and “a9 (7.45b)

 

Thus, for rotation about a fixed axis, the component of angular momentum

perpendicular to the fixed axis is constant. As L, = lok , we get from Eq. (7.45a),<(10)=1 (7.450)

 

If the moment of inertia I does not change with time,d, do

di (l@)=I di: la

and we get from Eq. (7.45c),

‘t=la (7.43)

We have already derived this equation using the work - kinetic energy route.

 

 7.13.1 Conservation of angular momentum

We are now in a position to revisit the principle of conservation of angular momentum in the context of rotation about a fixed axis. From Eq.(7.45c), if the external torque is zero,

L, = Jo = constant (7.46)

 

For symmetric bodies, from Eq. (7.44d}, L, may be replaced by L (Land L, are respectively the magnitudes of L and L..)

 

This then is the required form, for fixed axis rotation, of Eq. (7.29a), which expreases the general law of conservation of angular momentum of a system of particles. Eq. (7.46)

applies to many situations that we come across in daily life. You may do this experiment with your friend. Sit on a swivel chair with your arms folded and feet not resting on, f.e., away

from, the ground. Ask your friend to rotate the chair rapidly. While the chair is rotating with considerable angular speed stretch your arms

horizontally. What happens? Your angular

speed is reduced. If you bring back your arms closer to your body, the angular speed increases again. This is a situation where the principle of conservation of angular momentum is

applicable. If friction in the rotational mechanism is neglected, there is no external torque about the axis of rotation of the chair and hence Iais constant. Stretching the arms increases I about the axis of rotation, resulting

in decreasing the angular speed Bringing the arms closer to the body has the opposite effect.

 

Acircus acrobat and a diver take advantage of this principle. Also, skaters and classical,Indian or western, dancers performing a pirouette on the toes of one foot display ‘mastery’

over this principle. Can you explain?

 

7.14 ROLLING MOTION

One of the most common motions observed in daily life is the rolling motion. All wheels used in transportation have rolling motion. For specificness we shall begin with the case of a

disc, but the result will apply to any rolling body rolling on a level surface. We shall assume that the disc rolls without slipping. This means that

at any instant of time the bottom of the disc which is in contact with the surface is at rest on the surface.

 

We have remarked earlier that rolling motion is a combination of rotation and translation.We know that the translational motion of a system of particles is the motion of its centre of mass.

Let v,, be the velocity of the centre of mass and therefore the translational velocity of the

disc. Since the centre of mass of the rolling disc ia at ite geometric centre C (Fig. 7. 37), v,,,18 the velocity of C. It is parallel to the level

surface. The rotational motion of the disc is about its symmetry axis, which passes through Cc. Thus, the velocity of any point of the diac,like P,, P, or P,, consists of two parts, one is the

translational velocity v_ and the other is the linear velocity v.on account of rotation. The magnitude of v_is v_=re, where wis the angular velocity of the rotation of the disc about the axis

and ris the distance of the point from the axis (i.e. from C). The velocity v,is directed perpendicular to the radius vector of the given point with respect to C. In Fig. 7.37, the velocity

of the point P, (v,) and its components v,and v,,are shown; v,here is perpendicular to CP,.It is easy to show that v, is perpendicular to the

line P,P... Therefore the line passing through P,, and parallel to o is called the instantaneous axis of rotation.

 

At P,, the linear velocity, v,, due to rotation is directed exactly opposite to the translational velocity v,,,. Further the magnitude of v,here is Ro, where R is the radius of the disc. The

condition that P,is instantaneously at rest requires v= Res, Thus for the disc the condition for rolling without slipping is 0, = Re (7.47)

 

Incidentally, this means that the velocity of point P, at the top of the disc (v,) has a magnitude v_+ Rw or 2 v_,and is directed parallel to the level surface. The condition (7.47)applies to all rolling bodies.

 

7.14.1 Kinetic Energy of Rolling Motion

Our next task will be to obtain an expression for the kinetic energy of a rolling body. The kinetic energy of a rolling body can be separated

into kinetic energy of translation and kinetic energy of rotation. This is a special case of a general result for a system of particles,according to which the kinetic energy of a system of particles (KX) can be separated into

the kinetic energy of motion of the centre of mnass (translation) (MfV7/2) and kinetic energy of rotational motion about the centre of mass of the system of particles (K). Thus,K=K'+ MV? /2 (7.48)

 

We assume this general result (see Exercise 7.31), and apply it to the case of rolling motion.In our notation, the kinetic energy of the centre of mass, i.e., the kinetic energy of  translation,of the rolling body is mv*_ /2, where m is the mass of the body and v_, is the centre of the mnass velocity. Since the motion of the rolling body about the centre of mass is rotation, K’represents the kinetic energy of rotation of the body; K’=I@/2, where I is the moment of inertia about the appropriate axis, which is the symmetry axis of the rolling body. The kinetic energy of a rolling body, therefore, is given by K =5 lox +5iwi, (7.49a)

 

Substituting I = mk® where k = the

corresponding radius of gyration of the body and v_ = Ra, we get Kak etn yd mei,

2 RF 2

1 sos ke

or K= 2 MUG, ( Py ) (7.49b)

Equation (7.49b) applies to any rolling body:a disc, a cylinder, a ring or a sphere.

 

Example 7.16 Three bodies, a ring, a solid cylinder and a solid sphere roll down the sarge incHned plane without sipping. They start from rest. The radii of the bodies are identical. Which of the bodies reaches the ground with maxbuum velocity?

Answer We assume conservation of energy of the rolling body, Le. there is no loss of energy due to friction etc. The potential energy lost by the body in rolling down the inclined plane

(= mgh) must, therefore, be equal to kinetic energy gained. (See Fig.7.38) Since the bodies start from rest the kinetic energy gained is equal to the final kinetic energy of the bodies. From

1 2 ke Eq, (7.49), Kap mv (+f). where v is the final velocity of (the centre of mass of the body.Equating K and mgh,

mgh <1 ny? Ga

2 R

3 2gh

vy =| —

or (re |

Note is independent of the mass of the

rolling body;

For a ring, k° =

Dany = 2ah

. {ist .

= Jon

For a solid cylinder kK? = F°/2

_ | 2gh

Pew | 141/2

_ 4gh

7 { 3

For a solid sphere i* = 2R°/5

_ 2gh

Dophere com 14+2/5

_ 10gh

7 1 7

 

From the results obtained itis clear that among the three bodies the sphere has the greatest and the ring has the least velocity of the centre of mass

at the bottom of the inclined plane.

Suppose the bodies have the same mass. Which body has the greatest rotational Ininetic enexgy while reaching the bottem of the inclined plane? 4

 

SUMMARY

1. Ideally, a rigid body is one for which the distances between different particles of the body do not change, even though there are forces on them.

 

2. Arigid body fixed at one point or along a line can have only rotational motion. A rigid body not fixed in some way can have efther pure translation or a combination of translation and rotation.

 

3. In rotation about a fixed axis, every particle of the rigid body moves in a circle which Hes in a plane  perpendicular to the axis and has ite centre on the axis. Every Point in

the rotating rigid body has the same angular velocity at any instant of time.

 

4. In pure translation, every particle of the body moves with the same velocity at any instant of time.

 

5. Angular velocity is a vector. Ite magnitude ie @ = d0/dt and it fa directed along the axle of rotation. For rotation about a fixed axis, this vector has a fixed direction.

 

6. The vector or cross product of two vector a and b is a vector written as a x b. The magnitude of this vector ie absind and ite direction is given by the right handed screw or the right hand rule.

 

7. The linear velocity of a particle of a rigid body rotating about a fixed axis is given by v=o fF, where £ is the position vector of the particle with respect to an origin along the fixed axis. The relation applies even to more general rotation of a rigid body with one point fixed. In that case r is the position vector of the particle with respect to the fixed point taken as the origin.

 

8. The centre of mase of a system of particles is defined as the point whose position vector is

 

 

9. Velocity of the centre of mass of a ayatem of particles is given by V= P/M, where F is the linear momentum of the system. The centre of mass moves as if all the mass of the system is concentrated at this point and all the external forces act at it. If the total

external force on the system is zero, then the total linear momentum of the system is constant.

 

10. The angular momentum of a system of n particles about the origin is

L = ¥nxp,

t2l

The torque or moment of force on a system of n particles about the origin is

t=) 1xF,

1

 

The force F, acting on the ¢@ particle includes the external as well as internal forces.Assuming Newton's third law and that forces between any two particles act along the line joining the particles, we can show 1,, = 0 and

a

 

11. A rigid body is in mechanical equilibrium if

(1) itis in tranalational equilibrium, Le., the total external force on it is zero : SF=0,and

 

(2) it ie in rotational equilibrium, 1.e. the total external torque on it ia zero :¥a= ¥nxk=0.

 

12. The centre of gravity of an extended body is that point where the total gravitational torque on the body is zero.

 

13. The moment of intertia of a rigid body about an axis is defined by the formula lem where 7, is the  perpendicular distance of the th point of the body from the axis. The ol ye

kinetic energy of rotation ie Kes le,

 

14. The theorem of parallel axes: ‘el, +Ma?, allows us to determine the moment of intertia of a rigid body about an axis as the sum of the moment of inertia of the body about a parallel axis through its centre of masse and the product of masse and square of the perpendicular distance between these two axes.

 

15. Rotation about a fixed axis is directly analogous to linear motion in respect of kinematics and dynamics.

 

16. For a rigid bedy rotating about a fixed axis (say, z-axis) of rotation, L, = Im, where lis the moment of inertia about z-axis. In general, the angular momentum L for such a body is not along the axis of rotation. Only if the bedy is symmetric about the axis of rotation, L is along the axis of rotation. In that case, |L|= L, = Iw. The angular

acceleration of a rigid body rotating about a fixed axia ia gtven by Ig = +. If the external torque 7 acting on the body is zero, the component of angular momentum about the fixed axis (say, 2-axia), L, (=a) of such a rotating body is constant.

 

17. For rolling motion without alipping v_, = Re, where v_, is the velocity of translation (1.e.of the centre of maas), Ris the radtus and mia the mass of the body. The kinetic energy of such a rolling body is the aum of kinetic energies of translation and rotation:

K=im 2, +102,

2 2

 

POINTS TO PONDER

1. To determine the motion of the centre of masa of a system no knowledge of internal forces of the system ig required. For thia purpose we need to know only the external forcea on the body.

 

2. Separating the motion of a system of particles as the motion of the centre of mass, f1.c.,the tranalational motion of the aystem) and motion about {i.e relative to) the centre of masa of the system ja a useful technique m dynamics of a system of particles. One example of thia technique is separating the kinetic energy of a system of particles K as

the kinetic energy of the system about its centre of mass K’ and the kinetic energy of the centre of mass MV?/2,

K= K’+ MV7/2

 

3. Newton's Second Law for finite alized bodies (or syatems of particles} ia based in Newton's Second Law and also Newton's Third Law for particles.

 

4. To establish that the time rate of change of the total angular momentum of a system of particles is the tota) external torque in the syatem, we need not only Newton's second law for particles, but also Newton's third law with the proviaton that the forcea between.any two particlea act along the Hne joining the particles.

 

5. The vanishing of the total external force and the vanishing of the total external torque are independent conditiona. We can have one without the other. In a couple, total external force is zero, but total torque is non-zero.

 

6. The total torque on a syatem is Mdependent of the origin tf the total external force is zero.

 

7. The centre of gravity of a body coincides with its centre of maas only if the gravitational field does not vary from one part of the body to the other.

 

8. The angular momentum L and the angular velocity are not necessarily parallel vectors.However, for the simpler situations discussed in this chapter when rotation ia about a

fixed axis which is an axis of aymmetry of the rigid body, the relation L = ia holds good,where I is the moment of the inertia of the body about the rotation axis.

 

EXERCISES

7.1 Gave the location of the centre of masse of a {i) sphere, (ii) cylinder, (iii) ring, and {iv)cube, cach of uniform mass density. Does the centre of mass of a body necessarily lie inside the bedy ?

7.2 In the HC1 molecule, the separation between the nucle of the two atoms is about

 

1.27 A(1 A= 10-10 ny. Find the approximate location of the CM of the molecule,given that a chlorine atom is about 35.5 times as massive as a hydrogen atom and nearly all the mass of an atom is concentrated in its nucleus.

 

7.3 Achild alte stationary at one end of a long trolley moving uniformly with a speed V on a emooth horizontal floor. If the child gete up and rune about on the trolley in any manner, what ia the speed of the CM of the (trolley + child) system ?

 

7.4 Show that the area of the triangle contained between the vectors a and b is one half of the magnitude of a x b.

 

7.6 Show that a-(b x c) is equal in magnitude to the volume of the parallelepiped formed on the three vectors , a, b and c.

 

7.6 Find the components along the x, y, z axca of the angular momentum IJ of a particle,whose position vector is r with components x, y, z and momentum ia p with components p,, p, and p,, Show that if the particle moves only in the x-y plane the angular momentum has only a z-component.

 

7.7 Two particles, cach of mass mand speed v, travel in opposite directions along parallel lines separated by a distance d. Show that the vector angular momentum of the two particle system is the same whatever be the point about which the angular momentum ie taken.

 

7.8A non-uniform bar of weight W is suspended at rest by two strings of negligible weight as shown in Fig.7.390. The angles made by the strings with the vertical are 36.9° and 53.1°respectively. The bar is 2 m long. Calculate the distance d of the

centre of gravity of the bar from ite left end.

 

7.9 Acar weighs 1800 kg. The distance between its front and back axics is 1.8 m. Its centre of gravity is 1.05 m behind the front axle. Determine the force exerted by the level ground on each front wheel and each back wheel.

 

7.10 Find the moment of inertia of a sphere about a tangent to the sphere, given the moment of inertia of the sphere about any of its diameters to be 2MR®°/5, where M is the masse of the sphere and R is the radius of the sphere.

 

(b) Given the moment of inertia of a disc of mass M and radius R about any of its diameters to be MF°/4, find its moment of inertia about an axis normal to the disc and passing through a point on ite edge.

 

7.11 Torques of equal magnitude are applied to a hollow cylinder and a solid sphere, both having the same mass and radtus. The cylinder is free to rotate about its standard axie of symmetry, and the sphere is free to rotate about an axis passing through its centre. Which of the two will acquire a greater angular speed after a given time.

 

7.12 Aaolid cylinder of mass 20 kg rotates about its axis with angular speed 100 rad "1.The radiue of the cylinder is 0.25 m. What ia the kinetic energy associated with the rotation of the cylinder? What fa the magnitude of angular momentum of the cylinder

about ita axis?

 

7.13 (a) A child stands at the centre of a turntable with his two arms outstretched. The turntable ts set rotating with an angular speed of 40 rev/min. How much is the angular speed of the child if he folds his hands back and thereby reduces his moment of inertia to 2/5 times the initial value ? Assume that the turntable rotates without friction.

 

(b) Show that the child’s new kinetic energy of rotation is more than the mitial Kinetic energy of rotation. How do you account for this increase in kinetic energy?

 

7.14 Arope of negligible mass is wound round a hollow cylinder of mass 3 kg and radius 40 cn. What ia the angular acceleration of the cylinder if the rope ie pulled with a force of SO N ? What is the linear acceleration of the rope ? Assume that there ia no slipping.

 

7.18 To maintain a rotor at 2 uniform angular speed of 200 rad s”!, an engine needs to transmit a torque of 180 N m. What is the power required by the engine ?

(Note: uniform angular velocity in the absence of friction implies zero torque. In practice, applied torque is needed to counter frictional torque). Assume that the engine is 100% efficient.

 

7.18 From a uniform disk of radius R, a circular hole of radius R/2 is cut out. The centre of the hole is at R/2 from the centre of the original disc. Locate the centre of gravity of the resulting fat body.

 

7.17 Armetre stick is balanced on a knife edge at ita centre. When two coins, each of mass 5 g are put one on top of the other at the 12.0 cm mark, the stick is found to be balanced at 45.0 cm. What is the mass of the metre stick?

 

7.18 A solid sphere rolls down two different inclined planes of the same heights but different angles of inclination. (a) Will it reach the bottom with the same speed in each case? (b) Will it take longer to roll down one plane than the other? (c) If so,

which one and why?

 

7.18 Ahoop of radius 2 m weighe 100 kg. It rolle along a horizontal floor so that its centre of mass has a speed of 20 cmn/e. How much work has to be done to atop it?

 

7.20 The oxygen molecule has a mass of 5.30 x 10” kg and a moment of inertia of

1.94x10 kg m? about an axis through ite centre perpendicular to the lines joining the two atoms. Suppose the mean speed of such a molecule in a gas is 500 m/s and that its kinetic energy of rotation is two thirds of its kinetic energy of translation.Find the average angular velocity of the molecule.

 

7.21 A solid cylinder rolls up an inclined plane of angle of inclination 30°. At the bottom of the inclined plane the centre of mass of the cylinder has a speed of 5 m/s.

 

(a) How far will the cylinder go up the plane?

(b) How long will it take to return to the bottom?

 

Additional Exercises

7.22 As ehown in Fig.7.40, the two sides of a step ladder BA and CA are 1.6 m long and hinged at A. Arope DE, 0.5 m is ted half way up. A weight 40 kg is suspended from a point F, 1.2 m from B along the ladder BA. Assuming the floor to be frictionless and neglecting the weight of the ladder. find the tension in the rope and forces exerted by the floor on the ladder. (Take g = 9.8 m/s4)

(Hint: Consider the equilibrium of each side of the ladder separately.)

 

7.23 A man stands on a rotating platform, with his arme stretched horizontally holding a 5 kg weight in each hand. The angular speed of the platform is 30 revolutions per minute. The man then brings his arms close to his body with the distance of each weight from the axis changing from 90cm to 20cm. The moment of inertia of the man together with the platform may be taken to be constant and equal to 7.6 kg m*.

(a} What ie his new angular speed? (Neglect friction.)

 

(b) Ie kinetic energy conserved in the process? If not, from where does the change come about?

 

7.24 A bullet of masse 10 g and speed 500 m/s is fired into a door and gets embedded exactly at the centre of the door. The door is 1.0 m wide and weighs 12 kg. It is hinged at one end and rotates about a vertical axis  practically without friction. Find

the angular speed of the door just after the bullet ambeds inte it.

(Hint: The moment of inertia of the door about the vertical axis at one end is ML?/3.}

 

7.25 Two disce of moments of inertia J) and Ip about their respective axes (normal to the diec and passing through the centre), and rotating with angular speeds a) and «2 are brought into contact face to face with their axes of rotation coincident. (a) What

is the angular speed of the two-disc system? (b) Show that the kinetic energy of the combined system is less than the sum of the initial kinetic energies of the two discs.How do you account for this loss in energy? Take 0] # @32.

 

7.26 (a} Prove the theorem of perpendicular axes.

(Hint : Square of the distance of a point (x, y) in the x-y plane from an axis through the origin and perpendicular to the plane is 17+y3.

 

(b) Prove the theorem of parallel axes.

(Hint : If the centre of masa is chosen to be the origin ) 1,1, = 0),

 

7.27 Prove the result that the velocity vu of translation of a rolling body {like a ring, disc,cylinder or sphere) at the bottom of an inclined plane of a height h is given by 2 2gh w= oF

(14k? /R*)using dynamical consideration (i.c. by consideration of forces and torques). Note kis the radius of gyration of the body about ite symmetry axis, and R is the radius of the body. The body starts from rest at the top of the plane.

 

7.28 A disc rotating about ite axis with angular speed @p is placed lightly (without any tranalational push) on a perfectly frictionless table. The radius of the disc is R. What are the lincar velocities of the pointa A, B and C on the disc shown in Fig. 7.41? Will the disc roll in the direction indicated ?

 

7.29 Explain why friction is necessary to make the disc in Fig. 7.41 roll in the direction indicated.

 

(a) Give the direction of frictional force at B, and the sense of frictional torque, before perfect rolling

begins.

 

(b) What is the force of friction after perfect rolling begins ?

 

7.30 Asolid disc and a ring, both of radius 10 cm are placed on a horizontal table simultanecualy, with mitial angular speed equal to 10 1 rad 6-1. Which of the two will start to roll

earlier ? The co-efficient of kinetic friction ia as 0.2.

 

7.31 Acylinder of mass 10 kg and radius 15 om ia rolling perfectly on a plane of inclmation 30°. The co-efficient of static friction fj, = 0.25.

(a) How much is the force of friction acting on the cylinder ?

 

(b) What is the work done against friction during rolling ?

 

(c) If the inclination of the plane is increased, at what value of does the cylinder begin to skid,and not roll perfectly ?

 

7.32 Read each statement below carefully, and state, with reasons, ff ft is true or false;

(a) Durmg rolling, the force of friction acts in the same direction as the direction of motion of the CM of the body.

 

(b) The instantaneous speed of the point of contact during rolling is zero.

 

(c) The instantaneous acceleration of the point of contact during rolling is zero.

 

(d) For perfect rolling motion, work done against friction is zero.'

 

(e) A wheel moving down a perfectly frictionless inclmed plane will undergo slipping (not rolling)motion.

 

7.33 Separation of Motion of a system of particles into motion of the centre of mass and motion about the centre of mass :( Show p=p’ +m,V where p,is the momentum of the ith particle (of mass m) and p’,= m,v’, Note v’, is the velocity of the ith particle relative to the centre of mass.Also, prove using the definition of the centre of mass > p =0

(b) Show K = K’+'42MV" where K is the total kinetic energy of the syatem of particles, ;<‘ is the total kinetic energy of the system when the particle velocities are taken with respect to the centre of mass and MV*/2 is the kinetic energy of the translation of the system as a whole {i.c. of the centre of masse motion of the system). The result has been used in Sec. 7.14.

 

(c) Show L=L’+ Rx MV

where [’= ) 1’ X pi is the angular momentum of the system about the centre of mase with velocitics taken relative to the centre of mass. Remember r’=r, -R; reat of the notation is the standard notation used in the chapter. Note |’ and MRxV can be said to be angular momenta, respectively, about and of the centre of mass of the system of

particles.

 

(a) Show a te Further, show that

a’ a Vert where 7, is the sum of all external torques acting on the system about the centre of mase.(Hint : Use the definition of centre of mass and Newton's Third Law. Assume the internal forces between any two particles act along the line joining the particles.)

Pluto - A Dwarf Planet

 

The International Astronomical Union ([AU) at the IAU 2006 General Assembly

held on August 24, 2006, in Prague in Czech Republic, adopted a new definition of planets in our Solar System.According to the new definition,Pluto is no longer a planet. This means that the Solar System consists of eight planets: Mercury, Venus, Earth, Mars, Jupiter, Saturn, Uranus and Neptune. According to the [AU usage, the ‘planet’ and ‘other bodies’ in our Solar System, except satellites, are to be defined into three distinct  categories of celestial objects in the following way:

 

1. A ‘planet’ is a celestial body that {a) is in orbit around the Sun, (b) has

sufficient mass for its self- gravity to overcome rigid body forces so that it

assumes a hydrostatic equilibrium (nearly round) shape, and (c) has

cleared the neighbourhood around its orbit.

 

2. A ‘dwarf planet’ is a celestial body that (a) is in orbit around the Sun,

(b) has sufficient mass for its self- gravity to overcome rigid body forces so

that it assumes a hydrostatic equilibrium (nearly round) shape, (c} has not cleared the neighbourhood around its orbit, and {d) is not a satellite.

 

3. All ‘other objects’, except satellites, orbiting the Sun, shall be referred to collectively as ‘Small Solar-System Bodies’.

 

Unlike other eight planets in the Solar System, Pluto's orbital path overlaps

with ‘other objects’ and the planet Neptune. The ‘other objects’ currently

include most of the Solar System asteroids, most of the Trans-Neptunian

Objects (TNOs), comets, and other small bodies.Pluto is a ‘dwarf planet’ by the above definition and is recognised as the prototype of a new category of Trans-Neptunian Objects.