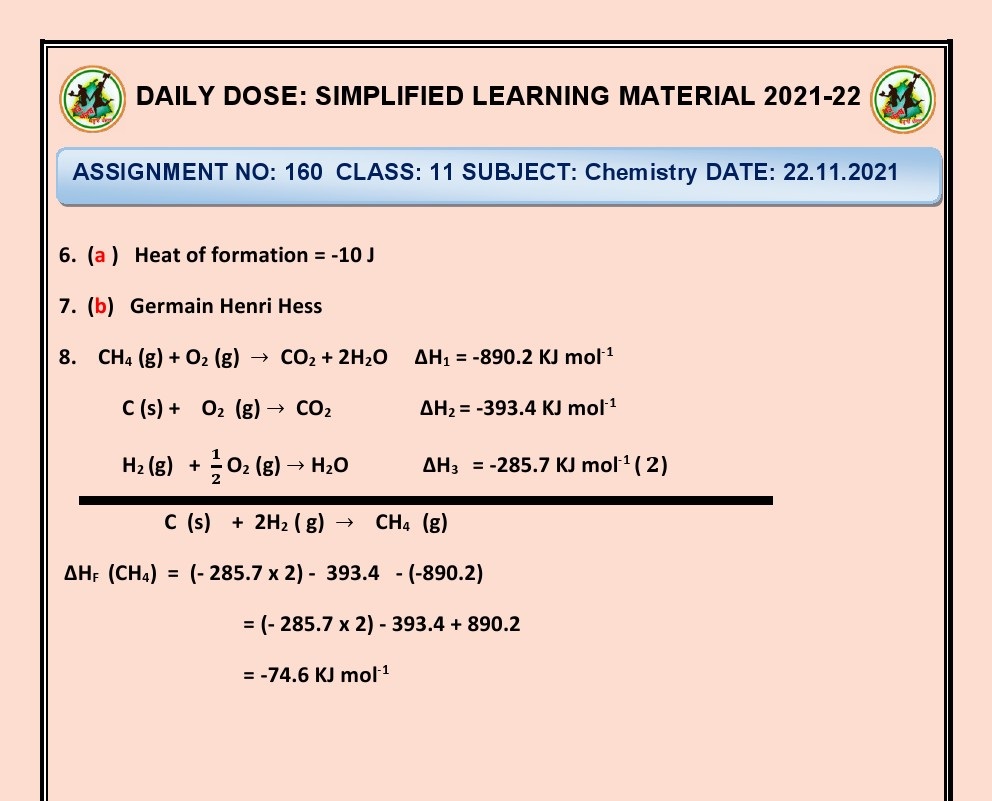
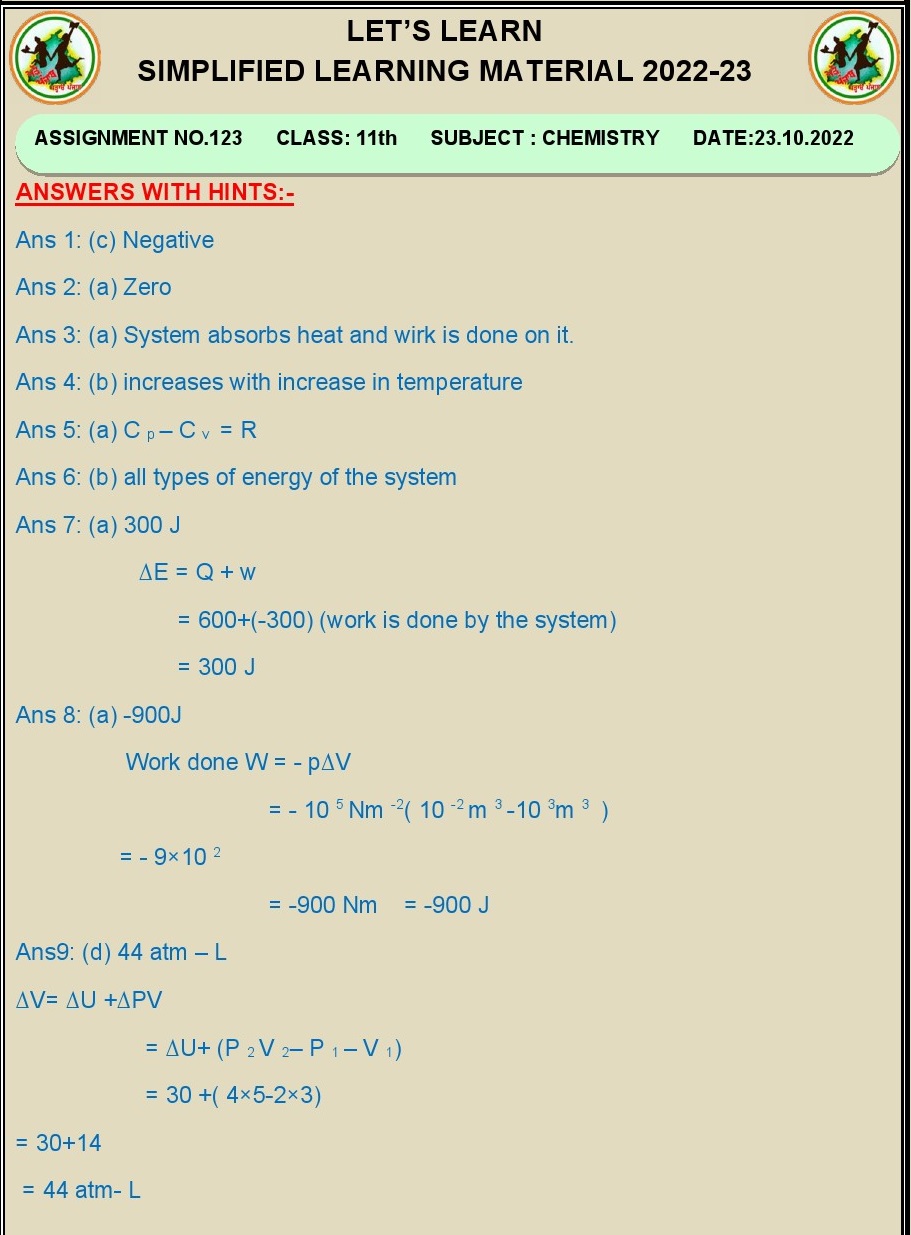
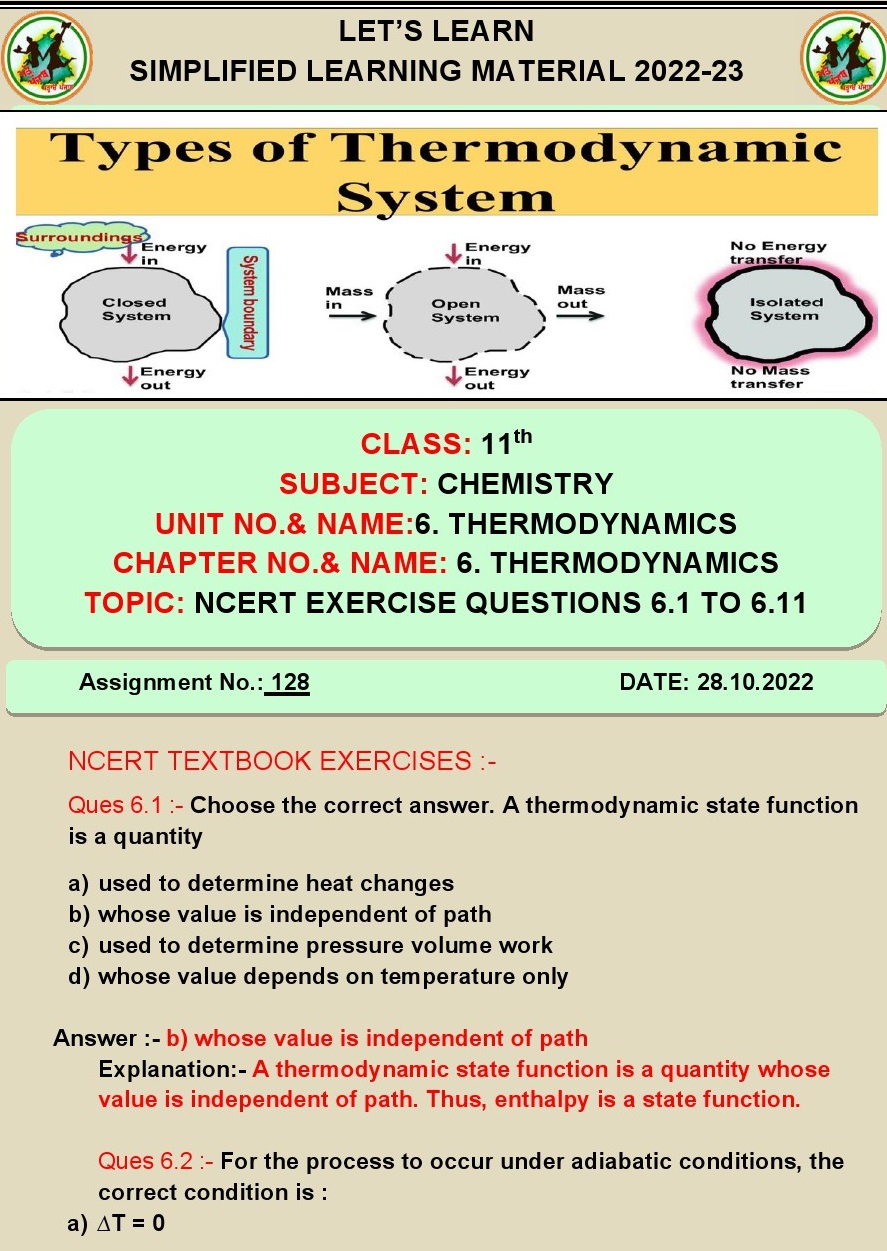
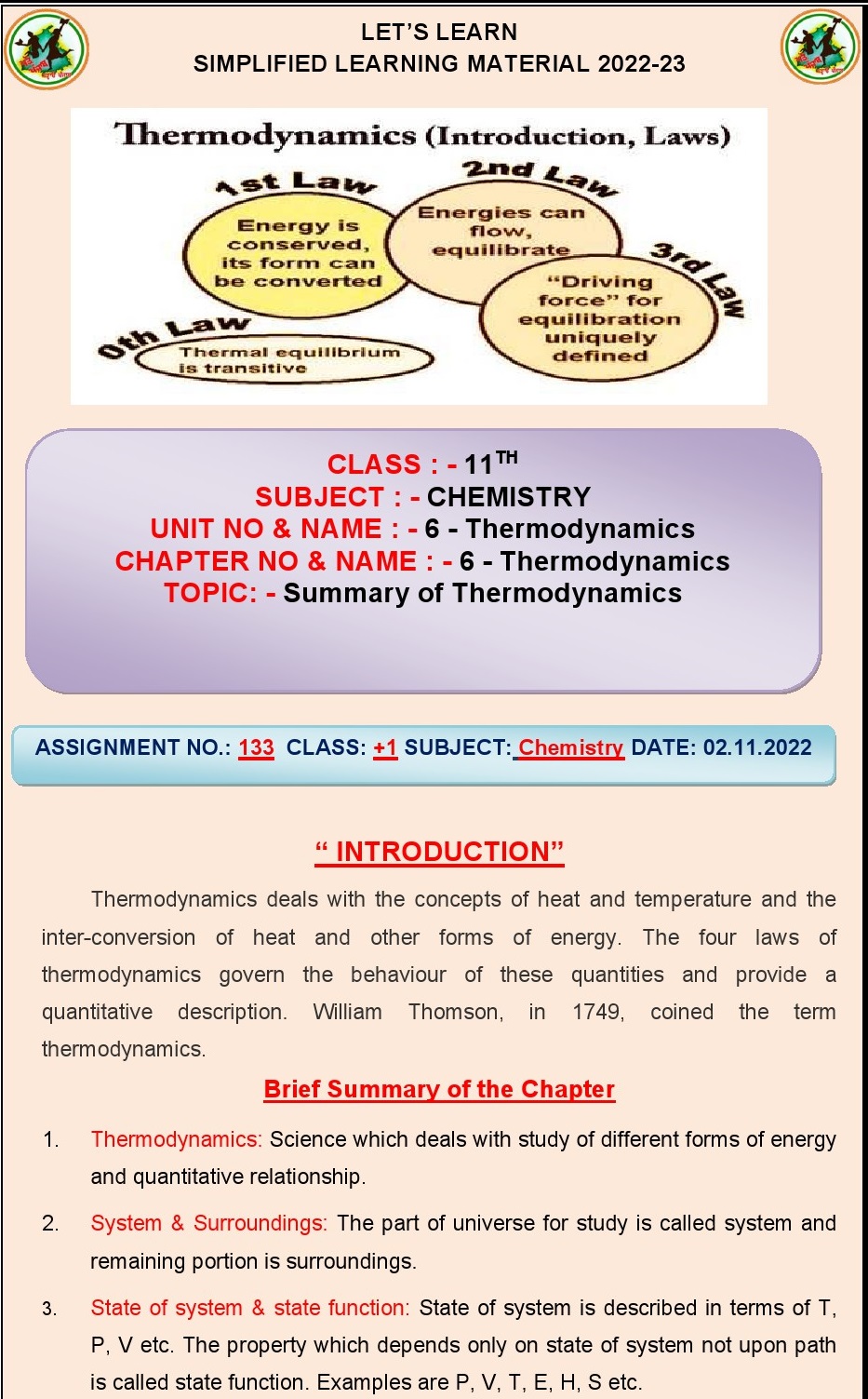
6- THERMODYNAMICS
THERMODYNAMLCS
VERY SHORT QUESTIONS
ANSWER
Q.1.Distinguish between a system a
surroundings?
Ans. System: The specific part of interest under study.
Surroundings:
Everything outside the system that can
interact with it.
Q.2.What do you understand by the ‘
state of system?
Ans. The specific condition of a system described by its
properties like temperature, pressure, volume, and composition.
Q.3.What are state functions or state
of a system?
Ans. Properties of a system that depend only on its current
state and not on the path taken to reach that state.
Q.4.Define intensive property?
Ans. Properties of a system that depend only on its current
state and not on the path taken to reach that state.
Q.5. Define extensive property?
Ans. Extensive property: A property of a system that depends
on the size or amount of the system.
Q.6. Define isolated system and give
one example?
Ans. Isolated system: A system that does not exchange energy or matter with its
surroundings.
Example: A thermally insulated flask containing a reaction taking
place inside without any heat or matter exchange with the surroundings.
Q.7. Differentiate between open and
closed systems?
Ans. Open system: Can exchange both energy and matter with its
surroundings.
Closed
system: Can exchange only
energy but not matter with its surroundings.
Q.8. Define a thermodynamically
reversible process?
Ans. Thermodynamically reversible process: A process that
occurs infinitely slowly and with no entropy change.
Q.9. Can the reversible process be
realized in practice?
Ans. No, reversible processes are idealized and cannot be
realized in practice due to the absence of infinite slowness and perfect
conditions.
Q.10. How is a reversible process be
realized in practice?
Ans. A truly reversible process cannot be realized in
practice, but certain processes can be made close to reversible by carrying
them out very slowly to minimize entropy changes.
Q.11.Is spontaneous process reversible
in nature?
Ans. No.
Q.12. Give one difference between an
isothermal and an adiabatic process?
Ans. Isothermal process: Occurs at constant temperature, leading to no change in
internal energy.
Adiabatic
process: Occurs with no heat
exchange with the surroundings, leading to a change in internal energy.
Q.13.Give the physical significance of
enthalpy?
Ans. Enthalpy represents the heat flow at constant pressure
during a process.
Q.14.State the first law of
thermodynamics?
Ans. The first law of thermodynamics states that energy cannot
be created or destroyed, only converted from one form to another.
Q.15.Give the main limitation of first
law of thermodynamics?
Ans. The first law of thermodynamics does not provide
information about the direction of a process (i.e., it does not predict whether
a process will occur spontaneously or not).
Q.16.Give the main limitation of first
law of thermodynamics?
Ans. The first
law of thermodynamics does not indicate the direction of processes.
Q.17.Name the law which says that it is
impossible to construct a perpetual motion meachine?
Ans. The law is called the "second law of
thermodynamics."
Q.18.Can absolute value of H be
determined?
Ans. No, the absolute value of enthalpy (H) cannot be
determined, only changes in enthalpy (ΔH) can be measured.
Q.19.Define heat capacity?
Ans. Amount of heat required to raise the temperature of a
substance by one degree Celsius or Kelvin.
Q.20.What
is the other name for zeroth law of thermodynamics?
Ans. Thermal equilibrium law.
Q.21.Can thermodynamics predict the feasibility
of a process?
Ans. No, thermodynamics cannot predict the feasibility of a
process; it only determines whether energy conservation is satisfied.
Q.22.Can thermodynamics tell about some
system which is not in equilibrium?
Ans. Yes, thermodynamics can analyze systems that are not in
equilibrium through the study of non-equilibrium thermodynamics.
Q.23.Can thermodynamics predict the
feasibility of a process?
Ans. Yes.
Q.24.Heat and path are path functions
is it correct?
Ans. No, heat is a path function, but path is not a
thermodynamic property; it refers to the specific route taken during a process.
Q.25.Define zeroth law of
thermodynamics?
Ans. If two systems are each in thermal equilibrium with a
third system, then they are in thermal equilibrium with each other.
Q.26.Internal energy is a state
function how?
Ans. Internal energy is a state function because it depends
only on the current state of the system and not on the path taken to reach that
state.
Q.27.How is enthalpy change related to
internal energy change?
Ans. Enthalpy change (ΔH) is related to internal energy change
(ΔU) by the equation ΔH = ΔU + PΔV, where P is the pressure and ΔV is the
change in volume.
Q.28.Define the standard state of a
substance?
Ans. Standard state of a substance is its most stable form at
a defined pressure, temperature (usually 25°C or 298 K), and concentration
(usually 1 mol/L for solutions).
Q.29.Define enthalpy of a system?
Ans. Enthalpy of a system is the total heat energy it contains
at constant pressure.
Q.30.Define standard enthalpy of
formation?
Ans. Standard enthalpy of formation is the enthalpy change
when one mole of a compound is formed from its constituent elements in their standard
states at a specified temperature and pressure (usually 25°C and 1 atm).
Q.31.Which is the standard form of
carbon?
Ans. The standard form of carbon is graphite.
Q.32.What is calorific value?
Ans. Calorific value is the amount of heat energy released per
unit mass of a substance when it undergoes complete combustion.
Q.33.Stae Hess’s law of constant heat
summation?
Ans. Hess's law states that the total enthalpy change of a
reaction is independent of the pathway between the initial and final states.
Q.34.Define enthalpy of reaction?
Ans. Enthalpy of reaction is the heat energy exchange (heat
absorbed or released) during a chemical reaction at constant pressure.
Q.35.Define bond energy is bond
breaking exothermic or endothermic?
Ans. Bond energy is the energy required to break a chemical
bond, and bond breaking is an endothermic process because it requires energy
input.
Q.36.What is entropy?
Ans. Entropy is a measure of the degree of randomness or
disorder in a system.
Q.37.What is the effect of temperature
on entropy?
Ans. As temperature increases, the entropy of a system
generally increases, as there is a greater dispersion of energy and more
microstates available to the particles.
Q.38.Is entropy of the universe
constant?
Ans. No, the entropy of the universe tends to increase over
time due to the second law of thermodynamics.
Q.39.The entropy of a solution is
higher than that of pyre liquid why?
Ans. The entropy of a solution is higher than that of a pure
liquid due to the increased disorder and randomness of the solute particles
dispersed in the solvent.
Q.40.What is the importance of free
energy concept for chemical reactions?
Ans. The concept of free energy helps predict whether a
chemical reaction is spontaneous (favorable) or non-spontaneous (unfavorable)
and provides insights into its feasibility and direction.
SHORT QUESTIONS ANSWER
Q.1.Define free energy of a system?
Ans. The free
energy of a system, denoted by G, is the energy available to do useful work and
is a combination of the system's internal energy (U) and the product of its
absolute temperature (T) and entropy (S). It is given by the equation G = U -
TS.
Q.2.Define internal energy of a system?
Ans. The internal energy of a system is the total energy
contained within the system, including the kinetic energy of its particles and
the potential energy arising from their interactions. It is a fundamental
property used to describe the system's thermodynamic state.
Q.3.Define closed and isolated system
with examples?
Ans. Closed System: A closed system allows the exchange of
energy with its surroundings (in the form of heat and work), but it does not
allow the transfer of matter across its boundaries. The total mass of the
closed system remains constant.
Example: A sealed container of gas. Heat can flow in and out, but
the gas particles cannot escape or enter the container.
Isolated
System: An isolated system
does not allow the exchange of energy or matter with its surroundings. It is
entirely self-contained, and the total energy and mass within the system remain
constant.
Example: A well-insulated thermos flask containing hot coffee. The
heat stays trapped within the flask, and no matter (coffee or heat) can enter
or leave the system.
Q.4.What are homogeneous and
heterogeneous systems?
Ans. Homogeneous System: A homogeneous system is a system that has uniform
composition and properties throughout its entire volume. In other words, all
the components are uniformly mixed at a molecular level, and there are no
visible boundaries or phases within the system.
Example: A solution of salt dissolved in water is a homogeneous
system because the salt molecules are evenly distributed throughout the water,
and there are no visible separate phases.
Heterogeneous
System: A heterogeneous
system is a system that has non-uniform composition and properties, with
distinct regions or phases that can be distinguished from each other.
Example: A mixture of oil and water is a heterogeneous system
because the oil forms separate droplets within the water, creating visible boundaries
between the two phases.
Q.5.What is thermodynamic equilibrium?
Ans. Thermodynamic equilibrium is a state in which a system's
macroscopic properties, such as temperature, pressure, and composition, remain
constant over time, and there is no net exchange of heat, work, or matter
between the system and its surroundings. In this state, the system is in
perfect balance and has reached its most stable configuration.
Q.6.Define Adiabatic and Isochoric
processes?
Ans. Adiabatic Process: An adiabatic process is a thermodynamic process in which
there is no heat exchange between the system and its surroundings. In other
words, the system is thermally insulated, and no heat is added or removed
during the process. As a result, the change in the system's internal energy is
solely due to work done on or by the system.
Example: When a gas expands or compresses rapidly in a perfectly
insulated container, the process is considered adiabatic as there is no heat
transfer.
Isochoric
Process: An isochoric process,
also known as an isovolumetric process, is a thermodynamic process that occurs
at constant volume. In an isochoric process, the system does not perform any
work on its surroundings or vice versa, since the volume remains constant
throughout the process. Any change in the system's internal energy is solely
due to heat exchange.
Example: Heating a gas in a rigid container without allowing it to
expand or contract results in an isochoric process because the volume remains
constant.
Q.7.Define isobaric process?
Ans. An isobaric process is a thermodynamic process in which
the pressure of a system remains constant while other variables, such as volume
and temperature, may change. The term "isobaric" is derived from two
Greek words: "iso" meaning "equal" and "baros"
meaning "pressure."
During an isobaric process,
the system exchanges energy with its surroundings in the form of heat, allowing
for changes in volume and temperature while keeping the pressure constant. In
other words, the system experiences pressure equilibrium with its surroundings
throughout the process.
An example of an isobaric
process is heating a gas confined in a container with a movable piston. As heat
is added, the gas molecules gain kinetic energy, leading to an increase in
temperature and expansion of the gas, pushing the piston outward. The pressure
remains constant during this expansion because the heat input compensates for
the increase in volume, maintaining the pressure at a constant value.
Similarly, during cooling, the gas contracts, but the pressure remains
unchanged because the heat is removed to balance the reduction in volume.
In an ideal isobaric
process, the relationship between the variables can be described by the
following equation:
P * V = constant,
where P is the constant
pressure, V is the volume, and the product of pressure and volume remains
constant throughout the process.
Q.8.What do you understand by state and
path functions?
Ans. In thermodynamics, state functions and path functions are
two fundamental concepts used to describe the properties and behavior of a
system.
State functions:
State functions, also known
as state variables or state properties, are thermodynamic properties that
depend only on the current state of the system and are independent of the path
taken to reach that state. In other words, their values are determined solely
by the initial and final states of the system, not by the process or the
intermediate steps used to get from one state to another. The actual path or
history of the system doesn't matter; the state function's value remains the
same as long as the initial and final states are the same.
Examples of state functions include:
Temperature
(T): The temperature of a
system is solely determined by its current state and is independent of how the
system arrived at that temperature.
Pressure
(P): The pressure of a system
depends only on the current state and the molecular interactions within the
system, not on how the pressure was changed.
The change in a state
function between two states can be represented as ΔX, where X is the state
function.
Path functions:
Path functions, also known
as process functions, are thermodynamic properties that depend on the specific
path taken during a process and not just on the initial and final states of the
system. The actual route or sequence of changes from one state to another
significantly affects the value of the path function.
Examples of path
functions include:
Work
(W): The work done on or
by the system during a process is a path function because it depends on how the
work was performed and the specific changes in the system's state during the
process.
Heat
(Q): The heat transferred
to or from the system during a process is also a path function because the
amount of heat exchanged depends on the specific process and the temperature
variations at each step.
In summary, state functions
depend only on the initial and final states of the system, whereas path
functions depend on the path taken between those states. Understanding the
distinction between these two types of functions is essential in analyzing and
understanding thermodynamic processes and their associated properties.
Q.9.What are thermochemical equations?
Ans. Thermochemical equations are balanced chemical equations
that also include information about the heat energy (enthalpy) changes
associated with a chemical reaction. They provide a way to represent the
chemical reaction and the corresponding heat energy exchange (heat flow)
between the reactants and products during the reaction.
Thermochemical
equations are typically written in the following format:
Reactants ⟶ Products ΔH
Where:
Reactants are the substances
present at the beginning of the chemical reaction.
Products are the substances
formed as a result of the chemical reaction.
ΔH represents the change in
enthalpy (heat energy) for the reaction. It can be positive (endothermic, heat
is absorbed) or negative (exothermic, heat is released) and is usually
expressed in units like joules (J) or kilojoules (kJ) per mole of the balanced
reaction.
Here's an example of a
thermochemical equation for the combustion of methane (CH4):
CH4(g) + 2 O2(g) ⟶ CO2(g) + 2 H2O(g) ΔH
= -802 kJ/mol
In this example, methane
(CH4) and oxygen (O2) are the reactants, and carbon dioxide (CO2) and water
(H2O) are the products. The value of ΔH is -802 kJ/mol, indicating that the
reaction is exothermic, and 802 kilojoules of heat are released per mole of
methane reacted.
Thermochemical equations are
essential in understanding the energy changes associated with chemical
reactions and are commonly used in applications related to chemical processes,
engineering, and heat calculations. The enthalpy values provided in
thermochemical equations can be used to determine the heat exchanged in a
reaction under various conditions, such as different temperatures or reactant
quantities.
Q.10.How does an increase in internal
energy of a system manifest itself?
Ans. An increase in the internal energy of a system manifests
itself through changes in the system's thermodynamic properties, such as
temperature, pressure, volume, and the state of matter. Internal energy (U) is
a fundamental concept in thermodynamics that represents the total energy stored
within a system due to the microscopic motion and interactions of its particles
(atoms or molecules).
When the internal energy of
a system increases, it means that energy has been added to the system, either
in the form of heat transfer (Q) or work done (W) on the system, or both.
Here's how an increase in internal energy can manifest itself in different
ways:
Temperature
Increase: One of the most
common manifestations of an increase in internal energy is an increase in the
system's temperature. When energy is added to a substance, its particles gain
kinetic energy, and their average speed increases. This leads to a rise in
temperature, and the substance may change from a solid to a liquid or a liquid
to a gas if the conditions are appropriate.
Pressure
Change: If the volume of the
system is held constant, an increase in internal energy can lead to an increase
in pressure. This is particularly evident in gases, where an increase in
internal energy leads to higher molecular velocities and more frequent
collisions with the container walls, resulting in higher pressure.
Volume
Expansion: If the system's
pressure is constant, an increase in internal energy can cause the system to
expand its volume. For instance, when a gas is heated at constant pressure, its
internal energy increases, and the gas molecules move with greater speed,
leading to an expansion and an increase in volume.
Phase
Changes: In some cases, an
increase in internal energy can cause a substance to undergo a phase change,
such as melting, vaporization, or sublimation. During these phase transitions,
the temperature remains constant, but the internal energy is still increasing
due to energy being used to break intermolecular forces and rearrange the particles
into a different phase.
It's essential to note that
the manifestation of an increase in internal energy depends on the specific
conditions of the system, such as whether the process occurs at constant pressure,
constant volume, or under different constraints. Additionally, the specific
properties and behavior of the substance being considered also play a role in
how internal energy changes are observed.
Q.11.What is enthalpy of
neutralization?
Ans. The enthalpy of neutralization is the heat energy change
associated with the complete neutralization of an acid and a base to form a
salt and water. This chemical process is an exothermic reaction, meaning heat
is released into the surroundings. The enthalpy of neutralization is typically
measured under constant pressure conditions, such as at atmospheric pressure.
The general chemical
equation for the neutralization of a strong acid (HA) with a strong base (BOH)
is:
HA (aq) + BOH (aq) ⟶ B(A) (aq) + H2O (l)
Where:
HA is the strong acid,
BOH is the strong base,
B(A) represents the salt
formed, and
H2O is water.
During the neutralization
process, hydrogen ions (H+) from the acid react with hydroxide ions (OH-) from
the base to form water. The remaining ions combine to form a salt. The overall
reaction releases energy in the form of heat, which is the enthalpy of neutralization.
The enthalpy of
neutralization is typically expressed in units of joules per mole (J/mol) or
kilojoules per mole (kJ/mol) of the acid or base reacting. The value of the
enthalpy of neutralization is usually negative, indicating that heat is
released during the reaction. The specific value of the enthalpy of
neutralization depends on the specific acid and base involved in the reaction.
For example, the neutralization of hydrochloric acid
(HCl) with sodium hydroxide (NaOH) can be represented by the equation:
HCl (aq) + NaOH (aq) ⟶ NaCl (aq) + H2O (l)
The enthalpy change for this
reaction is typically around -57 kJ/mol, meaning that 57 kilojoules of heat are
released for every mole of HCl neutralized by NaOH. However, it's essential to
note that the exact enthalpy values can vary depending on the specific
experimental conditions and concentrations of the reactants.
Q.12.What is most important use of Hess’s
law?
Ans. The most important use of Hess's law in thermodynamics is
to calculate the enthalpy change for a chemical reaction indirectly. Hess's law
allows us to determine the overall enthalpy change for a reaction by combining
the enthalpy changes of one or more intermediate reactions.
Hess's law is based on the
principle that the enthalpy change of a chemical reaction is independent of the
pathway taken between the initial and final states of the reactants and
products. In other words, the change in enthalpy is a state function, and it
depends only on the initial and final states of the system, not on the specific
steps or intermediates involved in the reaction.
This is particularly useful
in cases where it is challenging or even impossible to directly measure the
enthalpy change of a specific reaction. By using Hess's law, we can determine
the enthalpy change indirectly through a series of intermediate reactions whose
enthalpy changes are known or can be measured experimentally.
The steps to use
Hess's law are as follows:
Identify and write down a
series of intermediate reactions that, when combined, will yield the target
reaction.
Write the balanced chemical
equations for these intermediate reactions, including the known or measured
enthalpy changes (∆H) for each reaction.
Apply the law of
conservation of energy and algebraically manipulate the intermediate reactions
to cancel out any common reactants or products and obtain the target reaction.
The overall enthalpy change
for the target reaction will be the sum of the enthalpy changes for the
intermediate reactions, taking into account the stoichiometric coefficients.
Hess's law is particularly
useful in situations where direct measurements of enthalpy changes are
difficult or impractical, and it has applications in various fields, including
chemical engineering, thermodynamic calculations, and the study of combustion
processes. It allows scientists and engineers to understand and predict the
energetics of complex reactions by using simpler, well-understood reactions.
Q.13.Define enthalpy of transition?
Ans. The enthalpy of transition, also known as the heat
(enthalpy) of phase transition or heat of transformation, refers to the heat
energy change associated with a physical change in a substance from one phase
to another at a constant temperature and pressure. Phase transitions involve
the conversion of matter between different states, such as solid, liquid, and
gas, without any change in chemical composition.
The three primary
types of phase transitions are:
Melting
(fusion): The transition from a
solid to a liquid state, characterized by the absorption of heat energy. The
enthalpy of transition for melting is also known as the heat of fusion.
Freezing: The transition from a liquid to a solid state,
characterized by the release of heat energy. The enthalpy of transition for
freezing is equal in magnitude but opposite in sign to the heat of fusion.
Vaporization
(boiling or evaporation): The
transition from a liquid to a gas state, characterized by the absorption of
heat energy. The enthalpy of transition for vaporization is also known as the
heat of vaporization.
The enthalpy of transition
is quantified as the amount of heat energy required or released per unit mass
(usually per mole) of the substance undergoing the phase change. It is
typically expressed in units of joules per mole (J/mol) or kilojoules per mole
(kJ/mol).
For example, let's consider
the process of water boiling and transitioning from a liquid to a gas at its
boiling point of 100°C (at atmospheric pressure). The heat of vaporization for
water is approximately 40.79 kJ/mol. This means that for each mole of water
vaporized at its boiling point, approximately 40.79 kJ of heat energy is absorbed
from the surroundings.
Similarly, when water
freezes at 0°C (at atmospheric pressure), it releases the same amount of heat
energy (40.79 kJ/mol) per mole in the form of heat of fusion.
The enthalpy of transition
is an important concept in thermodynamics and is used in various applications,
including the design of cooling and heating systems and the study of phase
equilibria in materials.
Q.14.Hess’s law is a corollary of the first
law of thermodynamics comment?
Ans. Hess's law can be considered a corollary of the first law
of thermodynamics, also known as the law of conservation of energy. The first
law of thermodynamics states that energy cannot be created or destroyed in a
closed system; it can only change forms. In other words, the total energy of a
system remains constant.
Hess's law is based on this
fundamental principle of energy conservation. It states that the overall
enthalpy change (∆H) for a chemical reaction is the same, regardless of the
pathway taken between the initial and final states of the reactants and
products. This means that the change in enthalpy is a state function and
depends only on the initial and final states of the system, not on the specific
steps or intermediates involved in the reaction.
To put it another way, if a
chemical reaction can occur via multiple intermediate steps, the sum of the
enthalpy changes for these steps will be equal to the enthalpy change for the
overall reaction. This is in line with the first law of thermodynamics, which
dictates that the total energy change in a system is independent of the
process's specific details.
Mathematically, Hess's
law can be expressed as follows:
∆H (overall) = Σ∆H
(intermediate reactions)
Where:
∆H (overall) is the enthalpy
change for the overall reaction.
Σ∆H (intermediate reactions)
is the sum of the enthalpy changes for the intermediate reactions.
By applying Hess's law,
chemists and thermodynamicists can determine the enthalpy changes for complex
reactions indirectly through a series of simpler, well-understood reactions.
This is extremely valuable when direct measurement of a particular reaction's
enthalpy change is challenging or not feasible.
In summary, Hess's law is a
corollary of the first law of thermodynamics because it is based on the
principle of energy conservation and highlights the relationship between energy
changes and the initial and final states of a system, regardless of the specific
path taken during a chemical reaction.
Q.15.Prove that the decrease in free
energy in a system during a process is equal to the useful work done?
Ans. To prove that the decrease in free energy (∆G) in a
system during a process is equal to the useful work done (w), we can start with
the definition of free energy change and then relate it to the work done.
The change in Gibbs free energy (∆G) for a closed system
is given by the equation:
∆G = ∆H - T∆S
Where:
∆G = Change in Gibbs free
energy
∆H = Change in enthalpy
(heat energy change)
T = Temperature of the
system in Kelvin
∆S = Change in entropy
(disorder) of the system
Now, let's consider a system
undergoing a process, and during this process, it performs work on its
surroundings. According to the first law of thermodynamics, the energy of the
system (U) changes due to heat transfer (Q) and work done (w):
ΔU = Q - w
Since the system is closed
(no matter transfer), the change in internal energy (∆U) is equal to the change
in enthalpy (∆H):
∆H = Q - w
Now, let's rearrange the
equation to isolate Q:
Q = ∆H + w
Next, we can use the
definition of entropy change (∆S) as follows:
∆S = q/T
Where q is the heat
transfer, and T is the absolute temperature.
Now, we'll substitute q with
∆H, as the enthalpy change (∆H) represents the heat transfer at constant
pressure:
∆S = ∆H/T
Now, let's modify the
expression for ∆G:
∆G = ∆H - T∆S
Substitute the value of ∆S:
∆G = ∆H - (∆H/T) * T
∆G = ∆H - ∆H
∆G = 0
Hence, we find that ∆G = 0.
At equilibrium, the free
energy change (∆G) is zero. This means that the system has reached a stable
state with minimal free energy, and no useful work is being done.
However, during a
non-equilibrium process, the decrease in free energy (∆G) is equal to the
useful work done (w):
∆G = -w
Therefore, the decrease in
free energy during a process is indeed equal to the useful work done by the
system.