Chapter 13 Kinetic Theory
CHAPTER NO.13 KINETIC THEORY
13.1 INTRODUCTION
Boyle discovered the law named after him in 1661.
Boyle,Newton and several others tried to explain the behaviour of
gases by considering that gases are made up of tiny
atomic particles. The actual atomic theory got catablished more than
150 years later. Kinetic theory explains the
behaviour of gases based on the idea that the gas consists of rapidly moving
atoms or molecules. This is possible as the
inter-atomic forces,which are short range forces that are important for solids
and liquids, can be neglected for gases. The kinetic theory was developed in
the nineteenth century by Maxwell,
Boltzmann and others. It has been remarkably
successful. It gives a molecular interpretation of pressure and temperature of
a gas, and is consistent with gas laws and Avogadro's hypothesis. It correctly
explains specific heat capacities of Many gases. It also relates measurable
properties of gases
such as viscosity, conduction and diffusion with
molecular parameters, yielding estimates of molecular sizes and masses.This
chapter gives an introduction to kinetic theory.
13.2 MOLECULAR NATURE OF MATTER
Richard Feynman, one of the great physicists of 20th
century considers the discovery that “Matter is made up of atoms” to be a very
significant one. Humanity may suffer annihilation
(due to nuclear catastrophe) or extinction (due to
environmental disasters) if we do not act wisely. If that happens, and all of
scientific knowledge were to be destroyed
then Feynman would like the ‘Atomic Hypothesis’ to
be communicated to the next generation of creatures in the
universe. Atomic Hypothesis: All things are made of
atoms - little particles that move around in perpetual motion,
attracting each other when they are a little
distance apart,but repelling upon being squeezed into one another.
Speculation that matter may not be continuous,
existed in many places and cultures. Kanada in India and Democritus
Atomic Hypothesis tn Anctent India and Greece Though
Jobo Dalton is credited with the introduction of atomic viewpoint fo modern
science, scholars in
andent India and Greece conjectured long before the
existence of atoms and molecules. In the Vaiseshika school of thought in India
founded by Kanada (Sixth century B.C.) the atomic picture was developed in
considerable detail. Atoms were thought to be eternal. indiviaible.
infinitesimal and ultimate parts of matter.It was argued that if matter could
be subdivided without an and, there would be no difference between a mustard
seed and the Meru mountain. The four kinds of atoms (Paramanu — Sanskrit word
for the
analest particle) postulated were Bhoomi (Earth), Ap
(water), Tefas (fre) and Vayu (air) that have characteristic
mass anid other attributes, were propounded. Akasa
(space) was thought to have no atomic structure and was continuous and inet.
Atoms combine to form different molecules (e.g. two atoms combine to fan a
diatomic molecule dvyanuka, three atoms form a tryanuka or a triatomic
molecule), their properties depending upon the mature and ratio of the
constituent atoms. The size of the atoms was also catimated, by conjecture
or by methods that are not known to us. The
estimates vary. In Lalitavistara, a famous biography of the Buddha written
mainly in the second century B.C., the estimate is close to the modem estimate
of atomic aire, of the order of 10m.
In ancient Greece, Democritus (Fourth ceutury B.C.)
is best known for his atomic hypothesis. The word ‘atom’ means ‘indivisible’ in
Greek. According to him, atoms differ from each other physically, in shape,
size and other properties and this resulted in the different propertica of the
substances formed by thetr combination. The atoms of water were smooth and
round and unable to ‘hook’ on to each other, which is why Hquid /water flows
caally. The atoms of carth were rough and jagged, so they held together to form
hard substances. The atoms of fire were thorny which is why it caused painful
burns.These fascinating ideas, despite their ingenuity, could uot evolve much further,
perhaps because they were intuitive conjectures and speculations not tested and
modified by quantitative experiments - the hallmark of modem science.in Greece
had suggested that matter may consist of indivisible constituents. The
scientific ‘Atomic
Theory’ is usually credited to John Dalton. He
proposed the atomic theory to explain the laws of definite and multiple
proportions obeyed by
elements when they combine into compounds.The first
law says that any given compound has,a fixed proportion by mass of its
constituents.The second law says that when two elements form more than one
compound, for a fixed mass
ofone element, the masses of the other elements are
in ratio of small integers.
To explain the laws Dalton suggeated, about 200
years ago, that the smallest constituents of an element are atoms. Atoms of ane
element are identical but differ from those of other elements. A small number
of atoms of each
element combine to form a molecule of the compound.
Gay Lussac’s law, also given in carly 19% century, states: When gases combine
chemically to yield another gas, their volumes are in the ratios of small
integers. Avogadro's
law (or hypothesis) says: Equal volumes of all gases
at equal temperature and pressure have the same number of molecules. Avogadro's
law,when combined with Dalton’s theory explains
Gay Lussac's law. Since the elements are often in
the form of molecules, Daltan’s atomic theory can also be referred to as the
molecular theory of matter. The theory 1s now well accepted by scientists. However
even at the end of the nineteenth century there were famous sctentists who did
not heHeve in atomic theory !
From many observations, in recent times we now know
that molecules (made up of one or more atoms) constitute matter. Electron
microscopes and scanning tunnelling microscopes enable us to even see them. The
size of an atom 1s about an angstrom (10° m).In solids, which are tightly
packed, atoms are spaced about a few angstroms (2 A) apart. In liquids the
separation between atoms is also
about the same. In liquids the atoms are not as
rigidly fixed as in solfds, and can move around, This enables a liquid to flow.
In gases the interatomic distances are in tens of angstroms. The average
distance a molecule can travel without colliding fs called the mean
free path. The mean free path, in gases, is of the
order of thousands of angstroms. The atoms are much freer in gases and can
travel long distances without colliding. If they are not
enclosed, gases disperse away. In solids and liquids
the closeness makes the interatomic force important. The force has a long range
attraction and a short range repulsion. The atoms attract
when they are at a few angstroms but repel when they
come closer. The static appearance ofa gas is misleading. The gas ia fill of activity
and the
equilibrium is a dynamic one. In dynamic
equilibrium, molecules collide and change their
speeds during the collision. Only the average properties are constant.
Atomic theory ia not the end of our queat, but the
beginning. We now know that atoms are not indivisible or elementary. They
consist of a
nucleus and electrons. The nucleus itself is made up
of protons and neutrons. The protons and neutrons are again made up of quarks.
Even
quarks may not be the end of the story. There may be
atring Ifke elementary entities. Nature always has surprises for us, but the
search for truth is often enjoyable and the discoveries
beautiful. In this chapter, we shall limit ourselves
to understanding the behaviour of gases (and a little bit of solids), as a collection
of moving
molecules in incessant motion.
13.3 BEHAVIOUR OF GASES
Properties of gases are easter to understand than
those of solids and Nquids. This is mainly because in a gas, molecules are far
from each other and their mutual interactions are
negligible except when two molecules collide.Gases
at low preasures and high temperatures much above that at which they Hquefy (or
solidify) approximately satisfy a simple relation between their pressure,
temperature and volume
given by (see Ch. 11)PV=kT (13.1)for a given sample
of the gas. Here T ts the temperature in kelvin or (absolute) scale. Kis a
constant for the given sample but varies with the volume of the gas. If we now
bring in the idea of atoms or molecules then Kis proportional
to the number of molecules, (aay} N in the sample.
We can write K = Nk. Observation tells us that this k is same for all gases. It
is called Boltzmann constant and is denoted by k..PV, _ PVo _ _As NT,” NST, =
constant = k, (13.2)
if P, Vand Tare same, then N is also same for all
gases. This is Avogadro's hypothesis, that the mumber of molecules per unit
volume is same for all gases at a fixed temperature and pressure.
The number in 22.4 litres of any gas is 6.02 x 10%,
This is known as Avogadro number and is denoted by N,. The mass of 22.4 litres
of any gas is equal to its molecular weight in grams at S.T.P (standard
temperature 273 K and pressure
1 atm). Thia a mount of substance ts called a mole
(see Chapter 2 for a more precise definition).Avogadro had guessed the equality
of numbers in equal volumes of gas at a fixed temperature and pressure from
chemical reactions. Kinetic theory fustifies this hypothesis.The perfect gas
equation can be written as PV =p RT (13.3)where » is the number of moles and R
= N,k, is a universal constant. The temperature Tis
absolute temperature. Choosing kelvin scale for John
Dalton (1788 1844)
He was ap Engiish chemist. When dfffcrent types of
atoms combine,
they obcy certain simple laws. Dalton’s atomic
theory explains these laws in a simple way. He also gave a theory of colour
blindness.Amedeo Avogairo (1778 — 1858)He made a brilHant gucss that equal
volumes of gasca have cqual number of moleculcs at the same temperature and
pressure. This helped in understanding the combination of different gases in
@ very simple way. It is now called Avogadro’s
hypothesis (or law). He also
suggested that the smallcst constituent of gasca
like hydrogen, oxygen and
nitrogen are not atoms but diatomic
molecules.absolute temperature, R = 8.314 J mof'K".Here M N N= aE =H
(13.4)
where M is the mass of the gas containing N
molecules, M, is the molar mass and N, the Avogadro's number. Using Egs. (13.4)
and (13.3)can also be written as PV=k,NT or P=k, nT where nis the number
density, i.e. number of
molecules per unit volume. i, is the Boltzmann
constant introduced above. Its value in SI units is 1.38 x 10" JK}.
Another useful form of Eq. (13.3) is
RT pe a (13.5) where p is the mass density of the
gas.A gas that satisfies Eg. (13.3) exactly at all pressures and temperatures
is defined to be an
ideal gas. An ideal gas is a simple theoretical
model of a gas. No real gas is truly ideal.Fig. 13.1 shows departures from
ideal gas behaviour for a real gas at three different temperatures. Notice that
all curves approach the ideal gas behaviour for low pressures and high
temperatures.
At low pressures or high temperatures the molecules
are far apart and molecular interactions are negligible. Without interactions
the gas behaves like an ideal one.If we fix » and Tin Eq. (13.3), we get PV =
constant (13.6)
Le., keeping temperature constant, pressure of a
given mass of gas varies inversely with volume.This is the famous Boyle's law.
Fig. 13.2 shows comparison between experimental P-V curves and the theoretical
curves predicted by Boyle's
law. Once again you see that the agreement is good
at high temperatures and low pressures.Next, if you fix P, Eq. (13.1) shows
that Ve T iLe., for a fixed pressure, the volume of a gas is
proportional to its absolute temperature T (Charies’
law). See Fig. 13.3.
Finally, consider a mixture of non-interacting ideal
gases: 4, moles of gas 1, », moles of gas 2, etc. in a veasel of volume V at
temperature T and pressure P. It is then found that the
equation of state of the mixture is :
PV=(4,+4,+... ) RE (13.7)
RT RT
Le. P=, Vv + My Vv tu. (13.8)
=P\+P,+... (13.9)
Clearly P,= y, RT/V is the pressure gas 1 would exert at the same conditions of volume
and temperature ifno other gases were present.This is called the partial
pressure of the gas.Thus, the total pressure of a mixture of ideal
gases is the sum of partial pressures. This is
Dalton’s law of partial pressures.
We next consider some examples which give us
information about the volume occupied by the molecules and the volume of a
single molecule.Example 13.1 The density of wateris 1000 kgm. The denstty of
water vapour at 100 °C and 1 atm pressure is 0.6 kg m’%. The volume of a
molecule multiplied by the total
number gives ,what fs called, molecular
vohime. Esttmate the ratio (or fraction) of the molecular
volume to the total volume occupied by the water vapour under the above
conditions of temperature and pressure.
Answer For a given mass of water molecules,the
density is less if volume is lange. So the volume of the vapour is 1000/0.6 =
/(6 x10 *) times larger. If densities of bulk water and water
molecules are same, then the fraction of
molecular volume to the total volume in liquid state
is 1. As volume in vapour state has increased, the fractional volume is less by
the same amount, fc. 6x10.
Example 13.2 Estimate the volume of a
water molecule using the data in Example
13.1.
Answer In the liquid (or solid) phase, the molecules
of water are quite closely packed. The density of water molecule may therefore,
be regarded as roughly equal to the density of bulk water = 1000 kg mr. To
eatimate the vohime of
a water molecule, we need to know the mass of a
single water molecule. We know that 1 mole of water has a mass approximately
equal to (2+16)g =18¢g = 0.018 kg.
Since 1 mole contains about 6x 10”
10%) kg = 3x 10% kg. Therefore, a rough estimate of
the volume of a water molecule is as follows :
Volume of a water molecule
= x 10 kgj/ (1000 kg m*)
=3x 107 m?®
= (4/3) x (Radius)®
Hence, Radius = 2 x10 m=2A Example 18.3. What is the
average distance between atoms (interatomic distance) in water? Use the data
gtven in Examples 13.1 and 13.2.
Answer: Agiven maas of water in vapour state has
1.67x10° times the vohime of the same mass of water in liquid state (Ex. 13.1).
This is also the increase in the amount of volume available
for each molecule of water. When volume
increases by 10° times the radius increasea by Vv‘
or 10 times, f.e., 10 x 2A = 20 A. So the average distance is 2 x 20 =40 A <
Example 13.4 A vessel contains two non-
reactive gases : neon (monatomic) and
oxygen (diatomic). The ratio of their partial
pressures is 3:2. Eattmate the ratio of (f number of molecules and (t) mass
density of neon and oxygen in the vessel. Atomic mass of Ne = 20.2 1, molecular
mass of O,= 32.0 u.
Answer Partial pressure of a gas in a mixture is the
pressure it would have for the same volume and temperature ff it alone occupied
the vessel.(The total pressure of a mixture of non-reactive
gases is the sum of partial pressures due to its
constituent gases.) Each gas (assumed ideal)obeys the gaa law. Since Vand Tare
common to the two gases, we have P,V=p, RTand hV=#, RT, Le. (P,/P,) = (, / u,).
Here 1 and 2 refer
to neon and oxygen respectively. Since (P,/P,) =
(3/2) (given). (4,/ #,) = 3/2.
By definition #, = (N,/N,) and 4, = (N,/N,)where N,
and N, are the number of molecules of 1 and 2, and N, ts the Avogadro's
number.Therefore, (N,/N,) = (4, / #.) = 3/2.
(i) We can also write yw, = (m,/M,) and y, = (m,/M,)
where m, and m, are the masses of 1 and 2; and M, and M, are their molecular
masses. (Both m, and M,; a9 well as m, and M, should be expreased in the same
units).If p, and p, are the mass densities of 1 and 2 respectively, we have Bm
mM P2 mz /Voomz, He \M;;
3 20.2 a -2% 3997 0-947 <4
13.4 KINETIC THEORY OF AN IDEAL GAS
Kinetic theory of gases is based on the molecular
picture of matter. A given amount of gas is a collection of a large number of
molecules (typically of the order of Avogadro's number) that
are in incessant random motion. At ordinary pressure
and temperature, the average distance between molecules is a factor of 10 or
more than the typical size of a molecule (2 A). Thus the
interaction between the molecules {s negligible and
we can assume that they move freely in straight lines according to Newton’s
first law.
However, occasionally, they come close to each
other, experience intermolecular forces and thetr velocities change. These
interactions are called
collisions. The molecules collide incessantly
against each other or with the walls and change their velocities. The
collisions are considered to
be elastic. We can derive an expression for the
pressure of a gas based on the kinetic theory.
We begin with the idea that molecules of a gas are
in incessant random motion, colliding against one another and with the walls of
the container. All collisions between molecules among themselves or between
molecules and the
walls are elastic. This implies that total kinetic
energy is conserved. The total momentum ts conserved as usual.
13.4.1 Pressure of an Ideal Gas
Conaider a gas enclosed in a cube of side 1 Take the
axes to be parallel to the sides of the cube,as shown in Fig. 13.4. A molecule
with velocity
(u, v,, ¥,) hits the planar wall parallel to yz-
plane of area A {= P). Since the collision is elastic,
the molecule rebounds with the same velocity;its y
and z components of velocity do not change in the collision but the xcomponent
reverses sign. That is, the velocity after collision is
(-v, vu, ¥,) . The change in momentum of the
molecule is} mw, — (mw) = — 2mv,. By the principle of conservation of momentum,
the momenttmn imparted to the wall in the collision =2mv, .
To calculate the force (and pressure) on the wall,
we need to calculate momentum imparted to the wall per unit time. In a small
time interval At, a molecule with «component of velocity v,will hit the wall if
it is within the distance v, At
from the wall. That is, all molecules within the
volume Av, At only can hit the wall in time At.But, on the average, half of
these are moving towards the wall and the other half away from
the wall. Thus the niunber of molecules with
velocity (v, u,v.) hitting the wall in thne At is 4A v, Atn where nis the
number of molecules per unit volume. The total momentum transferred to the wall
by theae molecules in time At 1s:
O = (2m) (% nAv, At) (13.10)
The force on the wall is the rate of momentum
transfer QO/At and pressure is force per unit area :P= 9 /{(AAO = nmv? (3.11)
Actually, all molecules in a gas do not have the same velocity; there is a
distribution in velocities. The above equation therefore, stands for pressure
due to the group of molecules with
speed v, in the x-direction and n stands for the
number density of that group of molecules. The total pressure is obtained by
summing over the contribution due to all groups:
P=nmv. (13.12)
where. ia the average of u,? . Now the gas is
isotropic, i.e. there is no preferred direction of velocity of the molecules in
the vessel.Therefore, by symmetry,geuee =(1/3)[u2 + of + )=0/3) 13.13)where v
is the speed and »? denotes the mean of the squared speed. Thus P=(1/3) am
(13.14)
Some remarks on this derivation. Firat,
though we choose the container to be a cube,the
shape of the vessel really is immaterial. For a vessel of arbitrary shape, we
can always choose a small infinitesimal (planar) area and carry
through the steps above. Notice that both Aand At do
not appear in the final result. By Pascal's law, given in Ch. 10, pressure fn
one portion of the gas in equilfbrium is the same as anywhere else. Second, we
have ignored any collisions in the derivation. Though this assumption is
difficult to justify rigorously, we can qualitatively
see that it will not lead to erroneous results.The
mumber of molecules hitting the wall in time At-was found to be %4 n Av, At Now
the collisfons are random and the gas 1s in a steady state.Thus, if a molecule
with velocity (v, uv, u, )
acquires a different velocity due to collision with
some molecule, there will always be some other molecule with a different
initial velocity which
after a collision acquires the velocity (v, v,,
v,).Hf this were not so, the distribution of velocities would not remain
ateady. In any case we are
finding .° . Thus, on the whole, molecular
collisions (if they are not too frequent and the time spent in a colliston is
negligible compared
to time between collisions) will not affect the
calculation above.
13.4.2 Kinetic Interpretation of Temperature
Equation (13.14) can be written as PV = (1/3) nVm v? (13.15a)
Founders of Kinetic Theory of Gases
rn — — = James Clerk Maxwell (1831 -— 1879}, born in
Edinburgh,Scotland, was among the greatest physicists of the nineteenth
century. He dertved the thermal velocity diatribution of molecules in a gas and
waa among the first to obtain reHable estimates of
f . molecular parameters from measurable quantities
like viscosity,rad y etc. Maxwell's greateat achievement was the unification of
the laws . of electricity and magnetiam (discovered by Coulomb, Oersted,Ampere
and Faraday) into a consistent set of equations now called
, Maxwell's equationa. From these he arrived at the
most important conclusion that light fa an x electromagnetic wave.
LN re Interestingly, Maxwell did not a
vs agree with the idea (strongly a
> suggested by the Faraday’s we
. laws of electrolysia) that f electricity was
particulate in ;
@ nature. "Ate et ‘| Ss }Ludwig Boltzmann f
(1844-19086) born in 2 ’
Vienna, Austria, worked on the kinetic theory of
gases ‘independently of Maxwell. A firm advocate of atomiam, that is Cr
"basic to kinetic theory, Holtzmann provided a statistical * ;
interpretation af the Second Law of thermodynamics
and the f Fy "i ,
concept of entropy. He is regarded as one of the
founders of dlasairal t ro
statistical mechanics. The proportionality constant
connecting . ; , oan energy and temperature in kinetic theory is known aa
Boltzmann's 5 * oe & i constant in his honour. a a
PV = (2/3)Nx¥%m (13.15b)where N (= nV) is the mumber
of molecules in the sample.
The quantity in the bracket is the average
translational kinetic energy of the molecules in the gas. Since the interna]
energy E of an ideal gas is purely kinetic’,
E=Nx (1/2 m (13.16)
Equation (13.15) then gives :
PV=(2/3)E (13.17)
We are now ready for a kinetic interpretation of
temperature. Combining Eq. (13.17) with the ideal gas Eq. (13.3), we get
E=(3/2) k, NT (13.18)
ao E/N=e%my = 8/2kT (13.19)f.e., the average kinetic
energy of a molecule is
proportional to the absolute temperature of the gas;
it is independent of pressure, volume or the nature of the ideal gas. This is a
fundamental
result relating temperature, a macroscopic
measurable parameter of aie gas (a thermodynamic variable as it is called) to a
molecular quantity, namely the average kinetic energy of a molecule. The two
domains are
connected by the Boltzmann constant We note in
passing that Eq. (13.18) tells us that internal energy of an ideal gas depends
only on temperature, not on pressure or vohime. With this interpretation of
temperature, kinetic theory of an ideal gas is completely consistent with the
ideal gas equation and the various gas laws based on it
For a mixture of non-reactive ideal gases, the total
pressure gets contribution from each gas in the mixture. Equation (13.14) becomes
P=(1/9) [am vo? tm, v2 +... ] (13.20)
In equilibrium, the average kinetic energy of the
molecules of different gases will be equal.That is,am yi =¥m, v= 6/Ik,T so that
P=(n, +7, +... )k,T (13.21) which is Dalton's law of partial pressures.From Eq.
(13.19), we can get an idea of the typical speed of molecules in a gas. At a
temperature T= 300 K, the mean square speed of a molecule in nitrogen gas is :
My, 28 26
=—s= ola 4, 10"
m= T= Go2xlom™ ~ VOO* 10" keg.
v =3k,T/m = (616? ms?
The square root of »? is known aa root mean square
(rms) speed and is denoted by v,,,,(We canalsowrite (° as <v*>)
v4, = 516me!
The speed is of the order of the speed of sound in
air. It follows from Eq. (13.19) that at the same temperature, lighter
molecules have greater rms
speed.Exampie 13.5 A flask contains argon and
chlorine in the ratio of 2:1 by mass. The temperature of the mixture is 27 °C.
Obtain the ratto of () average kinetic energy per molecule, and (ti) root mean
square speed v_,, Of the molecules of the two gases.Atomic mass of argon = 39.9
u; Molecular mass of chlorine = 70.9 u.
Answer The important point to remember is that the
average kinetic energy (per molecule) of any Gidea) gas (be it monatomic like
argon, diatomic like chlorine or polyatomic) is always equal to (8/2) k,T. It
depends only on temperature, and is independent of the nature of the gas.
Since argon and chlorine both have the same
temperature in the flask, the ratio of average kinetic energy {per molecule) of
the two gases is 1:1.
(i) Now % mu__,? = average kinetic energy per
molecule = (8/2) ) k,T where m is the mass of a molecule of the gas.
Therefore,(View), (M. (M), “79.9
(v.)~(m),. 7 (), = 399 =h77 (Vins hey ( rt ne ( dat
39.9
where Mdenotes the molecular mass of the gas.(For
argon, a molecule is just an atom of argon.)Taking square root of both
sides,(Vins Ve 1 33 (Vines Jey ~ .
You should note that the composition of the mixhure
by mass is quite trrelevant to the above Maxwell Distribution Function In a
given mass of gas, the velocities of all molecules are not the same, even when
bulk parameters like pressure, volume and temperature are fixed. Collisions
change the direction
and the speed of molecules. However in a state of
equilibrium, the distribution of speeds is constant or fixed.
Distributions are very important and useful when
dealing with systems containing large number of objects. As an example consider
the ages of different persons in a city. It ts not
feasible to deal with the age of each individual. We
can divide the people into groups: children up to age 20 years, adulte between
ages of 20 and 60, old people above 60. If we want more
detailed information we can choose smaller
intervals, 0-1, 1-2.,.... 99-100 ofage groups. When the size of the interval
becomes smaller, say half year, the number of persons in the interval
will also reduce, roughly half the original number
tn the one year interval. The number of persons dN(¥ in the age interval xand
x+d.xia proportional to dx or dN = n, dx. We have used n,, to denote the number
of persons at the value of x.
SK 9 0.5 1 Av 15 2.0 ©/ Orns}
Maxwell distribution of molecular speeds
In a similar way the molecular speed distribution
gives the number of molecules between the speeda v and vt dv, dMv) = 4p N @e*
v? dv =ndv. This is called Maxwell distribution.The plot ofn, against v is
shown in the figure. The fraction of the molecules with speeds v and v+dv is
equal to the area of the strip shown. The average of any quantity like vu? is
defined by
the integral <u> = (1/N) [= dMu) = Vk, T/m
which agrees with the result derived from more elementary considerations.
calculation. Any other proportion by mass of argon
and chlorine would give the same answers to (0 and (i), provided the
temperature remains unaltered. 4
Example 13.6 Uranium has two isotopes
of masses 235 and 238 units. If both are
present in Urantum hexafluoride gas which would have
the larger average speed ? If atomic mass of fluorine is 19 units,estimate the
percentage difference in speeds at any temperature.
Answer At a fixed temperature the average energy = 4
m <vu’> is constant. So smaller the mass of the molecule, faster will be
the speed.The ratio of speeds is {inversely proportional to
the square root of the ratio of the masses. The
maasaes are 349 and 352 units. So Us / Ugg, = (352/349) = 1.0044.Hence
difference “= 0.44%,
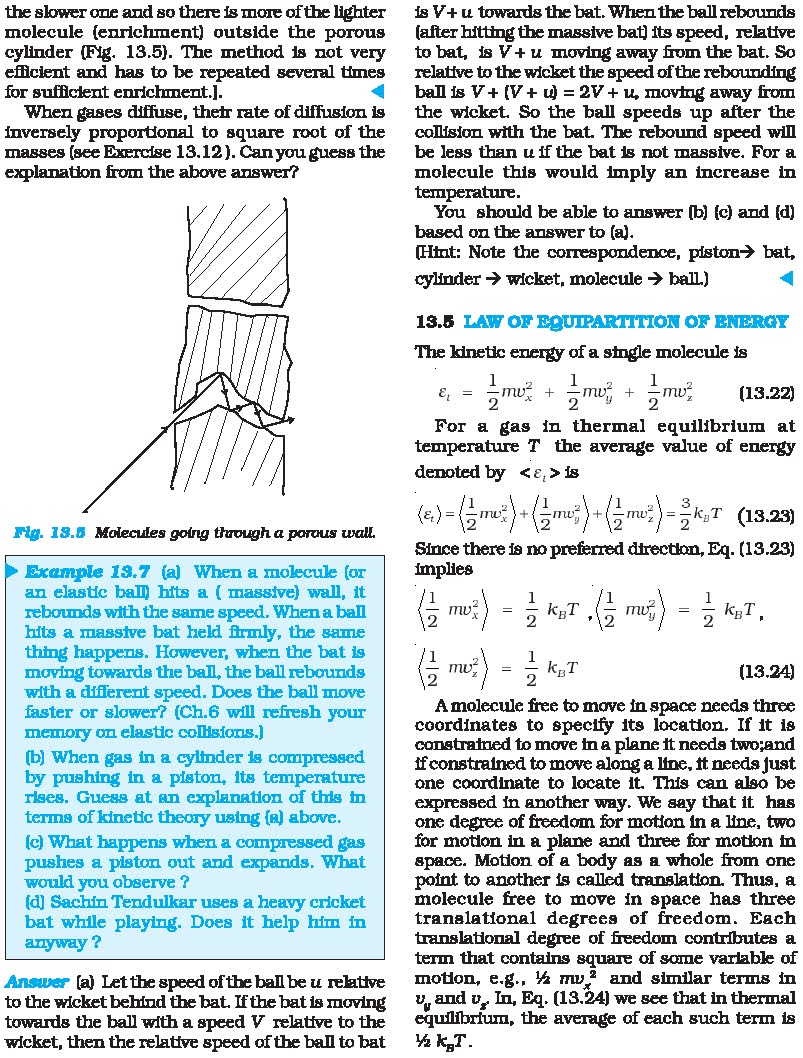
[*U is the isotope needed for nuclear fisaion.To
separate tt from the more abundant isotope 281], the mixture {ts surrounded by
a porous cylinder. The porous cylinder must be thick and
narrow, so that the molecule wanders through
individually, colliding with the walis of the long pore. The faster molecule
will leak ont more than the slower one and so there is more of the lighter
molecule (enrichment) outside the porous cylinder (Fig. 13.5). The method is not
very efficient and has to be repeated several times for sufficient
enrichment.]}.When gases diffuse, their rate of diffusion is inversely
proportional to square root of the
masses (see Exercise 13.12). Can you guess the
explanation from the above answer?
Example 13.7 (a) When a molecule (or
an elastic bal) hits a ( massive) wall, it rebounds
with the same speed. When a ball hits a massive bat held firmly, the same thing
happens. However, when the bat is moving towards the ball, the ball rebounds
with a different speed. Does the ball move faster or slower? (Ch.6 will refresh
your memory on elastic colHsions.)
(b) When gas in a cylinder is compressed
by pushing in a piston, its temperature
rises. Gueas at an explanation of this in terms of
kinetic theory using {a) above.
(c) What happens when a compressed gas
pushes a piston out and expands. What
would you observe ?
(d) Sachin Tendulkar uses a heavy cricket bat while
playing. Does it help him in anyway ?
Answer (a) Let the speed of the ball be u relative
to the wicket behind the bat. If the bat is moving towards the ball with a
speed V relative to the
wicket, then the relative speed of the ball to bat
is V+ u towards the bat. When the ball rebounds (after hitting the massive bat)
its speed, relative
to bat, is V+ u moving away from the bat. So
relative to the wicket the speed of the rebounding ball is V+ (V+ = 2V + u,
moving away from the wicket. So the ball speeds up after the collision with the
bat. The rebound speed will
be less than u if the bat is not massive. Fora
molecule this would imply an increase in temperature.You should be able to
answer (b) (c) and (d)based on the answer to (a).(Hint: Note the
correspondence, piston> bat,cylinder > wicket, molecule > ball.)
13.5 LAW OF EQUIPARTITION OF ENERGY
The kinetic energy of a single molecule is F = Lint
+ Ame + Lin? 13.2 67 9 x 2 Yy 2 z ( . 2)For a gas in thermal equilibrium at
temperature T the average value of energy denoted by <<¢,>is
, 1 A fi oh fl a\ 3 {e) = G me’) + (Jie ) + G me?)
=o k,T (13.23)Since there is no preferred direction, Eq. (13.23)
implies Low?) = dit (2 me\ = 4 G me’) = 9 k,T AG
mui) = 9 kT ,Jl 4 1 G mei) = 5 Ket (13.24)
Amolecule free to move in space needs three
coordinates to specify its location. If it is constrained to move in a plane it
needa two;and ifconstrained to move along a line, it needs just one coordinate
to locate it. This can also be expreased in another way. We say that it has one
degree of freedom for motion in a line, two
for motion in a plane and three for motion in space.
Motion of a body as a whole from one point to another is called translation.
Thus, a molecule free to move in space has three
tranalational degreea of freedom. Each
translational degree of freedom contributes a term
that contains square of some variable of motion, e.g., % mv? and similar terms
in uy, and vy In, Eq. (13.24) we see that in thermal equilibrium, the average
of each such term is “’k,f.
Molecules of a monatomic gas like argon have only
translational degrees of freedom. But what about a diatomic gas such as O, or
N,? A molecule of O, has three translational degrees of freedom. But in
addition it can also rotate
about its centre of mass. Figure 13.6 shows the two
independent axes ofrotation 1 and 2, normal to the axis joining the two oxygen
atoms about
which the molecule can rotate®. The molecule thus has two rotational degrees of freedom, each of which contributes a term to the total energy consisting of translational energy <, and rotational energy <,Po. dow Tog da ly Et Ea pM + Fey + pes + gae + ghe (19.25)
where , and ,
are the angular speeds about the axea 1 and 2 and J, L are the corresponding
moments of inertia. Note that each rotational degree
of freedom contributes a term to the energy that contains square of a
rotational variable of motion.
We have assumed above that the O, molecule is a
‘rigid rotator’, i.e. the molecule does not vibrate. This assumption, though
found to be
true (at moderate temperatures) for O,, is not
always valid. Molecules like CO even at moderate temperatures have a mode of
vibration, i.e. its atoms oscillate along the interatomic axis like a
one-dimensional oscillator, and contribute a vibrational energy term e, to the
total energy:
1 fdy¥ 1, ,
zm dt | + gh
€=& + €, +€, (13.26)
where k is the force constant of the oscillator and
y the vibrational co-ordinate.Once again the vibrational energy terms in Eq.
(13.26) contain squared terms of vibrational variables of motion y and dy/dt
.At this point, notice an important feature in Eq.(13.26). While each
translational and
rotational degree of freedom has contributed only
one ‘squared term’ in Eq.(13.26), one vibrational mode contributes two ‘squared
terms’ : kinetic and potential energies.
Each quadratic term occurring in the
expression for energy is a mode of absorption of
energy by the molecule. We have seen that in thermal equilibrium at absohite
temperature T,for each translational mode of motion, the
average energy is 44 kT. Amost elegant principle of
classical statistical mechanics (first proved by Maxwell states that this is so
for each mode
of energy: translational, rotational and
vibrational. That is, in equilibrium, the total
energy ia equally distributed in all possible energy modes, with each mode
having an average energy equal to % k,T. This ia known aa the law of
equipartition of energy. Accordingly,
each translational and rotational degree of freedom
ofa molecule contributes % k,T to the energy while each vibrational frequency
contributes 2x 4 kT =k,T, since a vibrational mode has both kinetic and potential
energy
modes.
The proof of the law of equipartition of energy is
beyond the scope of this book. Here we shall apply the law to predict the
specific heats of gases theoretically. Later we shall also discuss briefly, the
application to specific heat of solida.
13.6 SPECIFIC HEAT CAPACITY
13.6.1 Monatomic Gases The molecule of a monatomic
gas has only three translational degrees of freedom. Thus, the average energy
of a molecule at temperature T is (3/2)k,T . The total internal energy of a
mole of such a gas is U =3k,TxN,=2RT
(13.27)2 2
The molar specific heat at constant volume,C,, is
‘dU 3 C, (monatomic gas) = ar = RT (13.28)For an ideal gas,
C,-C,=R (13.29)where C, is the molar specific heat
at constant pressure. Thus,C,=2R (13.30)2
The ratio of apectic heats y = a - 3 (13.31) 13.6.2
Diatomic Gases
As explained eartiier, a diatomic molecule treated
aa a rigid rotator like a dumbbell has 5 degrees of freedom : 3 translational
and 2 rotational.
Uatng the law of equipartition of energy, the total internal
energy of a mole of auch a gas is 175 5 U= g ket x Ny = 3 RT (13.32)The molar
specific heats are then given by C, (rigid diatomic) = 3B C= aR (13.33)7
y (rigid diatomic) = = (13.34)If the diatomic
molecule is not rigid but has
in addition a vibrational mode | 5 7
U -( Faure kT ps = —RkT 2 2 7 9 9
=—R. C,5=R yas .C, 2 C, gry 7R (13.35)
13.6.9 Polyatomic Gases
In general a polyatomic molecule has 3
translational, 3 rotational degrees of freedom
and a certain number (f) of vibrational
modes.According to the law of equipartition of energy,it is easily seen that
one mole of such a gas has
u=(@ kr+ 2 kr+fk,T) N,2 2 Le. C,=B+fR C=44+R (4+)f
(3+ f) 118.36)
Note that C,- C, = R is true for any ideal gas,
whether mono, di or polyatomic.
Table 13.1 summarises the theoretical
predictions for specific heats of gases ignoring any
vibrational modes of motion. The values are in good agreement with experimental
values of specific heats of several gases given in Table 13.2.Of course, there
are discrepancies between predicted and actual values of specific heats of
several other gases (aot shown in the table), such
as CL, C,H, and many other polyatomic gases.Usually,
the experimental values for specific heats of these gases are greater than the
predicted values given in Table13.1 suggesting that the agreement can be
improved by tncloding
vibrational modes of motion in the calculation.The law of equtpartition of energy is thus
well verified experimentally at ordinary
temperatures.
Example 13.8 A cylinder of fixed capacity 44.8 Htres
contains helium gas at standard temperature and pressure. What is the amount of
heat needed to raise the temperature of the gas in the cylinder by 15.0 °C ?
(R= 8.31 Jmol! K).
Answer Using the gas law PV = #RT, you can easily
show that 1 mol of any (ideal) gas at standard temperature (273 K) and pressure
(1 atm = 1.01 x 10° Pa) occupies a volume of 22.4 litres. This universal vohime
is called molar
volume. Thus the cylinder in this example contains 2
mol of helium. Further, since helium is monatomic, its predicted (and observed)
molar
specific heat at constant volume, C, = (3/2) R,and
molar specific heat at constant pressure,C_=(8/2) R+ R= 6/2) R. Since the
volume of the cylinder is fixed, the heat required {is determined by C,,
Therefore,Heat required = no. of moles x molar specific heat x rise in
temperature =2x*%15Rx15.0=45R
= 45 x 8.31 = 374 J.
19.6.4 Specific Heat Capacity of Solids
We can use the law of equipartition of energy
todetermine specific heats of solids. Consider a solid of N atoms, cach
vibrating about its mean
postition. An oscfllation in one dimension has
average energy of 2x % k,T=k,T . In three dimensiona, the average energy is 3
kT. For a
mole of solid, N = N,, and the total
energy is U= 3 kT xN, =3 RT
Now at constant pressure AQ = AU + PAV
= AU, since for a solid AV is negligible. Hence,AQ
Au C= AT AF 3R (13.37)
As Table 13.3 shows the prediction generally agrees
with experimental values at ordinary temperature (Carbon is an exception).
19.6.6 Specific Heat Capacity of Water
We treat water like a solid. For each atom average
energy is 3k,T. Water molecule has three atoms,two hydrogen and one oxygen. So
it has U=3x%3k,T x N, =9RT and C = AQ/ AT=A U / AT =9R.
This is the value observed and the agreement is very
good. In the calorie, gram, degree units,water is defined to have unit specific
heat. As 1 calorie = 4.179 joules and one mole of water is 18 grams, the heat
capacity per mole is
~ 75 J mol! K'~ 9R. However with more
complex molecules like alcchol or acetone the arguments,
based on degrees of freedom, become More complicated.
Lastly, we should note an important aspect of the
predictions of specific heats, based on the classical law of equipartition of
energy. The predicted specific heats are independent of
temperature. As we go to low temperatures,however,
there is a marked departure from this prediction. Specific heats of all
substances approach zero as T>0. This is related to the fact that degrees of
freedom get frozen and
ineffective at low temperatures. According to
classical physica degrees of freedom must remain unchanged at all times. The
behaviour of apecific heats at low temperatures shows the inadequacy of
classical physics and can be
explained only by invoking quantum
considerations, as was first shown by
Einstein.Quantum mechanics requires a minimum,nonzero amount of energy before a
degree of freedom comes into play. This is also the reason why vibrational
degrees of freedom come into play only in some cases.
19.7 MEAN FREE PATH
Molecules in a gas have rather large speeds of the
order of the speed ofaound. Yet a gas leaking from a cylinder in a kitchen
takes considerable
time to diffuse to the other corners of the room.The
top of a cloud of smoke holds together for hours. This happens because
molecules in a gas have a finite though small size, so they are bound
to undergo collisions. As a result, they cannot
Seoing is Believing
Can one see atoms rushing about, Almost but not
quite. One can see pollen graine of a flower being pushed around by molecules
of water. The size of the grain is ~ 10% m. In 1827, a Scottish botanist Robert
Brown, while examining, under a microscope, pollen graina of a flower suspended
in water noticed that they continuously moved about in a zigzag, random
fashion.
Kinetic theory provides a simple explanation of the
phenomenon. Any object suspended in water is
continuously bombarded from all sides by the water
molecules. Since the motion of molecules is random,the number of molecules
hitting the object in any direction is about the same as the number hitting in
the opposite direction. The amall difference between these molecular hita is
negligible compared to the total number of hita for an object of ordinary aize,
and we do not notice any movement of the object.
When the object is sufficiently amall but still
visible under a microscope, the difference in molecular hits from different
directions is not altogether negligible, i.e, the impulses and the torques
given to the suspended object through continuous bombardment by the molecules
of the medium (water or some
other fluid) do not exactly sum to gero. There is a
net impulse and torque in this or that direction. The suspended object thus,
moves about in a zigzag manner and tumblea about randomly. This motion called
now ‘Brownian motion’ is a visible proof of molecular activity. In the last 50
years or so molecules
have been seen by scanning tunneling and other
special microscopes.
In 1987 Ahmed Zewail, an Egyptian scientist working
in USA was able to observe not only the molecules but also their detailed
interactions, He did this by illuminating them with flashes of laser ight for
very short durations, of the order of tens of femtoseconds and photographing
them. [( 1 femto-second = 10 a), One could atudy even the formation and breaking
of chemical bonds. That is really seeing !move straight unhindered; their paths
keep getting incessantly deflected.
Suppose the molecules of a gas are spheres of
diameter d. Focus on a single molecule with the average speed <u>. It
will suffer collision with any molecule that comes within a distance d
between the centres. In time Af, ft sweeps a volume
m# <y> At wherein any other molecule will collide with it (see Fig.
13.7). If n is the number of molecules per unit volume, the
molecule suffers nad? <u> At collisions in
time At. Thus the rate of collisions is nad? <u> or the time between two
successive collisions is on the
average,t =lf/ureu &) (13.38)
The average distance between two succeasive
collisions, called the mean free path 1, is :l=<w t= 1/(nad (13.39)
In this derivation, we imagined the other molecules
to be at rest. But actually all molecules are moving and the collision rate is
determined
by the average relative velocity of the
molecules.Thus we need to replace <y> by <v> in Eq.(13.38). A more
exact treatment gives" l= 1/(¥2 nzd*) (13.40)
Let us estimate land t for air molecules with
average speeds <u> = (485m/s). At STP (0.02 x 10**}m= (22.4107)=
2.7x10%m*> Taking, d=2 x 10 m,t=6.1% 10%
and t= 2.9 x 107 me 1500d (13.41)
As expected, the mean free path given by
Eq. (13.40) depends inversely on the number density
and the size of the molecules. Ina highly evacuated tube n is rather small and
the mean free path can be as large as the length of the
tube.
Example 13.9 Estimate the mean free path
for a water molecule in water vapour at 373 K.Use
information from Exercises 13.1 and Eq. (13.41) above.
Answer The d for water vapour is same as that of
air. The number density is inversely proportional to absolute temperature.ae 2s
273 9 as So n=2.7 10°" x 3732 x10°m Hence, mean free path |= 4 x10" m
S Note that the mean free path is 100 times the interatomic distance ~ 40
A=4%10*m calculated eartier. It is this large value of mean free path that
leads to the typical gaseous behaviour. Gases can
not be confined without a container.Using, the kinetic theory of gases, the
bufk Measurable properties like viscosity, heat conductivity and diffusion can
be related to the
microscopic parameters like molecular size. It is
through such relations that the molecular sizes were firat estimated.
SUMMARY
1. The ideal gas equation connecting pressure (P),
volume (V) and absolute temperature (T) is PV=URT ok, NT
where pis the number of moles and Nis the number of
molecules. R and k, are universal constants._R R=8.314J mark", k, = Na =
1,38 x 108% JK" Real gases satisfy the ideal gas equation only
approximately, more so at low pressures
and high temperatures.
2. Kinetic theory of an ideal gas gives the relation
1 2 pas 2 3 amv where n is number density of molecules, m the masa of the
molecule and we ia the mean of squared speed. Combined with the ideal gas
equation it yields a kinetic
interpretation of temperature.los 3 payee Skat gm =
ket, Ons = (0 } ~ m
This tells us that the temperature of a gas is a
measure of the average kinetic energy of a molecule, tndependent of the nature
of the gas or molecule. In a mixture of gases at a fixed temperature the
heavier molecule has the lower average speed.
3. The translational kinetic energy
E> k, NT.2 This leads to a relation
PV= - E
4. The law of equipartition of energy states that if
a system is in equilibrium at absolute temperature 7, the total energy is
distributed equally in different energy modes of
absorption, the energy in each mode being equal to %
k, T. Each translational and rotational degree of freedom corresponds to one
energy mode of absorption and has energy ¥% k, T. Each vibrational frequency
hae two modes of energy (kinetic and potential}
with corresponding energy equal to
2x %k, T=k, T.
5. Using the law of equtpartition of energy. the
molar specific heats of gases can be determined and the values are in agreement
with the experimental values of specific heats of several gases. The agreement
can be tmmproved by including vibrational modes of motion.
6. The mean free path ! is the average distance
covered by a molecule between two successive collisions :z___} © f2nznd? where
nis the number density and d the diameter of the molecule.
POINTS TO PONDER
1.‘ Pressure of a fluid is not only exerted on the
wall. Pressure exists everywhere in a fhuid.Any layer of gas inside the volume
of a container is in equilibrhim because the pressure
is the same on both sides of the layer.
2. We should not have an exaggerated idea of the
Mtermolecular distance IN a gas. At ordinary pressures and temperatures, this
is only 10 times or so the interatomic distance in solids and Hquidsa. What is
different is the mean free path which in a gas is 100
times the interatomic distance and 1000 times the
aize of the molecule.
3. The law of eq“uipartition of energy is stated
thus: the energy for each degree of freedom in thermal equilbrium is 4% kT.
Each quadratic term in the total energy expression of a molecule is to be
counted as a degree of freedom. Thus, each vibrational mode gives
2 fot 1) degrees of freedom (kinetic and potential
energy modes}, corresponding to the energy 2x 4k T=k_ T.3B B
4. Molecules of air in a room do not all fall and
settle on the ground (due to gravity)because of their high speeds and incessant
collisions. In equilibrtum, there is a very slight increase in density at lower
heights (like in the atmosphere). The effect is small
since the potential energy (mgh) for ordinary
heights is much less than the average kinetic energy 4 nw? of the molecules.
6. <v?> isnot always equal to ( < v >}*.
The average of a squared quanttty is not necessarily the square of the average.
Can you find examples for this statement.
EXERCISES
13.1 Estimate the fraction of molecular volume to
the actual volume occupied by oxygen gas at STP. Take the diameter of an oxygen
molecule to be 3 A.
13.2 Molar volume is the volume occupied by 1 mol of
any (ideal) gas at standard
temperature and pressure (STP : 1 atmogpheric
pressure, 0 °C). Show that it is 22.4 litres.
13.3 Figure 13.8 shows plot of PV/T versus P for
1.00x10° kg of oxygen gas at two different temperatures.
(a) What does the dotted plot signify?
(b) Which is true: T, > T, or T, < T,?
(c) What is the value of PV/T where the curves meet
on the y-axis?
(d) If we obtained similar plota for 1.00x10° kg of
hydrogen, would we get the same value of PV/T at the point where the curves
meet on the y-axis? If not, what mass of hydrogen yielda the same value of PV/T
(for low preasurehigh temperature region of the plot) ? (Molecular masa of H, =
2.02 u, of 0, = 32.0 u,R=8.31J mol K+)
13.4 = An oxygen cylinder of volume 30 litres has an
initial gauge pressure of 15 atm and @ temperature of 27 °C. After some oxygen
1s withdrawn from the cylinder, the gauge preasure drops to 11 atm and its
temperature drops to 17 °C. Estimate the mase of oxygen taken out of the
cylinder (R = 8.31 J mol! K", molecular mass of O, = 32 u).
13.5 An air bubble of volume 1.0 cm? rises from the
bottom of a lake 40 m deep at a temperature of 12 °C. To what volume does it
grow when it reaches the surface,which is at a temperature of 36 °C ?
13.6 Latimate the total number of air molecules
(inclusive of oxygen, nitrogen, water vapour and other constituents) in a room
of capacity 25.0 m* at a temperature of 27 °C and 1 atm pressure.
13.7 Eetimate the average thermal energy of a helium
atom at () room temperature
(27 °C), Gi) the temperature on the surface of the
Sun (6000 K), {iti} the temperature of 10 million kelvin (the typical core
temperature in the case of a star).
13.6 Three vessels of equal capacity have gases at
the same temperature and pressure.The first veasel contains neon (monatomic,
the second contains chlorine (diatomic),and the third containe uranium
hexafluoride (polyatomic). Do the vessels contain equal number of respective
molecules ? Is the root mean square speed of molecules the same in the three
cases? If not, in which case is u_, the largeat ?
13.9 At what temperature is the root mean square
speed of an atom in an argon gas cylinder equal to the nme speed of a helium
gas atom at -— 20 °C ? (atomic masa of Ar = 39.9 u, of He = 4.0 wu).
13.10 Eatimate the mean free path and colliaion
frequency of a nitrogen molecule in a cylinder containing nitrogen at 2.0 atm
and temperature 17 °C. Take the radius of a nitrogen molecule to be roughly 1.0
A. Compare the collision time with the time the
molecule moves freely between two succesaive
collisions (Molecular mass of N, = 28.0 w).
Additional Exercises
13.11 A metre long narrow bore held horizontally
(and closed at one end) contains a 76 cm long mercury thread, which traps a 16
cm column of air. What happens if the tube is held vertically with the open end
at the bottam ?
13.12 From a certain apparatus, the diffusion rate
of hydrogen has an average value of 28.7 cm' s"'. The diffusion of another
gas under the same conditions is measured to have an average rate of 7.2 cm®
s', Identify the gas.
[Hint : Use Graham's law of diffusion: R,/R, =(M,
/M, )'?, where R,, R, are diffusion rates of gases 1 and 2, and M, and M, their
respective molecular masses, The law is a simple consequence of kinetic theory.
13.18 A gas in equilibrium has uniform density and
pressure throughout ita volume. This is strictly true only if there are no
external influences. A gas column under gravity,for example, does not have
uniform density {and preasure). As you might expect, its density decreases with
height. The precise dependence is given by the so-called law
of atmospheres a = 1, exp [ -mg (fy - h)/ kT) where
n,, n, refer to number denaity at heights h, and h, respectively. Use this
relation to derive the equation for sedimentation equilibrium of a suspension
in a
Hquid column:n, =n, exp [-mgN, & - P) th, -h)/
(p RDI
where pis the density of the suspended particle, and
p that of surrounding medium.IN, is Avogaciro’s number, and R the universal gas
constant.] [Hint : Use Archimedes principle to find the apparent weight of the
suspended particle.
13.14 Given below are denaities of some solids and liquids. Give rough estimates of the size of their atoms :
[iint : Assume the
atoms to be ‘tightly packed’ in a solid or Hquid phase, and use
the known value of Avogadro's number. You should,
however, not take the actual
numbers you obtain for various atomic sizes too
literally. Because of the crudeness oe he Night pacing erproxkmetion, the
results ealy inflate thet winaic sizes are in the range of a few Al.